Figure shows a schematic view of an electrostatic analyzer. It can sort out charged particles by speed and charge to mass ratio. Spacecraft use such analyzers to characterize charged particles in interplanetary space. Two curved metal plates establish an electric field given by $E = E_{0} (\frac{b}{r})$ where $E_{0}$ and b are positive constants with unit of electric field and length. The field points toward the centre of curvature and r is distance from centre. There is no influence of gravity. Proton (change $+ e$ mass 'm') enters along y-axis and exits along x-axis while moving along a circular path.

Mark the correct option.

If $E_{0}$ is made larger then in order to maintain same trajectory initial speed has to be decreased.

If proton enters closer to the inner surface it will require smaller speed to follow circular trajectory.

It does not matter where the protons enter the device it requires same speed to follow circular trajectory.

A deuteron (charge $+ e$ mass 2m)will require greater speed as compared to proton to follow circular trajectory.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

v is independent of r.

Updated on:21/07/2023

Class 12 PHYSICS MASTER PRACTICE PROBLEM

Topper's Solved these Questions

Knowledge Check

Figure shows a schematic view of an electrostatic analyzer. It can sort out charged particles by speed and charge to mass ratio. Spacecraft use such analyzers to characterize charged particles in interplanetary space. Two curved metal plates establish an electric field given by $E = E_{0} (\frac{b}{r})$ where $E_{0}$ and b are positive constants with unit of electric field and length. The field points toward the centre of curvature and r is distance from centre. There is no influence of gravity. Proton (change $+ e$ mass 'm') enters along y-axis and exits along x-axis while moving along a circular path.

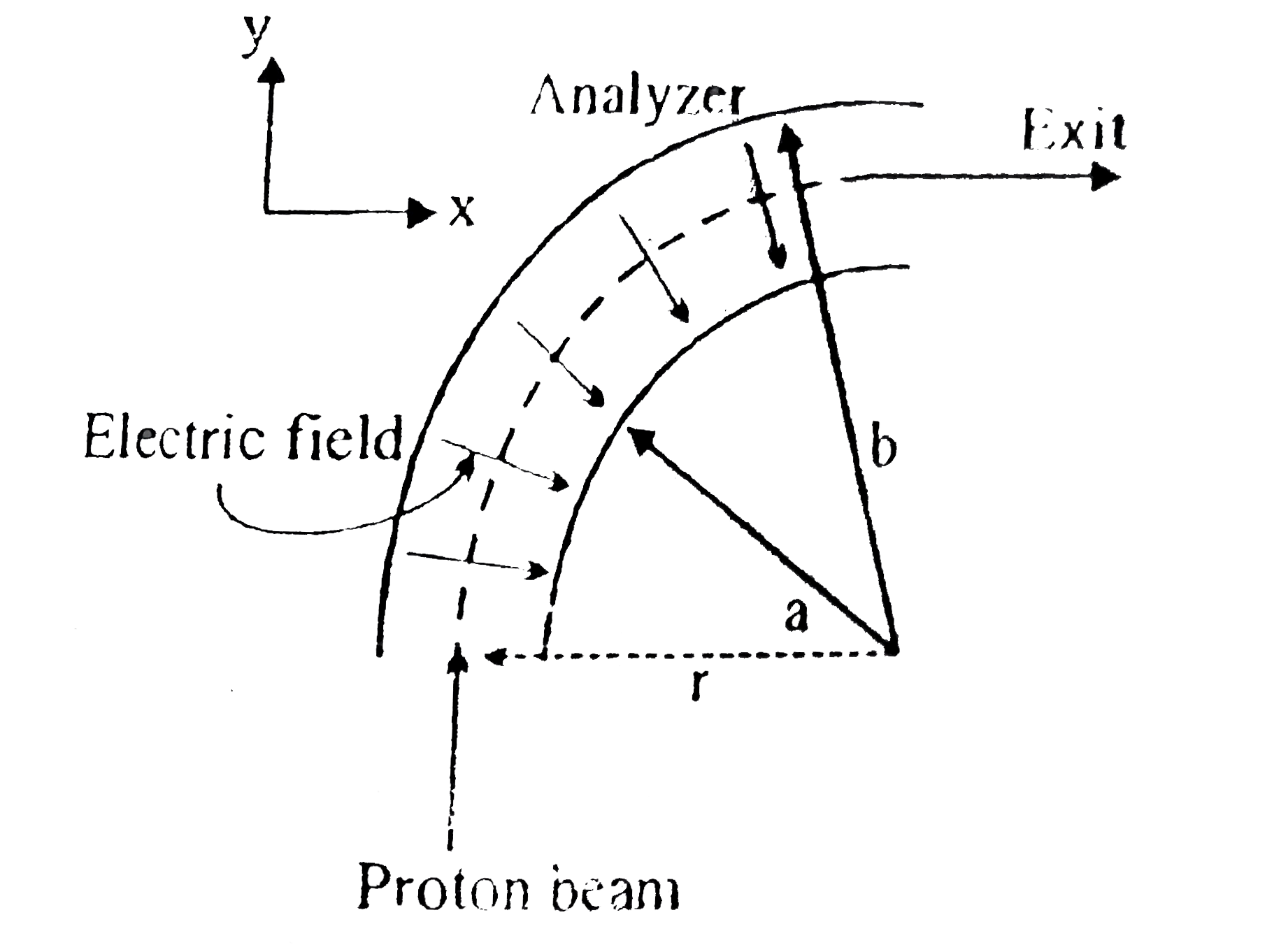
Speed with which protion is to be projected is 'v' and centripetal acceleration of electron is $' a_{c}'$ is given by respectively. Mark the correct statement
A $v = \sqrt{\frac{e E_{0} b}{m}}, a_{c} = \frac{2 e}{m} E_{0} (\frac{b}{r})$
B $v = \sqrt{\frac{2 e E_{0} b}{m}}, a_{c} = \frac{e}{2 m} E_{0} (\frac{b}{r})$
C $v = \sqrt{\frac{e E_{0} b}{2 m}}, a_{c} = \frac{2 e}{m} E_{0} (\frac{b}{r})$
D $v = \sqrt{\frac{e E_{0} b}{m}}, a_{c} = \frac{e}{m} E_{0} (\frac{b}{r})$
A charge particle is moving along positive y-axis in uniform electric and magnetic fields
$E = E_{0} ˆ k$
and $B = B_{0} ˆ i$
Here $E_{0}$ and $B_{0}$ are positive constants.choose the correct options.
Aparticle may be deflected towards positive x-axis
Bparticle may be deflected towards negative z-axis
Cparticle may pass undeflected
Dkinetic energy of particle may remain constant
How does the electric field (E) between the plates of a charged cylindrical capacitor vary with the distance r from the axis of the cylinder ?
A $E \propto \frac{1}{r^{2}}$
B $E \propto \frac{1}{r}$
C $E \propto r^{2}$
D $E \propto r$