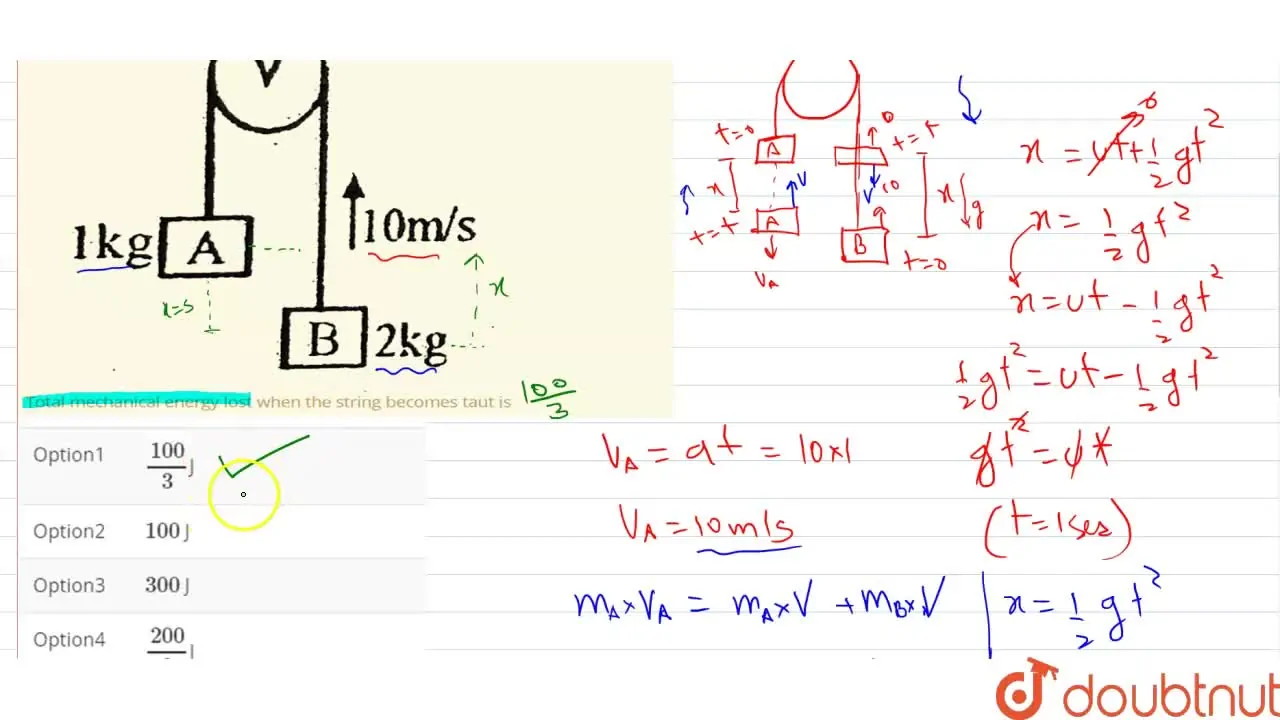
Two block of A and B of mass 1kg and 2kg are hung from light pulley. Initially the block B is held stationary. At block B is given velocity 10m//s in upward direction. String and pulley are light and there is no friction anywhere.

Total mechanical energy lost when the string becomes taut is
J
J
J
J
The correct Answer is:A
String will be taught when distance travelled by the two blocks become equal i.e.
Before t=1 sec acceleration of both block will be equation t 'g' in downward direction
After =1 sec, there will jerk in string and velocity of A and B will be given by
(u_(A)=velocity of A at t=1 sec)
after string becomes thought)
:. velocity of 'B' when 'A' has ascended by 5m is given by
(:. After t=1sec, a=
Related Playlists
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning75videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Multiple objective131videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Additional topic70videos
MASTER PRACTICE PROBLEM
- BOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE10videos
FLUID MECHANICS
- BOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question32videos
SEMICONDUCTORS
Similar Questions
Two block of A and B of mass 1kg and 2kg are hung from light pulley. Initially the block B is held stationary. At t=0 block B is given velocity 10m//s in upward direction. String and pulley are light and there is no friction anywhere.

Time till which acceleration (magnitude and direction both) of the two block ramains sameView SolutionTwo block of A and B of mass 1kg and 2kg are hung from light pulley. Initially the block B is held stationary. At t=0 block B is given velocity 10m//s in upward direction. String and pulley are light and there is no friction anywhere.

Velocity of block 'B' as block 'A' has acended by distance 5m from its original positionView SolutionTwo blocks A and B , each of mass 10kg , are connected by a light string passing over a smooth pulley as shown in the figure. Block A will remain at rest if the friction on it is
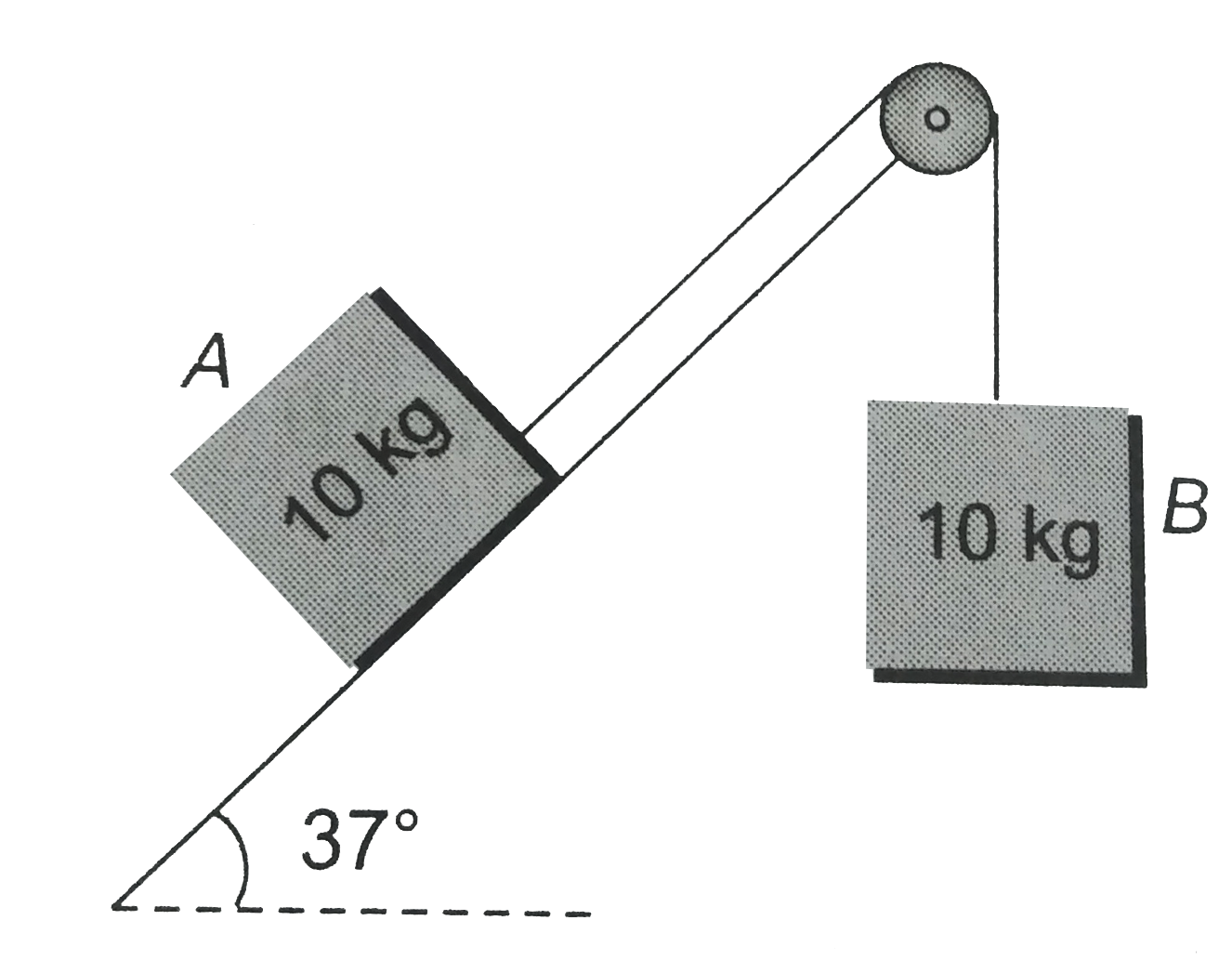 View Solution
View SolutionIn the arrangement shown, find the acceleration of each block, tension in the string and reaction in the pulley. The string and pulley are light (massless) (m1>m2). Neglect the friction in pulley.
 View Solution
View SolutionPQR is a wedge fixed to the floor. Two blocks A & B of equal mass m=1kg is placed on either side of wedge connected through a string passing over the pulley P. Initially PB=PA. Another block C of mass m=1kg moving with a velocity 10m/s collides inelasically with A. All the surfaces are smooth and pulley & string are massless.
(a) What should be the minimum length of the string AB so that block A does not hit the pulley ?
(b) What is the loss in meechanical energy from the instant C hits A to the instant when B starts moving upward ? (g=10m/s2)
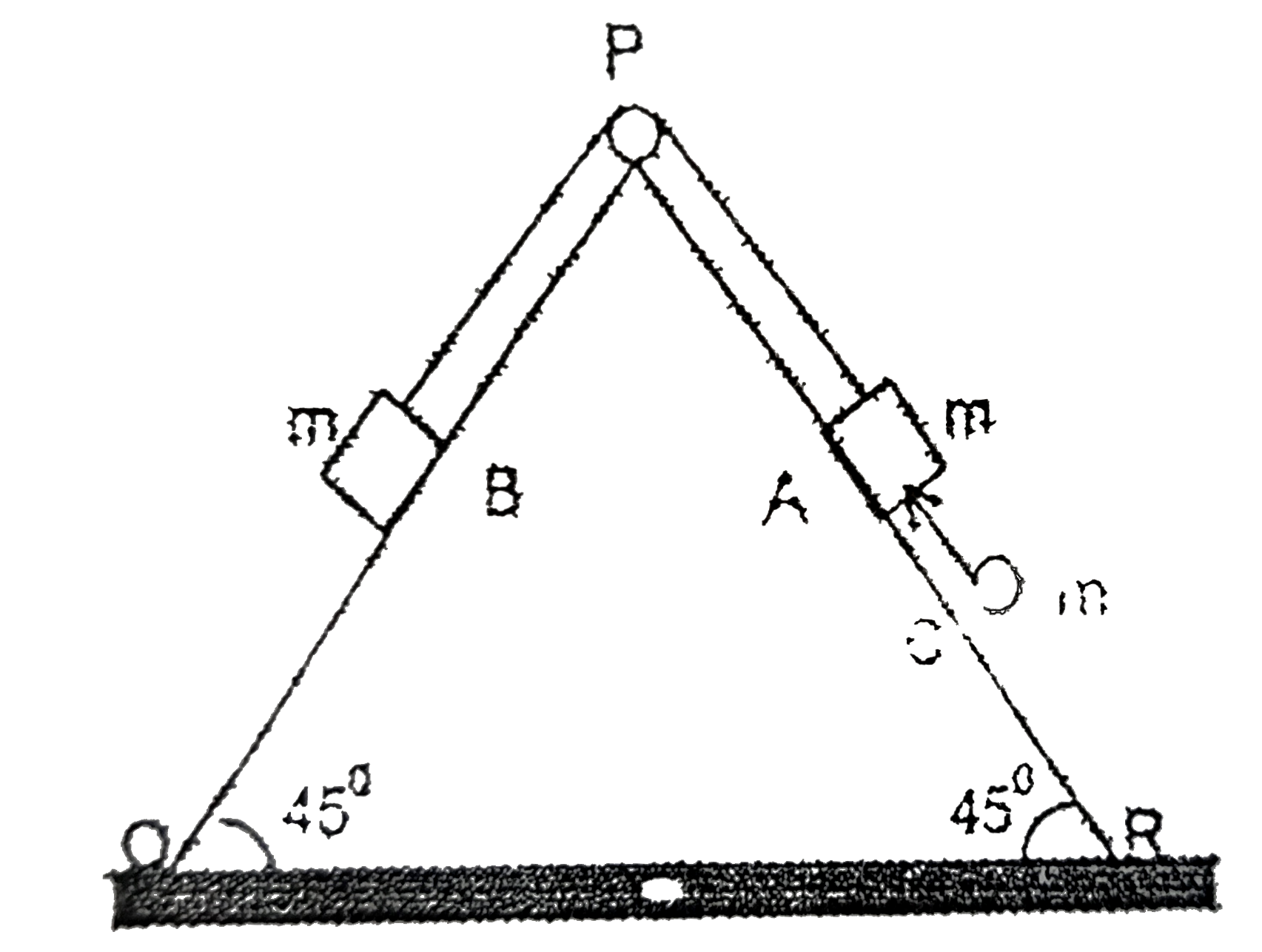 View Solution
View SolutionTwo block A and B of masses 1kg and2kg respectively are connected by a string, passing over a light frictionless pulley B as shown. Another string connect the center of pulley. Both the blocks are resting on a horizontal floor and the pulley is help such that string remains just taut. At moment t=0, a force F=20t starts acting on the pully along vertically upwards direction as shown in figure.Calculate

(a) velicity of A when B loses contact with the floor.
(b) height raised by the pulley upto that instant.
(Take =g=10m/s2)View SolutionString going over a pulley connects two blocks of masses m, and my. String and pulley are light. Calculate acceleration of centre of mass of blocks system.
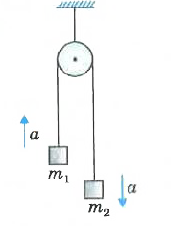 View Solution
View SolutionA light inelastic thread passes over a small frictionless pulley. Two blocks of masses m = 1 kg and M = 3 kg, respectively, are attached with the thread and heavy block rests on a surface. A particle P of mass 1 kg moving upward with a velocity of 10ms−1 collides with the lighter block and sticks to it. The speed of the bigger block just after the string is taut will be [g=10ms−2]
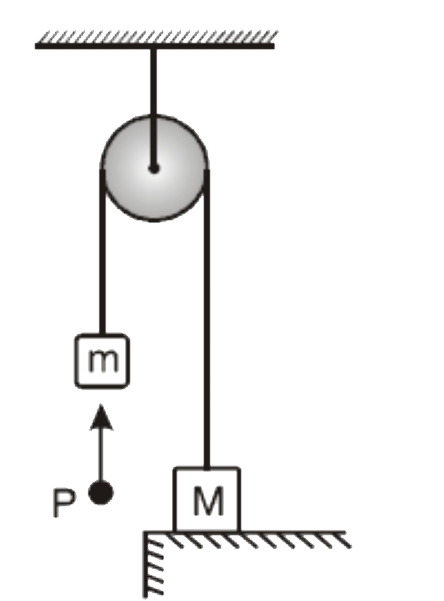 View Solution
View SolutionTwo blocks A and B having masses m1=1kgm2=4kg are arranged as shown in the figure The pulleys P and Q are light and frictionless. All the blocks are resting on a horizontal floor and the pulleys are held such that strings remains just taut. At moment t=0 a force F=30 t (N) starts acting on the pulley P along vertically upward direction as shown in the figure The time when the blocks A and B loose contact with ground is 4/x sec then x is:
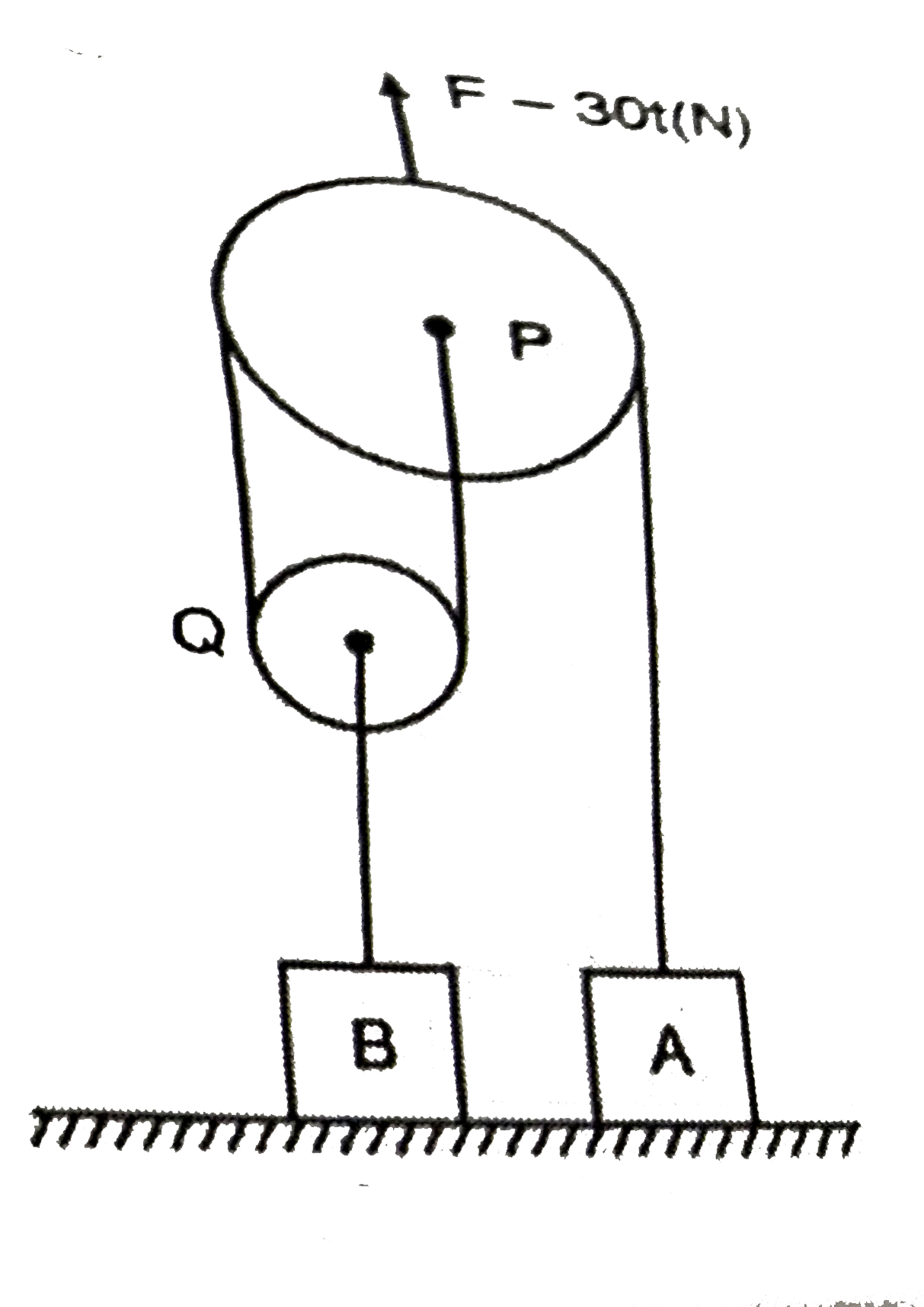 View Solution
View Solution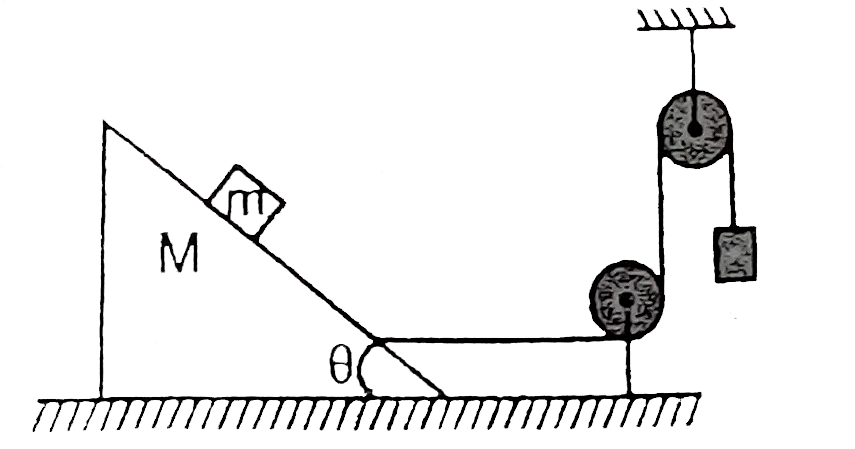
Find the mass (in kg) of the hanging block which will prevent the smaller block from slipping over the triangular block. All the surfaces are frictionless and the strings and the pulleys are light. Given m=M=1kg,cotθ=2,View Solution