A thin perfectly transparent glass sheet of thickness t and refractive index mu is pasted on one of the two identical slits. If the intensity of light at the centre of the screen is I0 in the absence of glass sheet, the intensity at O as a function of thickness of the glass plate is
I0cos(μtλ)
I0cos2(μtλ)
I0cos2(π(μ−I)tλ)
I0cos(π(μ−I)tλ)
The correct Answer is:C
S1O−S2O=(μ−1)t
Δϕ=2πλ(μ−1)t
I=Imaxcos2(Δϕ/2)
∴I=I0cos2(π(μ−1)tλ)
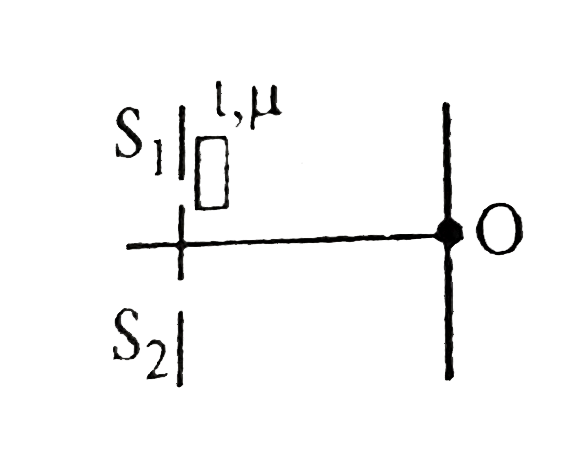
Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Comphrehension
Explore 134 Videos
- MASTER PRACTICE PROBLEMBook:BANSALChapter:MASTER PRACTICE PROBLEMExercise:Reasoning
Explore 75 Videos
- FLUID MECHANICSBook:BANSALChapter:FLUID MECHANICSExercise:PYQS AIEEE
Explore 10 Videos
- SEMICONDUCTORSBook:BANSALChapter:SEMICONDUCTORSExercise:CBSE Question
Explore 32 Videos
Similar Questions
A thin glass plate of thickness t and refractive index μ is inserted between screen and one of the slits in a young's experiment. If the intensity at the centre of the screen is I, what was the intensity at the same point prior to the introduction of the sheet.
View SolutionA thin glass plate of thickness t and refractive index μ is between screen &one of the slits in young's experiment.if the intensity at the center of the screen is I ,was the intenstity at the same point prior to the introduction of the sheet.
(b) One slit of a young's experiment is covered by a glass plate (μ1=1.4) and the other by another glass plate (μ2=1.7) of the same thickness. The point of central maxima of the screen,before the plates were introduced is now occupied by the third bright fringe.Find the thicknes of the thickness of the plates.the wavelength of light used in 4000Å.
View Solution
Knowledge Check
When a thin transparent plate of thickness t and refractive index μ is placed in the path of one the two interfering waves of light, then the path difference changes by
A(μ+1)tB(μ−1)tC(μ+1)tD(μ−1)tLight travels through a glass plate of thickness t and refractive index μ. If c is the speed of light in vacuum, the time taken by light to travel this thickness of glass is
AμtcBtcμC1μtDμtcLight travels through a glass plate of thickness t and refractive index μ. If c is the velocity of light in vaccum, the time taken by the light to travel this thickness of glass is
At(μc)BtμcCμtcDtcμ
A monochromatic light of λ=500nm is incident on two identical slits separated by a distance of 5×10−4m. The interference pattern is seen on a screen placed at a distance of 1m from the plane of slits. A thin glass plate of thickness 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the centre of the screen if the intensity is I0 in the absence of the plate. Also find the lateral shift of the central maxima and number of fringes crossed through centre.
View SolutionMonochromatic light of lamba=5000Å is incident on two slits separately by a distance of 5×10−4m. The interference patteren is seen on a screen placed at a distance of 1m form the slits. A thing glass plate of thicknes 1.5×10−6m and refractive index μ=1.5 is placed between one of the slits and the screen. Find the intensity at the centre of the screen if the density there is I0 in the absence of the plate. Also find the lateral shift of the central maximum. '
View SolutionIn Young’s double slit experiment a monochromatic light of wavelengthλ from a distant point source is inci- dent upon the two identical slits.The interference pattern is viewed on a distant screen. Intensity at a point P is equal to the intensity due to individual slits (equal toI0). A thin piece of glass of thickness t and refractive index μ is placed in front of the slit which is at larger distance from point P, perpendicular to the light path. Assume no absorption of light energy by the glass.
(a) Write intensity at point P as a function of t.
(b) Write all values of t for which the intensity at P is minimum.
View SolutionLight travel through a glass place of thickness t and refractive index 'n'. If c is the velocity of light in vacuum, then the time taken by light to travel the thickness of the plate will be
View SolutionIf a thin mica sheet of thickness 't' and refractive index μ is placed in the path of one of the waves producing interference , then the whole interference pattern shifts towards the side of the sheet by a distance
View Solution