Consider a very long stick of length 2R which extends from just above the surface of the earth to a radius 3R if initial conditions have been set up so that this stick moves in a circular orbit while always pointing radially what is the period of this orbit a represents acceleration due to gravity on surface of earth

A

$6 π \sqrt{\frac{R}{2 g}}$

B

$2 π \sqrt{6} \sqrt{\frac{R}{g}}$

C

$π \sqrt{6} \sqrt{\frac{R}{g}}$

D

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

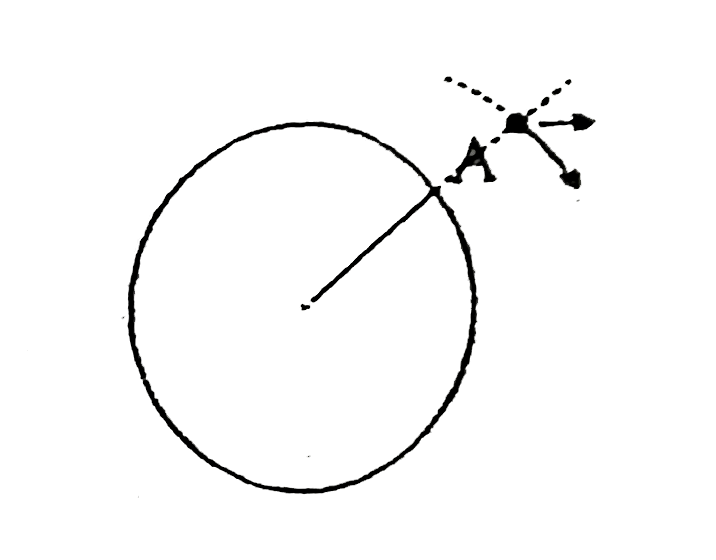
force on this element
$d f = \frac{G M (λ d x)}{x^{2}}$
total force on the stick
$f \int 0 = G m λ 3 R \int R \frac{d x}{x^{2}}$
$f = G m λ {[\frac{- 1}{x}]}_{R}^{3 R} = G m λ [\frac{1}{R} - \frac{1}{3 R}] = G M λ [\frac{2}{3 R}]$
CM of stick is rotating in a circle of radius 2R
$G M [\frac{2 λ}{3 R}] = λ (2 R) (2 R) ω^{2}$
$ω^{2} = \frac{G M}{6 R^{3}}$
$T = 2 π \sqrt{6} \sqrt{\frac{R^{3}}{g R^{2}}} = 2 π \sqrt{6} \frac{\sqrt{R}}{g}$

Updated on:21/07/2023

|

Class 12 PHYSICS MASTER PRACTICE PROBLEM

Topper's Solved these Questions

Similar Questions

Knowledge Check

The ratio of acceleration due to gravity at a height 3 R above earth's surface to the acceleration due to gravity on the surface of earth is
(R = radius of earth)
A $1 / 9$
B $1 / 16$
C $1 / 4$
D $1 / 3$
A small body of mass m falls to the earth from infintie distance away. What will be its velocity or reaching the earth? (Radius of the earth = R, acceleration due to gravity on the surface of the earth is g) :-
A $g R$
B2gR
C $\sqrt{g R}$
D $\sqrt{2 g R}$
A body which is initially at rest at a height R above the surface of the earth of radius R, falls freely towards the earth. Find out its velocity on reaching the surface of earth. Take $g =$ acceleration due to gravity on the surface of the Earth.
A $\sqrt{2 g R}$
B $\sqrt{\frac{3}{2} g R}$
C $\sqrt{g R}$
D $\sqrt{\frac{1}{2} g R}$

BANSAL-MASTER PRACTICE PROBLEM-Additional topic

Home
Ask Doubt
Profile