The young's modulus of material of a thin ring shaped elastic body is Y the mass of ring is m area of cross section is A its's initial radius is R ring is a little elongated then left alone At what time will ring circumference be same as it was initially neglect loss of energy
√πmR8YA
32√πmRYA
2√πmRYA
√πmR2YA
The correct Answer is:A
dF=2Tsin(dthη2)
a=dfdm=2(AYlΔl)(dθ2)(m2π.dθ)
=2πAYΔlml
2πAY(ΔR)mR
a=(AY2πm.R)ΔR
(ω2)ΔR
T=2πω=2π√mRAY(2π)
t=T4=π2√mRAY(2π)
√πmR8YA

Topper's Solved these Questions
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Comphrehension
134 Videos
- MASTER PRACTICE PROBLEMBOOK - BANSALCHAPTER - MASTER PRACTICE PROBLEMEXERCISE - Reasoning
75 Videos
- FLUID MECHANICSBOOK - BANSALCHAPTER - FLUID MECHANICSEXERCISE - PYQS AIEEE
10 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A ring of mass m and radius R is being rotated about its axis with angular velocity ω. If a increases then tension in ring
View SolutionA vertical cylindrical region has a horizontal radial magnetic field inside it. A wire ring is made of a wire of cross section S, density d and resistivity ρ. Radius of the ring is r. The ring is kept horizontal with its centre on the axis of the cylindrical region and released. The field strength at all points on the circumference of the ring is B. At a certain instant velocity of the ring is v downward. Find
(a) Current in the ring and
(b) Acceleration of the ring at the instant.
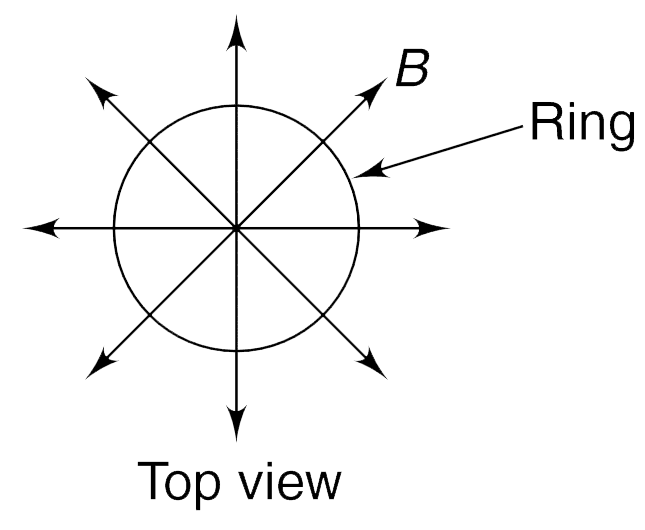
View Solution
Knowledge Check
The total energy of rolling ring of mass m and radius R is
A3/2mv2B1/2mv2Cmv2D5/2mv2A steel ring of radius r and cross section area A is fitted on to a wooden disc of radius R(R>r). If Young's modulus be E, then the force with which the steel ring is expanded is
AAERrBAE(R−rr)CEA(R−rA)DErARA uniform thin ring of radius R and mass m suspended in a vertical plane from a point in its circumference. Its time period of oscillation is
A2π√2RgBπ√2RgCπ2√RgDπ√R2g
_S01_005_Q01.png)
Find out the elongation in block. If mass area of cross-section and young modulus of block are m, A and y respectively.
View SolutionA thin ring and a solid disc of same mass and radius are rolling with the same linear velocity. Then ratio of their kinetic energies is
View SolutionA block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View SolutionYoung's modulus for a wire of length L and area of cross-section A is Y. What will be Young's Modulus for wire of same material, but half its original length and double its area?
View SolutionA metal ring of initial radius r and cross-sectional area A is fitted onto a wooden disc of radius R > r. If Youngs modules of metal is Y then tension in the ring is
View Solution