Which of the following numbers is NOT a solution of the inequality 7−5x≤−3(x−5)?
−5
−4
−2
1
The correct Answer is:A
To solve the inequality 7−5x≤−3(x−5), we will follow these steps:
Step 1: Expand the right side of the inequality
Start by distributing −3 on the right side:
7−5x≤−3x+15
Step 2: Move all terms involving x to one side
Add 3x to both sides to combine x terms:
7−5x+3x≤15
This simplifies to:
7−2x≤15
Step 3: Isolate the x term
Subtract 7 from both sides:
−2x≤15−7
This simplifies to:
−2x≤8
Step 4: Divide by −2
When dividing by a negative number, remember to reverse the inequality sign:
x≥−4
Conclusion
The solution to the inequality is:
x≥−4
Step 5: Identify which option is NOT a solution
Now, we need to check which of the given options does not satisfy x≥−4.
Assuming the options are:
- A) -5
- B) -4
- C) 0
- D) 5
From our solution:
- Option A: −5 (not a solution, since −5<−4)
- Option B: −4 (is a solution, since −4=−4)
- Option C: 0 (is a solution, since 0>−4)
- Option D: 5 (is a solution, since 5>−4)
Thus, the number that is NOT a solution to the inequality is:
Option A: -5
Start by distributing −3 on the right side:
7−5x≤−3x+15
Add 3x to both sides to combine x terms:
7−5x+3x≤15
This simplifies to:
7−2x≤15
Subtract 7 from both sides:
−2x≤15−7
This simplifies to:
−2x≤8
When dividing by a negative number, remember to reverse the inequality sign:
x≥−4
The solution to the inequality is:
x≥−4
Now, we need to check which of the given options does not satisfy x≥−4.
- A) -5
- B) -4
- C) 0
- D) 5
- Option A: −5 (not a solution, since −5<−4)
- Option B: −4 (is a solution, since −4=−4)
- Option C: 0 (is a solution, since 0>−4)
- Option D: 5 (is a solution, since 5>−4)
Option A: -5
Topper's Solved these Questions
Similar Questions
Knowledge Check
Which of the following numbers is NOT a solution of the inequality 3x−5≥4x−3?
A−1B−2C−3D−5Which of the following numbers is not a solution to the inequality 6x−9≥7x−5?
A−8B−5C−4D−2Whenever a>0 , which of the following number line graphs represents the solutions for x to the inequality |x−a|≤3 ?
A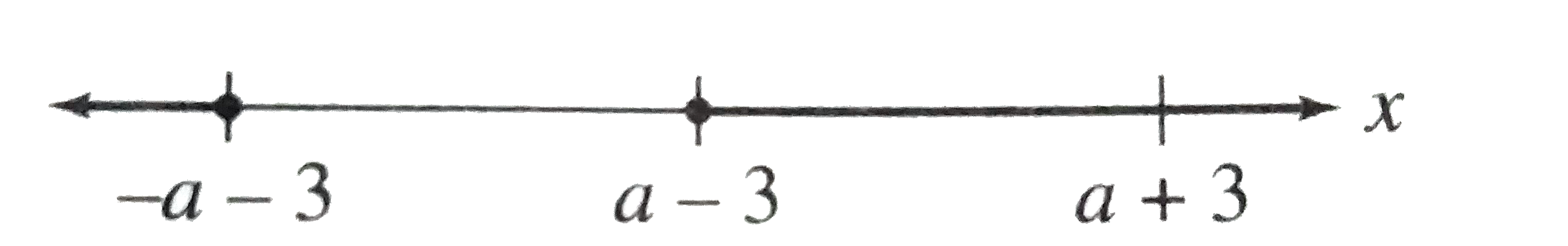 B
B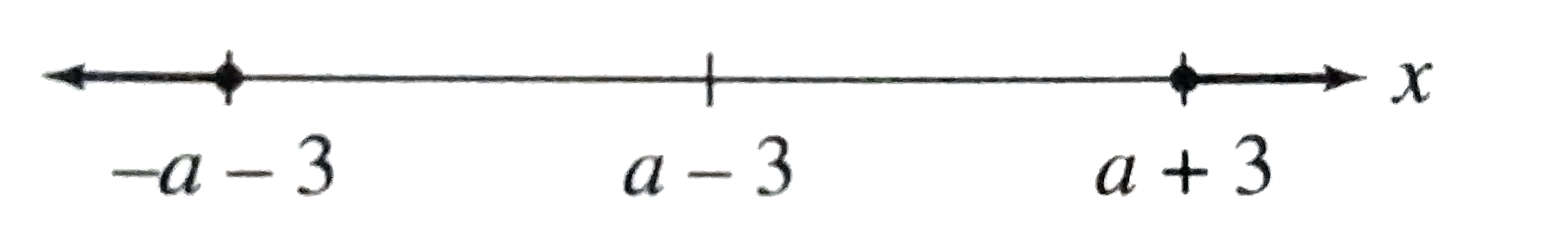 C
C D
D
The solution set of the inequality (x+3)5−(x−1)5≥244 is
View SolutionThe solution set of the inequality log58(2x2−x−38)≥1 is-
View SolutionSolve the inequation: 5x−1>3x+7
View SolutionThe solution set of the inequation 4x+32x−5<6, is
View SolutionWhich of the following are true for the inequation (x−1)(x−2)(x−4)(x−9)(x−12)≤0? (a)Number of natural numbers satisfying the inequation are 8. (b)Number of prime numbers satisfying the inequation are 3. (c)Number of composite numbers satisfying the inequation are 4.
View Solution
