Question

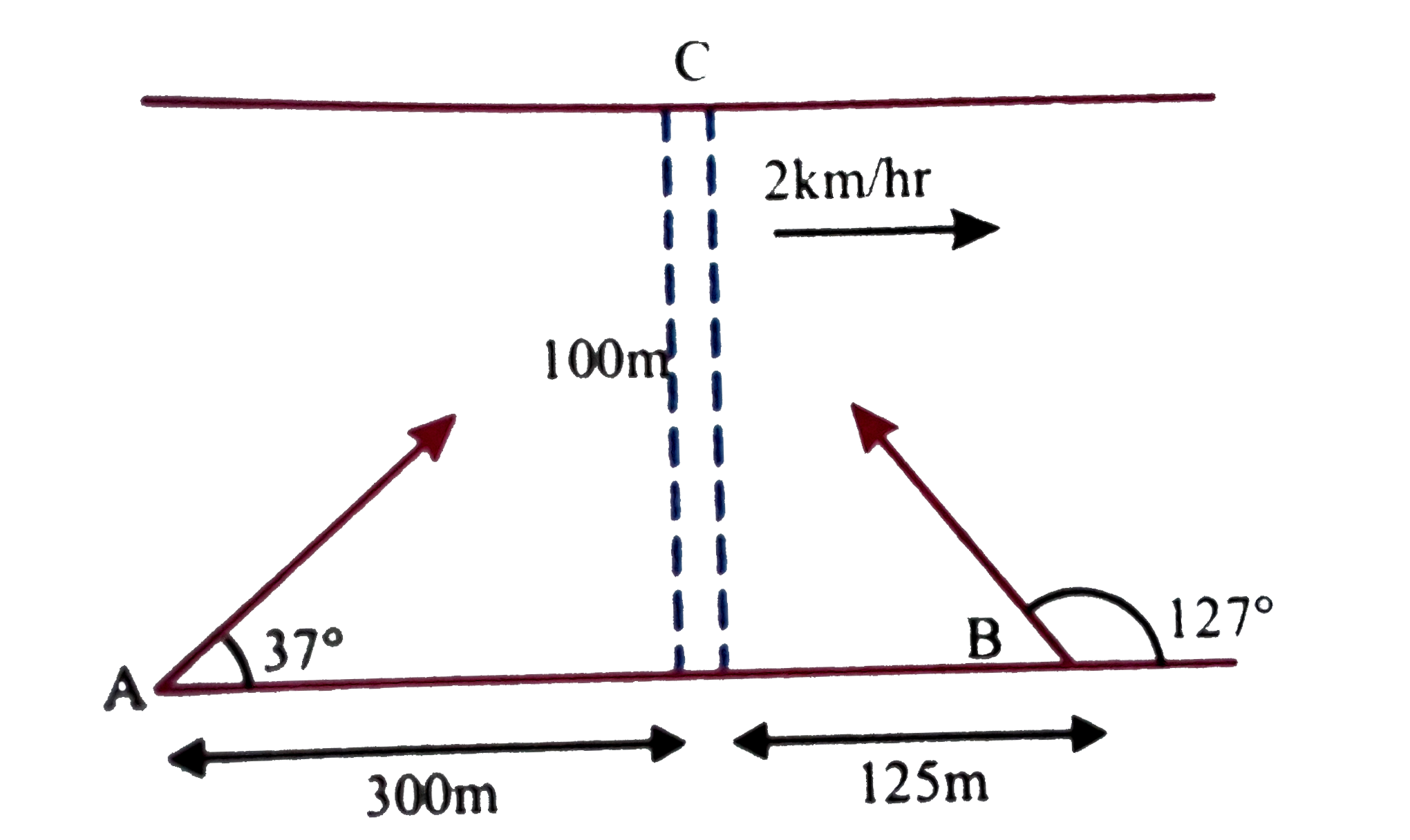
Two swimmers start a race. One who reaches the point ${\displaystyle C}$ first on the other bank wins the race.${\displaystyle A}$ makes his strokes in a direction of ${\displaystyle {37}^0}$ to the river flow with velocity ${\displaystyle 5km/hr}$ relative to water. ${\displaystyle B}$ makes his strokes in a direction ${\displaystyle {127}^0}$ to the river flow with same relative velocity.River is flowing with speed of ${\displaystyle 2km/hr}$ and is ${\displaystyle 100m}$ wide.speeds of ${\displaystyle A}$ and ${\displaystyle B}$ on the ground are ${\displaystyle 8km/hr}$ and ${\displaystyle 6km/hr}$ respectively.

Answer

`B, t_(A)=165s, t_(B)=150 s`

Explanation

`V_(A)=(4+2)hat(i) +3 hat(j),V_(B)=(-3+2)hat(i) +4 hat(j)`

Time to cross the river `t_(A) =(100)/(3) ,t_(B)=(100)/(4)`
Drift `= (100)/(3) xx 6 = 200 m` , Drift `= -1 xx (100)/(4)`
Remaining distance `= 300 - 200 + 25 m`
`(t_("total"))_(A)=(100)/(3)+(100)/(8) ,t_(B)=(100)/(4)+(100)/(6)`
`t_(A)=(800+300)/(24)=(1100)/(24) ,t_(B)=(600+400)/(24)=(1000)/(24)`
`t_(A) = 165 sec t_(B)=150 sec`.