A wire having cross-sectional area S is attatched to wall on one side and a block of mass M on the other side which placed on a horizontal surface having coefficient of friction μ as shown . Material of wire has coefficient of thermal expansion α and Young's modulus Y. At initial temperature there is no stress in the wire. Now the wire is cooled. At what decrease in temperature the block will begin to move.

μMgαYS
2μMgαYS
μMg2αYS
none
The correct Answer is:A
On decreasing, the temperature by (Δθ), the tension developed will be
T=YSα(Δθ)
when about to ship, T=fmax
Ysα(Δθ)=μMg
Δθ=μMgαYS
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A wire of cross sectional ara A at temmperature T is held taut with negligible tension between two ridig supports . If the wire is cooled to a temperature (T−ΔT), what tension is developed in the wire? The coefficient of linear expansion is α and the Young's modulus of the wires is Y
View SolutionYoun'g modulus of the meterial of a wire is numerically equal to stress which will ……… of a wire.
View Solution
Knowledge Check
A metal rof having coefficient of linear expansion α and Young's modulus Y is heated to raise its temperature by Δθ. The stress exerted by the rod is
AYαΔθBYΔθαCYαΔθDαΔθYA metal rod having coefficient of linear expansion (α) and Young's modulus (Y) is heated to raise the temperature by Δθ. The stress exerted by the rod
AYαΔθBYΔθαCYαΔθDαΔθYThe young’s modulus of a wire is numerically equal to the stress which wire
Anot change the length of the wireBdouble the length of the wireCIncrease the length by 50%Dchange the radius of the wire to half
Which one of the following is the Young's modulus (in N/m) for the wire having the stress-strain curve shown in the figure
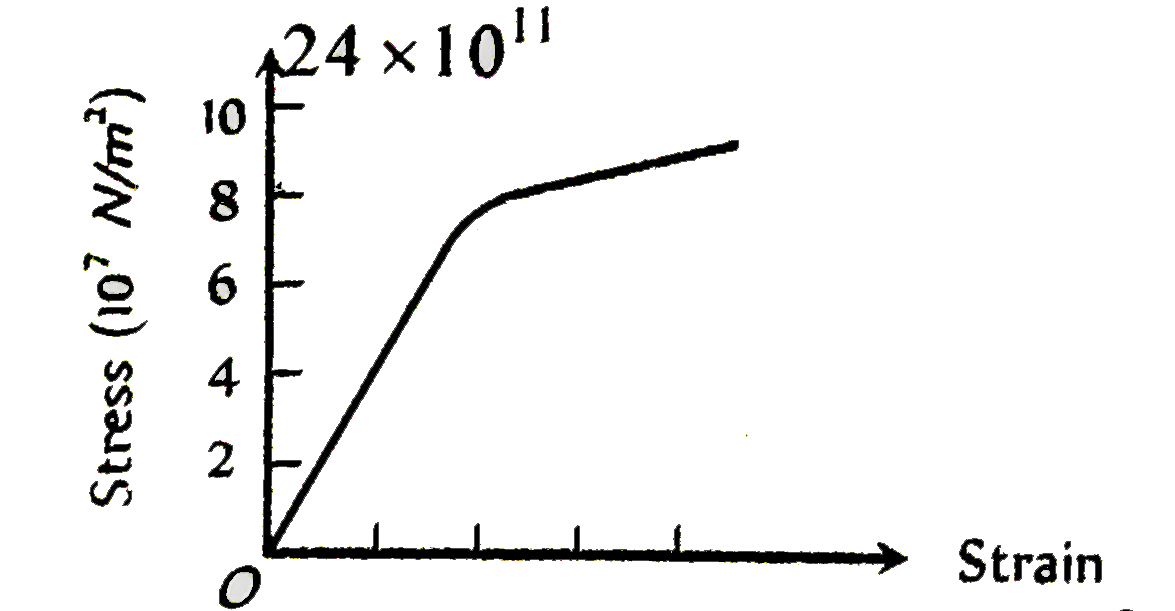
View SolutionIf 'S' is stress and 'Y' is young's modulus of material of a wire, the energy stored in the wire per unit volume is
View SolutionA wire of length L and cross-sectional area A is made of material of Young's modulus Y. The work done in stretching the wire by an amount x is
View SolutionTo determine Young's modulus of the material of a wire,
View SolutionA wire of length L0 is supplied heat to raise its temperature by T. if γ is the coefficient of volume expansion of the wire and Y is Young's modulus of the wire then the energy density stored in the wire is
View Solution