A flexible smooth track is fixed in two alternate arrangments, as shown in figure -1 and 2. the length of the track used is the same in each case, and the height through which it falls from the bench to the floor is the same. A toy car is released at rest and slides down the track. air resistance can be ignored.
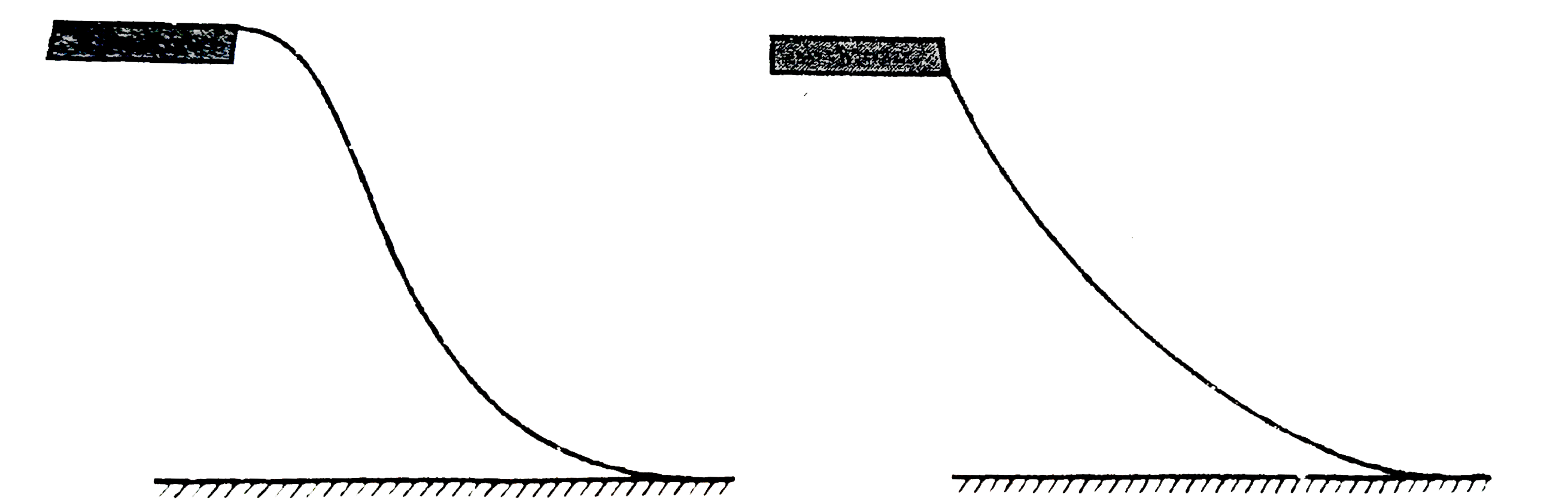
The speed at the bottom as well as time taken in both the traks are the same.
The speed at the bottom as well as time taken in both the tracks are different
The speed at the bottom is different but the time taken in both the tracks are the same.
The speed at the bottom is same but the time taken in both the tracks are different.
The correct Answer is:D
Step by step video, text & image solution for A flexible smooth track is fixed in two alternate arrangments, as shown in figure -1 and 2. the length of the track used is the same in each case, and the height through which it falls from the bench to the floor is the same. A toy car is released at rest and slides down the track. air resistance can be ignored. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
A particle slides along a track with elevated ends and a flat central part as shown in figure. The flat part has a length l=3m. The curved portions of the track are frictionless. For the flat part, the coefficient of kinetic friction is μk=0.2. The particle is released at point A which is at height h=1.5m above the flat part of the track. Where does the particle finally come to rest?

View Solution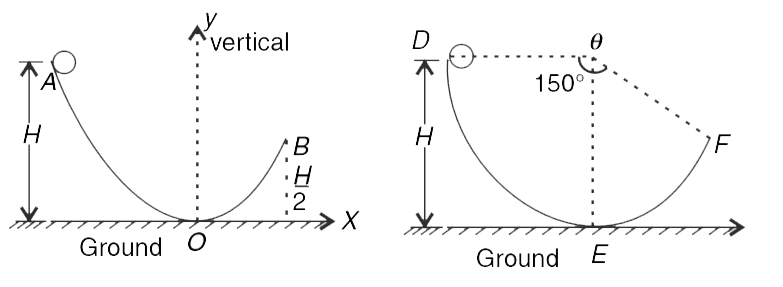
AOB is a frictionless parabolic track in vertical plane. The equation of parabolic track can be expressed as y=32Hx2 for co-ordinate system shown in the figure. The end B of the track lies at y=H2. When a uniform small ring is released on the track at A it was found to attain a maximum height of h1, above the ground after leaving the track at B. There is another track DEF which is in form of an arc of a circle of radius H subtending an angle an angle of 150∘ at the centre. The radius of the track at D is horizontal. The same ring is released on this track at point D and it rolls without sliding. The ring leaves the track at F and attains a maximum height of h2 above the ground. Find the ratio h1h2.
View Solution
Knowledge Check
A flexible track is fixed in two alternative arrangements, as shown in the figure. A toy car is released at rest and slides down the track air resistance can be ignored. The length of the track used is the same in each case and the height through which it falls from the bench to the floor is the same.

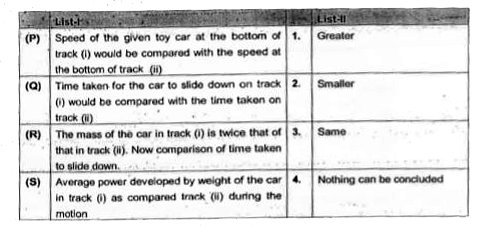
codes:APQRS1423BPQRS2312CPQRS3112DPQRS4132A small block is projected into each of the four tracks as shown below. Each of the tracks rises to the same height. The speed with which the block enters the track is the same in all cases. At the highest point of the track, the normal reaction is maximum in
A B
B C
C D
D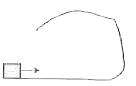
A small block is shot into each of the four tracks as shown below. Each of the tracks rises to the same height. The speed with which the block enters the track is the same in all cases. At the highest point of the track, the normal reaction is maximum in
A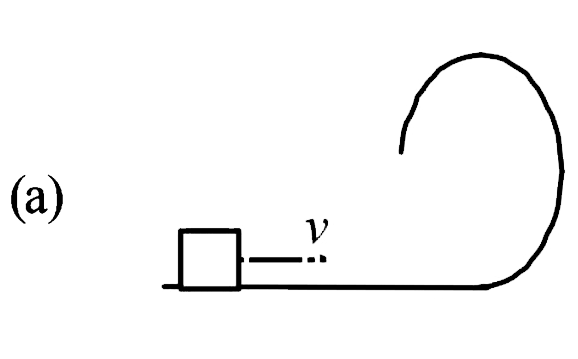 B
B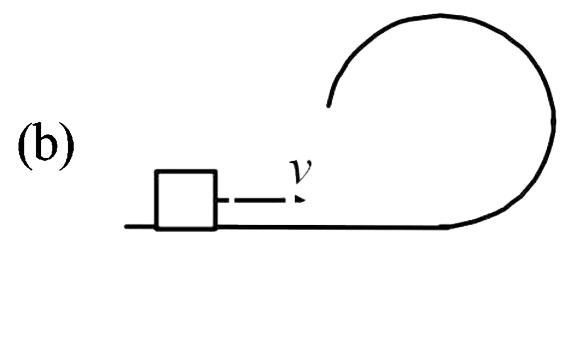 C
C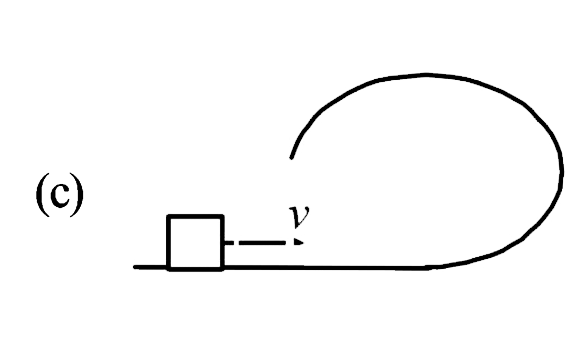 D
D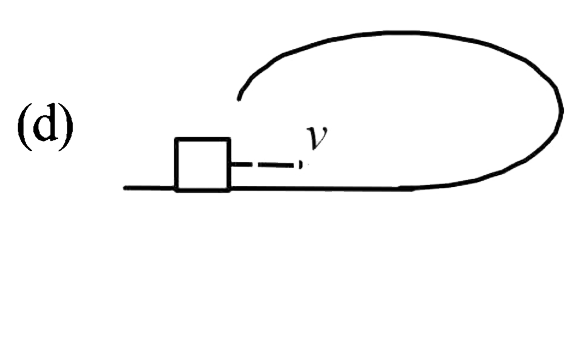
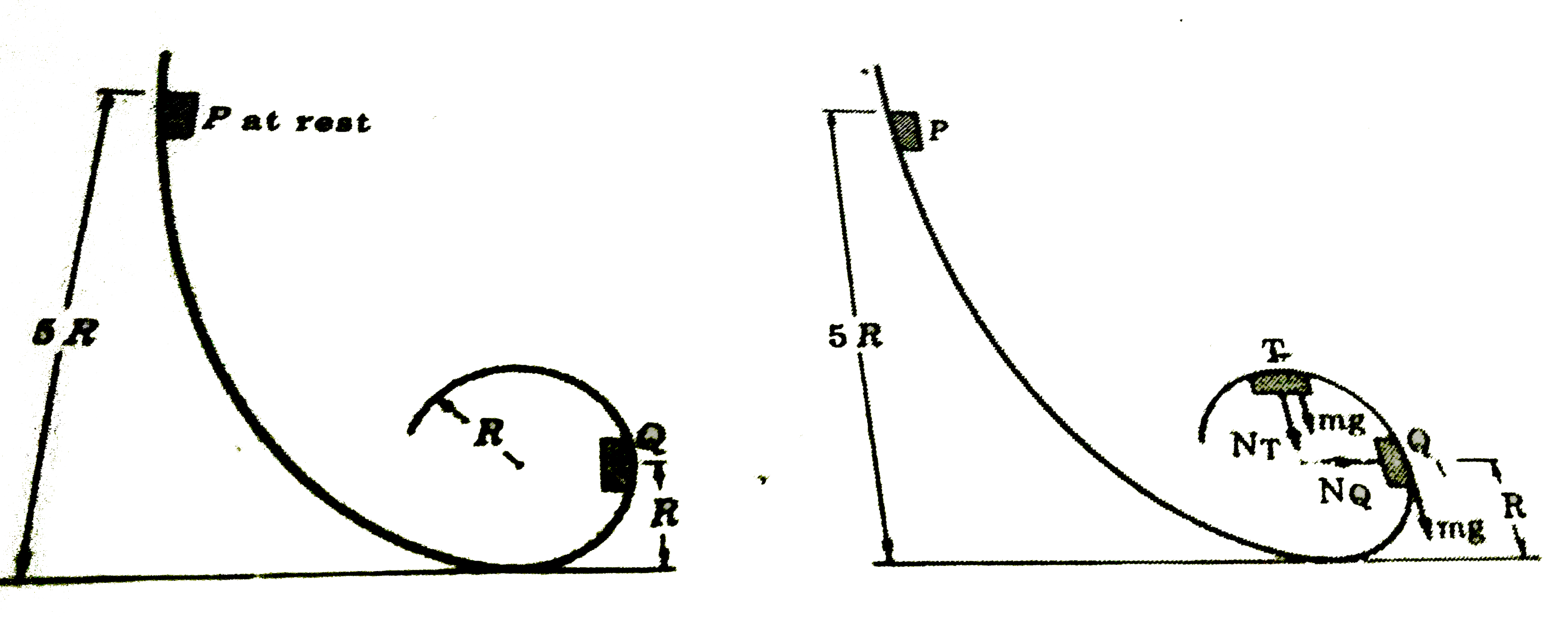
A small block of mass m slides along a smooth track, as shown in fig. (i) If it starts from rest at P, what is the resulting force acting on it at Q ? (ii) at what height above the bottom of the loop should the block be released so that the force it exerts against the track at the top of the loop equals its weight?
View SolutionA vertical frictionless semicircular track of radius 1m fixed on the edge og a movable trolley (figure). Initially, the system is rest and a mass m is kept at the top of the track. The trolly starts moving to the right with a uniform horizontal acceleration a=2g/9 . The mass slides down the track, eventually losing contact with it and dropping to the floor 1.3m below the trolly. This 1.3m is from the point where mass loses contact. (g=10m/s2)
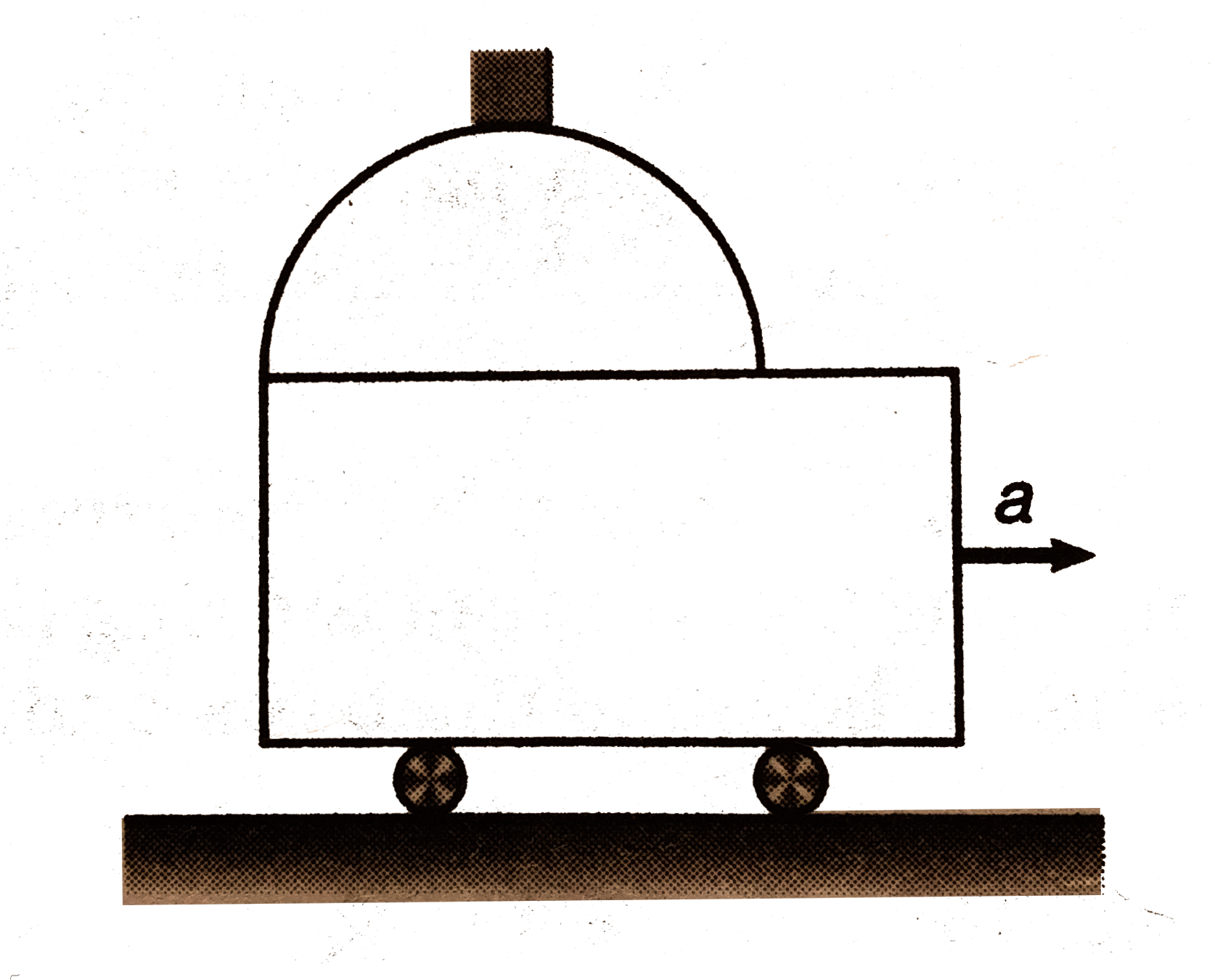
(a) Calculate the angle θ at which it loses contact with the trolly and
(b) the time taken by the mass to drop on the floor, after losing contact.
View SolutionA particle slides along a track with elevated ends and a flat central part as shown in figure. The flat part has a length l=3m. The curved portions of the track are frictionless. For the flat part, the coefficient of kinetic friction is μk=0.2. The particle is released at point A which is at height h=1.5m above the flat part of the track. Where does the particle finally come to rest?

View SolutionA small block is shot into each of the four tracks as shown below. Each of the tracks rises to the same height. The speed with which the block enters the tracks is the same in all cases. At the highest point of the track, the normal reaction is maximum in :
View SolutionAn object of mass m is released from rest at a height h above the surface of a table. The object slides along the inside of the loop. The loop track consisting of a ramp and a circular loop of radius R shown in the figure. Assume that the track is frictionless. When the object is at the top of the circular track it pushes against the track with a force equal to three times its weight. What height was the object dropped from?

View Solution
