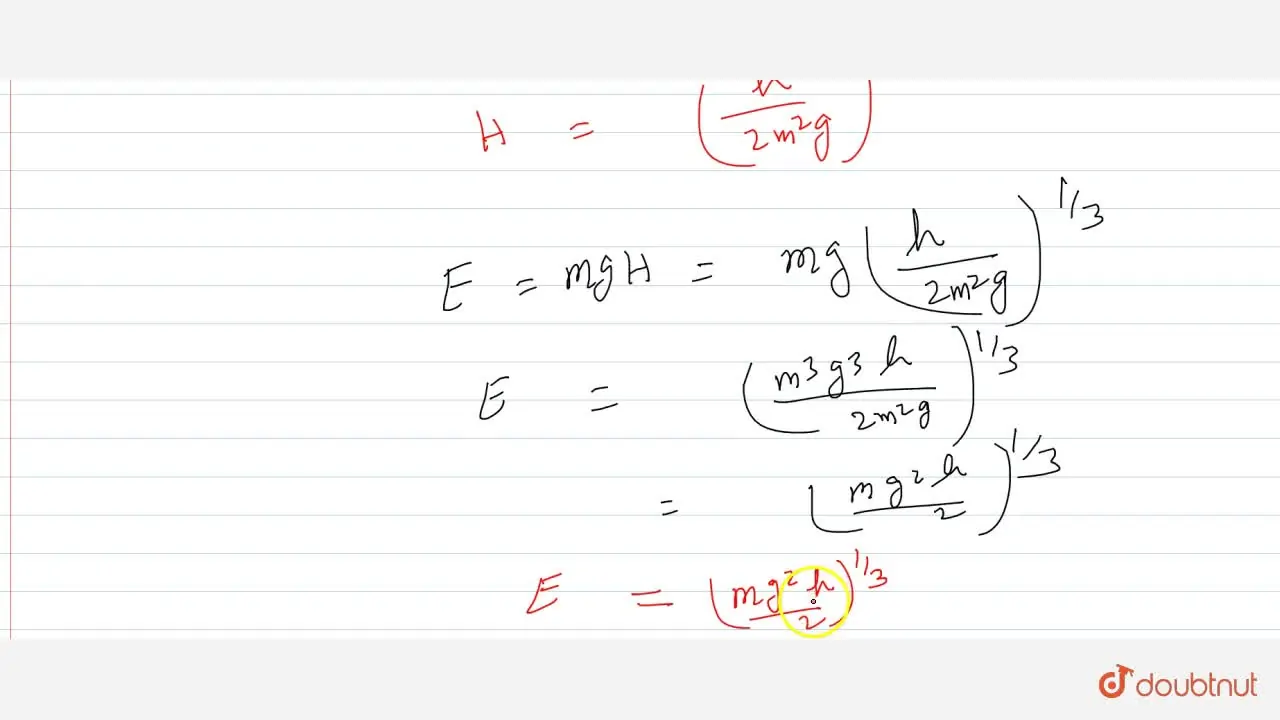A particle of mass m is allowed to fall freely under gravity on an elastic horizontal surface. The quantum effect become important if the smallest de-Broglie wavelength of the particle is of the same order as the height from which it was dropped. Write the mechanical energy of the particle if quantum effects become important
The correct Answer is:E=(mg2h22)1/3
Step by step video, text & image solution for A particle of mass m is allowed to fall freely under gravity on an elastic horizontal surface. The quantum effect become important if the smallest de-Broglie wavelength of the particle is of the same order as the height from which it was dropped. Write the mechanical energy of the particle if quantum effects become important by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
Write the relationship of de-Broglie wavelength λ associated with a particle of mass m in terms of its kinetic energy.
View SolutionA particle of mass m is released from rest at point A in the figure falling freely under gravity parallel to the vertical Y-axis. The magnitude of angular momentum of particle about point O when it reaches B is (where OA=b and AB=h)
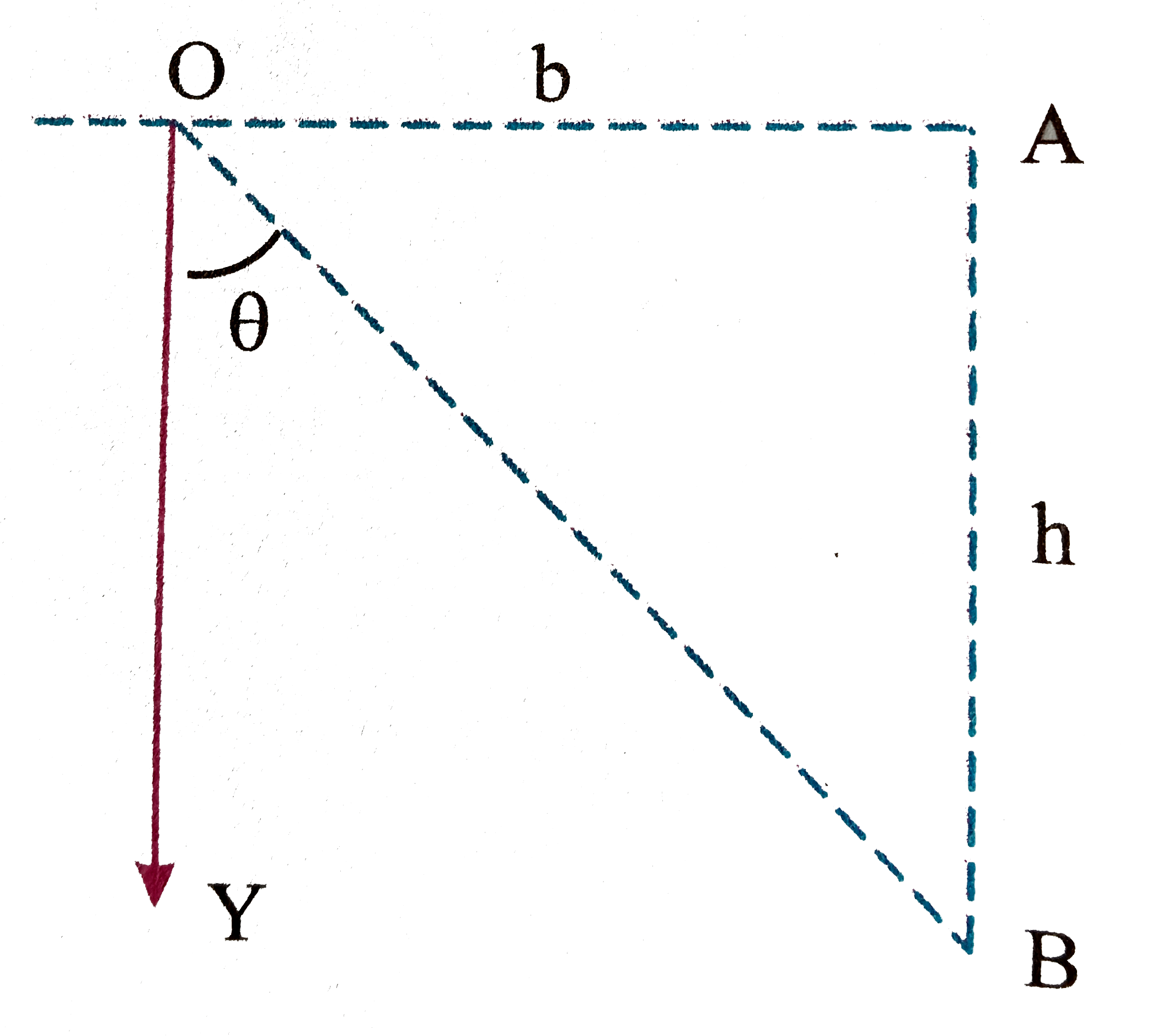 View Solution
View SolutionA particle A of mass m and initial velocity v collides with a particle of mass m/2 which is at rest. The collision is head on, and elastic. The ratio of the de-broglie wavelength λAandλB after the collision is
View SolutionA particle of mass m is flying horizontally at velocity u. It strikes a smooth inclined surface and its velocity becomes vertical.
(a) Find the loss in kinetic energy of the particle due to impact if the inclination of the incline is 60∘ to the horizontal.
(b) Can the particle go vertically up after collision if inclination of the incline is 30∘ ?
 View Solution
View SolutionA particle of mass m is projected upwards from the surface of the earth with a velocity equal to half the escape velocity. (R is radius of earth and M is mass of earth)Calculate the potential energy of the particle at its maximum. Write the kinetic energy of the particle when it was at half the maximum height.
View SolutionA particle of mass 'm' is raised to a height h=R from the surface of earth. Find increase in potential energy. R= radius of earth. g= acceleration due to gravity on the surface of earth.
View Solution(a) A particle is moving three times as fast as an electron. The ratio of the de-Broglie wavelength of the particle to that of the electron is 1.813×10−4. Calculate the particle's mass and identify the particle.
(b) An electron and proton have the same kinetic energy. which of the two will have larger de Broglie wavelength ? Give reason.View SolutionA nonconducting disk of radius a and uniform positive surface charge density σ is placed on the ground, with its axis vertical. A particle of mass m and positive charge q is dropped, along the axis of the disk, from a height H with zero initial velocity. The particle has q/m=4ε0g/σ.
(i) Find the value of H if the particle just reaches the disk.
(ii) Sketch the potential energy of the particle as a function of its height and find its equilibrium position.View SolutionA rod AB of mass 3m and length 4a is falling freely in a horizontal position and c is a point distance a from A. When the speed of the rod is u, the point c collides with a particle of mass m which is moving vertically upwards with speed u. if the impact between the particle and the rod is perfectely elastic find
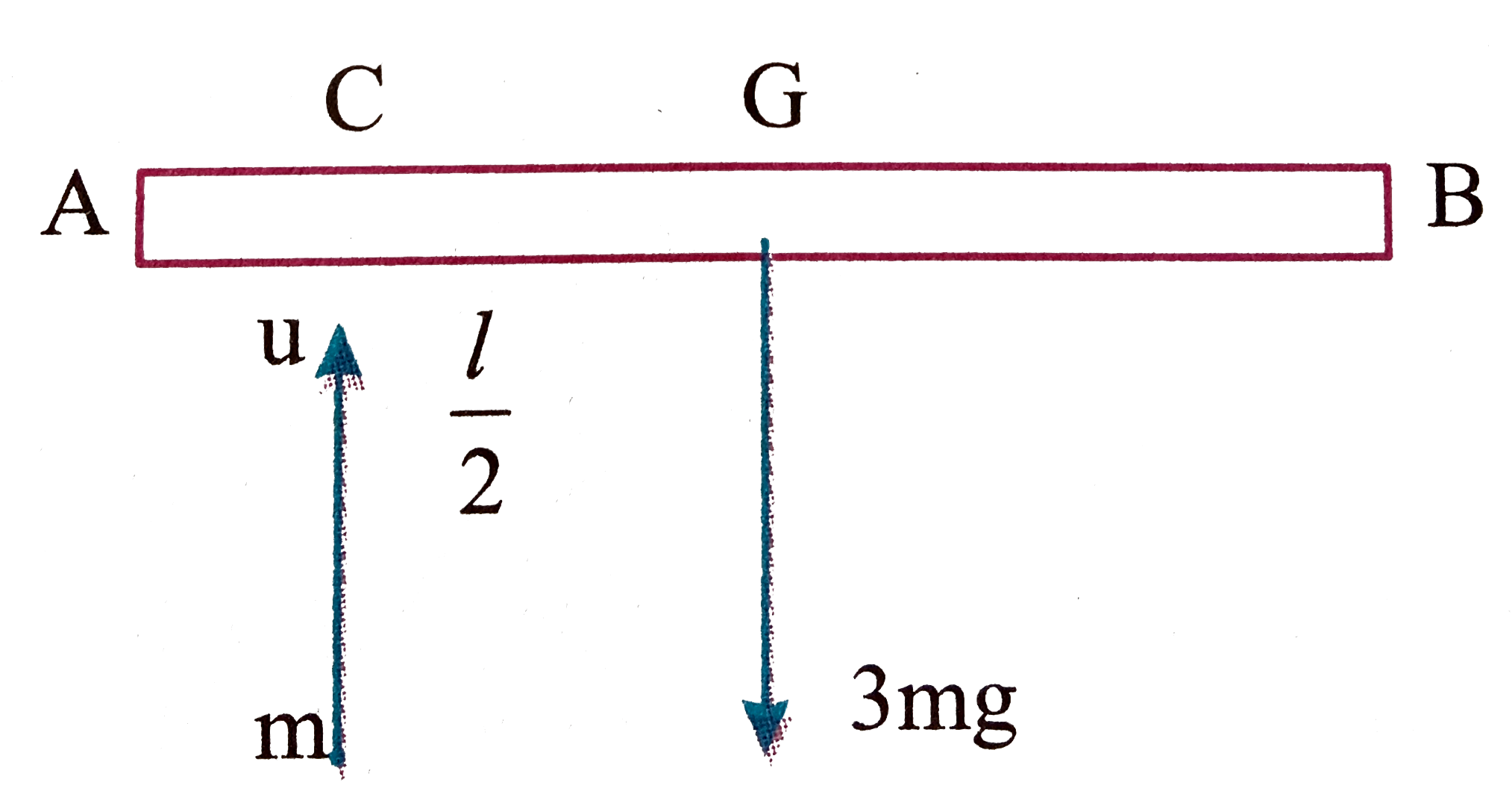
The angular velocity of the rod immediately after the impactView SolutionA particle A of mass 2m is held on a smooth horizontal table and is attached to one end of an inelastic string which runs over a smooth light pulley at the edge of the table. At the other end of the string there hangs another particle B of mass m, the distance from A to the pulley is l. The particle A is then projected towards the pulley with velocity u.

(a) Find the time before the string becomes taut, and shown that after the string becomes taut, the initial velocity of A and B is 4u/3.
(b) Find the common velocity when A reaches the pulley (assume that B has not yet reached the ground).View Solution