In young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S1 and S2 which act as two coherent sources. The sources S oscillates about its own position according to the equation y=0.5sinπt, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is
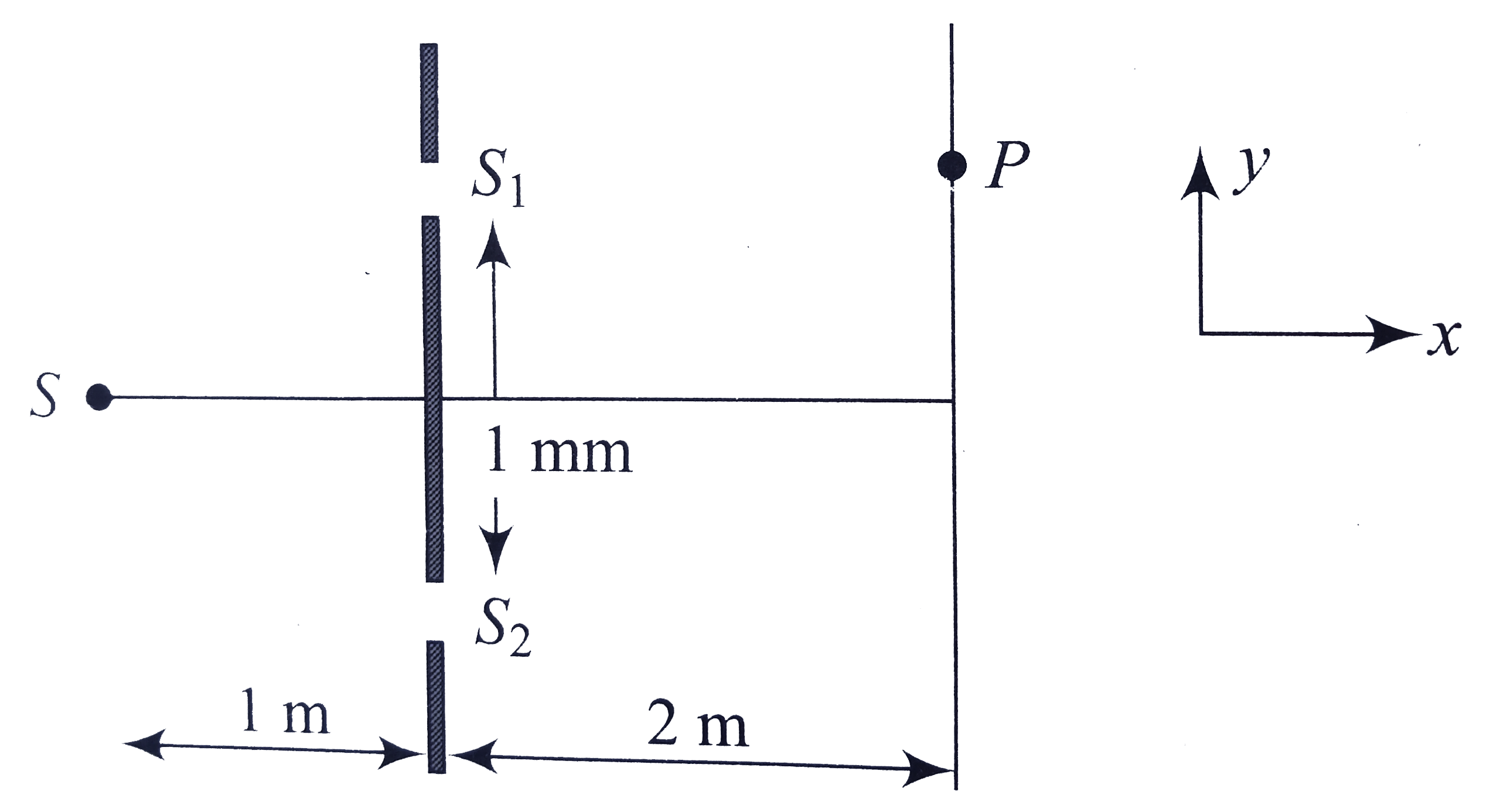
The correct Answer is:(a)y′=−sinπt (b)16s
Step by step video, text & image solution for In young's double-slit experiment set up, sources S of wavelength 50 nm illumiantes two slits S_(1) and S_(2) which act as two coherent sources. The sources S oscillates about its own position according to the equation y = 0.5 sin pi t, where y is in nm and t in seconds. The minimum value of time t for which the intensity at point P on the screen exaclty in front of the upper slit becomes minimum is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In a modified YDSE the sources S of wavelength 5000 A oscillates about axis of setup according to the equation y=0.5sin(π6)t where y is in millimeter and t in second. At what time ti will the intensity at P, a point exactly in front of slit S1 be maximum for the first time?
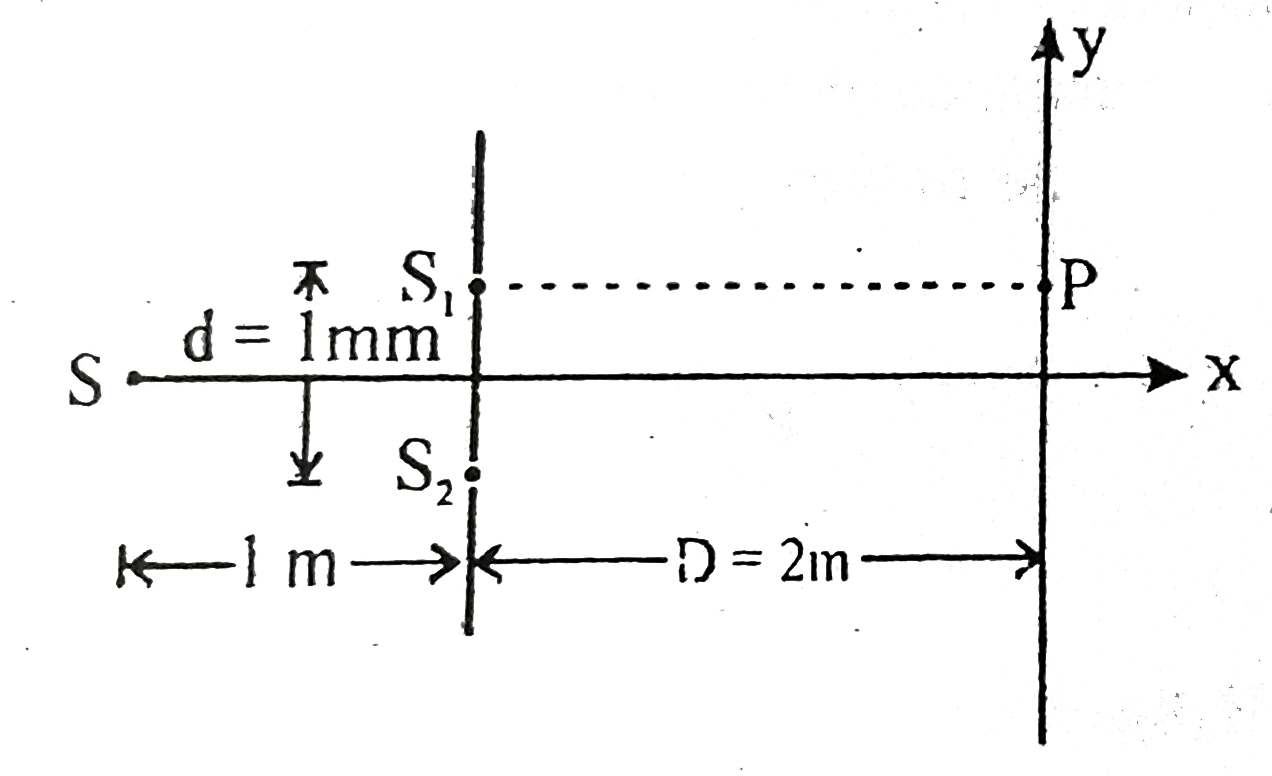
View SolutionIn young’s double slit experiment with a source of light of wavelength 6320Å, the first maxima will occur when
View Solution
Knowledge Check
In a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
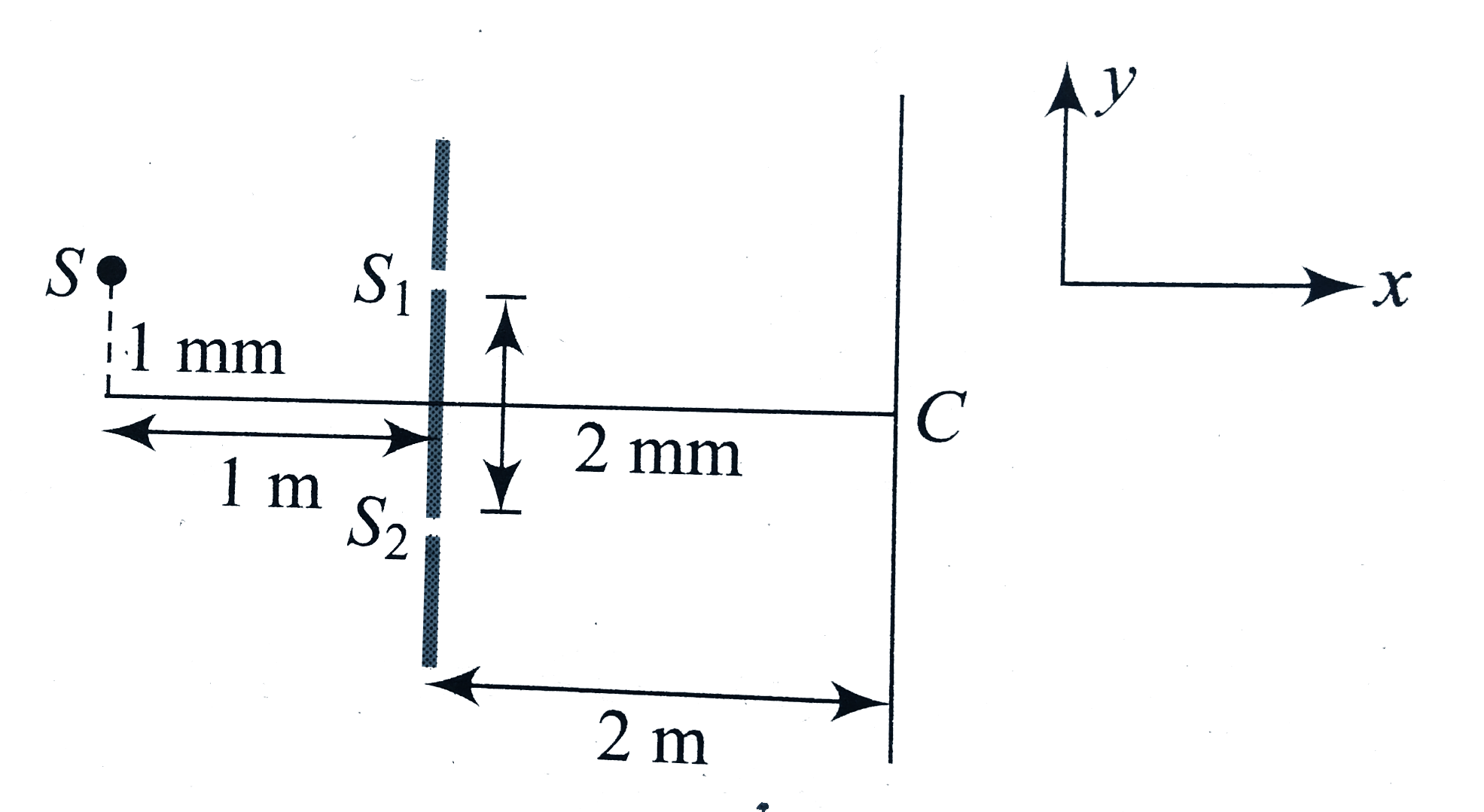
At t=2 s, the position of central maxima isA2 mm above CB2 mm below CC4 mm above CD4 mm below CIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
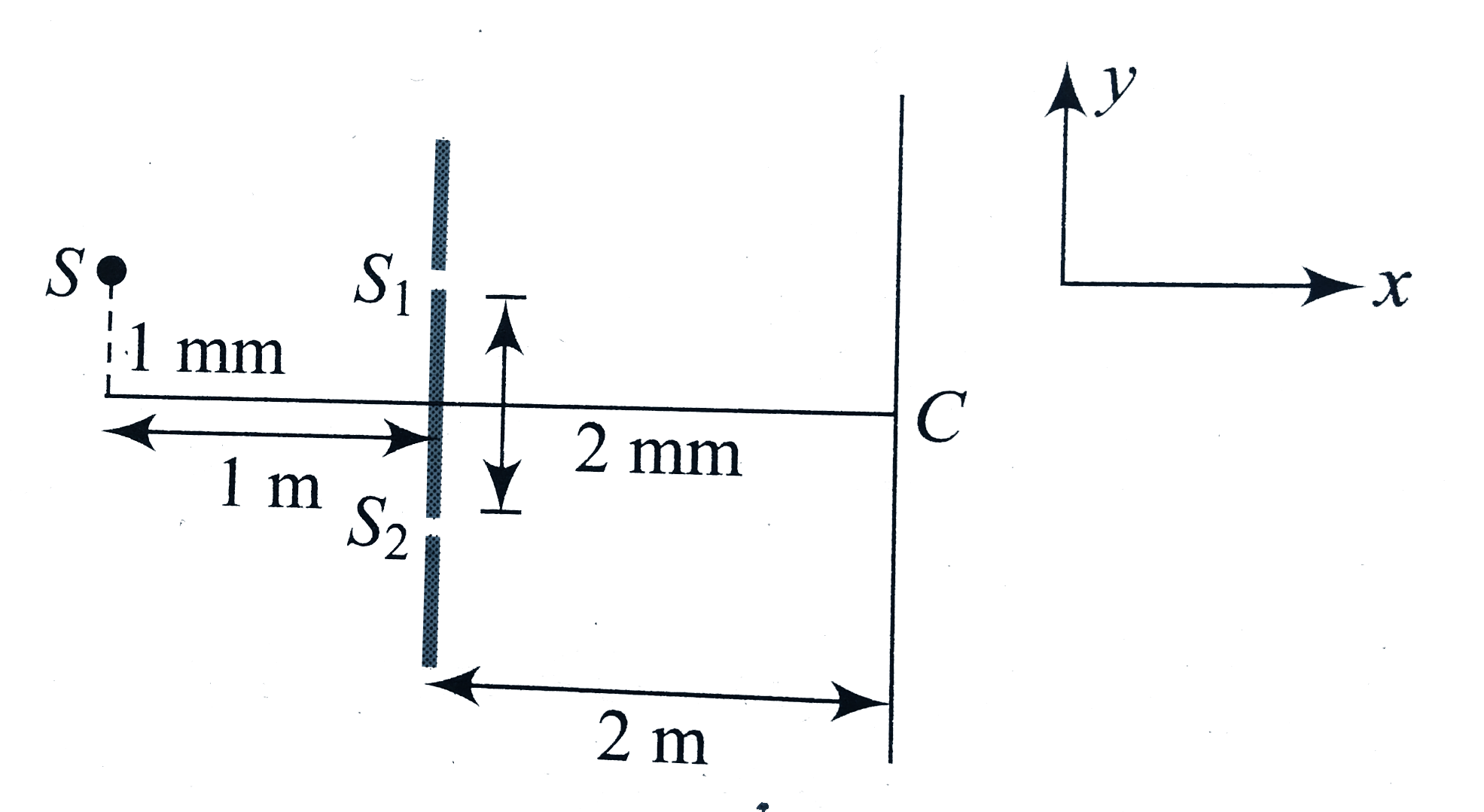
At t=0, fringe width is β1, and at t=2 s, width of figure is β2. ThenAβ1>β2Bβ2>β1Cβ1=β2Ddata is insufficientIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
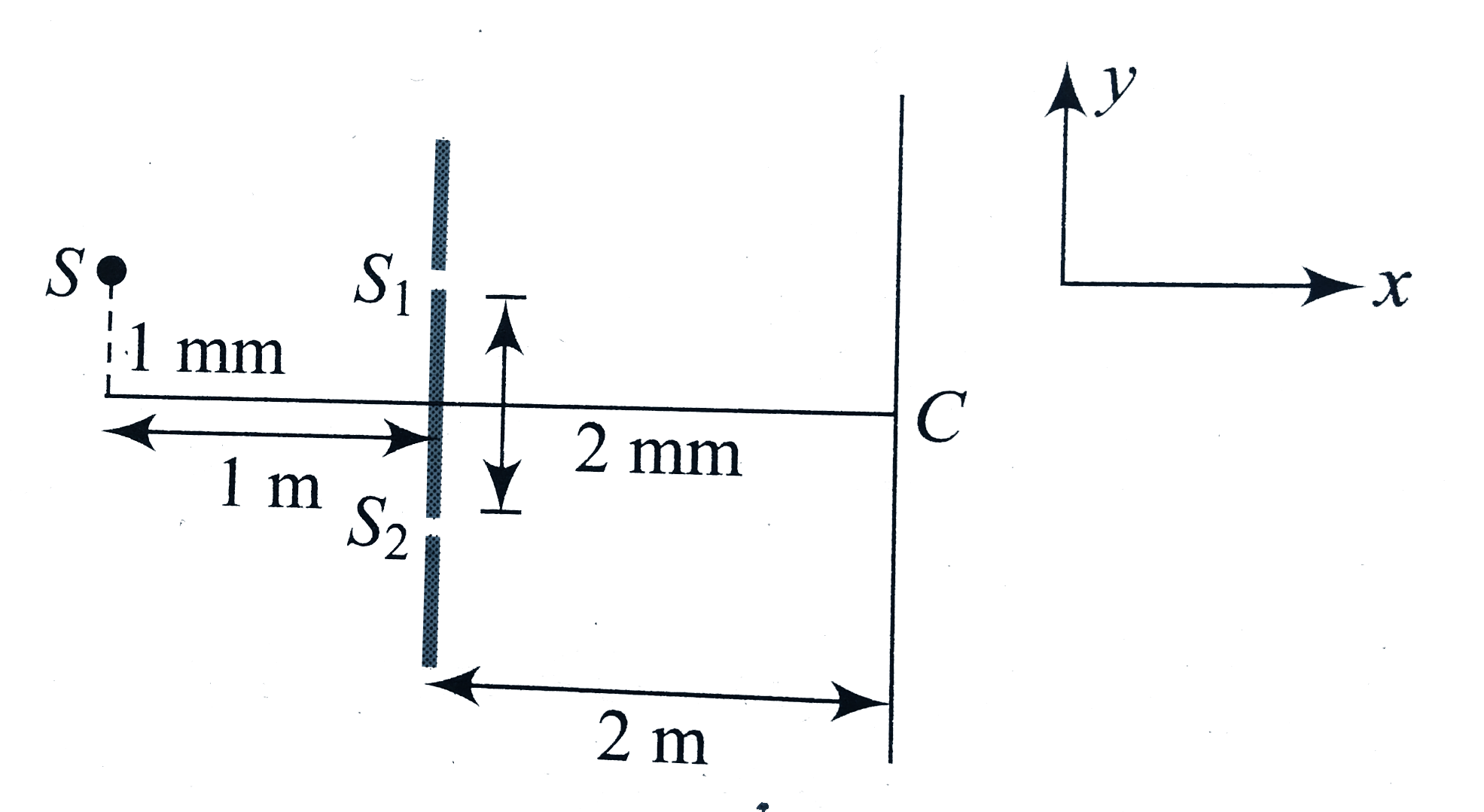
At t=1 s, a slab of thickness 2×10−3mm an refractive index 1.5 is placed just in front of S1. The central maximum is formed atA1 mm above CB1 mm below CC2 mm above CD2 mm below C
Figure shows a Young's double slit experiment set-up. The source S of wavelength 4000Å oscillates along y-axis according to the equation y−sinπt, where y is in millimeters and t is in seconds. The distance two slits S1andS2 is 0.5 mm.

View SolutionIn Young's double slit experiment, the ratio of maximum and minimum intensities in the fringe system is 9:1 the ratio of amplitudes of coherent sources is
View SolutionS1andS2 are two coherent sources. The intensity of both sources are same. If the intensity at the point of maxima is 4Wm−2, the intensity of each source is
View SolutionIn a Young’s double slit experiment, the source illuminating the slits is changed from blue to violet. The width of the fringes
View SolutionIn young's double slit experiment, distance between the slit S1andS2 is d and the distance between slit and screen is D . Then longest wavelength that will be missing on the screen in front of S1 is
View Solution
