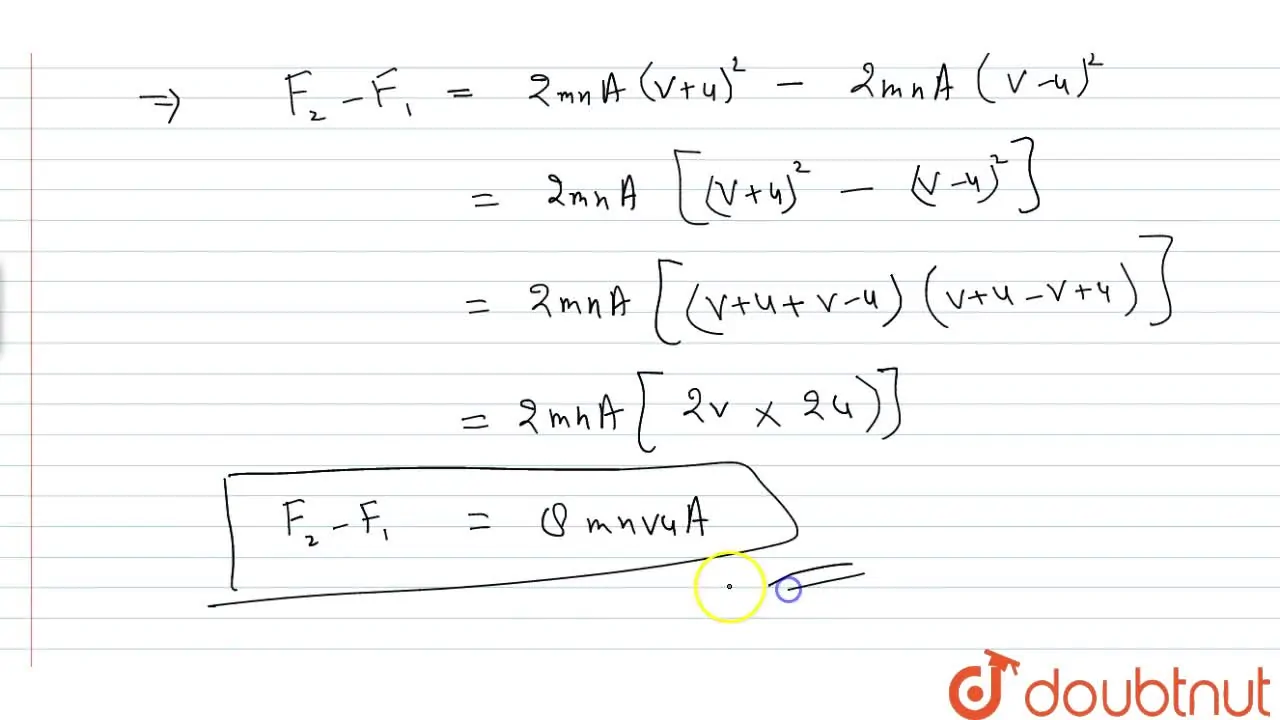
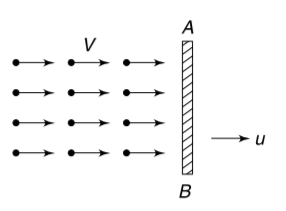
A beam of gas molecules is incident normally on a plate AB. Each gas molecule has mass m and velocity V. The incident beam falls on an area A on the plate and all the molecule strike the plate elastically. Number of molecules in unit volume of the beam is n. When the plate is moved to right (see fig.) a force F1 is needed to keep it moving with constant velocity u (>V) . When the plate is moved to left with constant velocity u, an external force F2 is needed. Find F2–F1.
The correct Answer is:8 m An Vu
Step by step video, text & image solution for A beam of gas molecules is incident normally on a plate AB. Each gas molecule has mass m and velocity V. The incident beam falls on an area A on the plate and all the molecule strike the plate elastically. Number of molecules in unit volume of the beam is n. When the plate is moved to right (see fig.) a force F_(1) is needed to keep it moving with constant velocity u (gt V) . When the plate is moved to left with constant velocity u, an external force F_(2) is needed. Find F_(2) – F_(1). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ARIHANTCHAPTER - KINETIC THEORY OF GASESEXERCISE - Level 34videos
KINETIC THEORY OF GASES
- BOOK - ARIHANTCHAPTER - KINETIC THEORY OF GASESEXERCISE - Level 34videos
KINETIC THEORY OF GASES
- BOOK - ARIHANTCHAPTER - KINEMATICSEXERCISE - Level 333videos
KINEMATICS
- BOOK - ARIHANTCHAPTER - MOMENTUM AND CENTRE OF MASSEXERCISE - Momentum And Center of Mass117videos
MOMENTUM AND CENTRE OF MASS
Similar Questions
The number of molecules per unit volume (n) of a gas is given by
View SolutionA molecule of mass m moving at a velocity v impinges elastically on the wall at an angle a with the wall. Then
View SolutionThe molecules of a gas move in
View SolutionIn a parallel plate capacitor, the distance between the plates is d and potential difference across the plate is V. Energy stored per unit volume between the plates of capacitor is
View SolutionA : Average velocity of gas molecules is zero,
R : Due to random motion of gas molecules, velocities of different molecules cancer each other.View SolutionA beam of light of wavelength 6400 Å is incident normally on a parallel glass plate of 5cm thick and R.I. 1.6. The beam takes the same time to travel from source to incident surface and through the glass plate. The distance of source from incident surface is
View SolutionA plate of mass M is held at rest by firing bullets from below. Each bullet has a mass m, velocity u (up) just before hitting and stops after hitting the plate for a moment and falls. Determine the number of bullets striking the plate Fig. per unit time
 View Solution
View SolutionA parallel beam of particles of mass m moving with velocity v impinges on a wall at an angle θ to its normal. The number of particles per unit volume in the beam is n. If the collision of particles with the wall is elastic, then the pressure exerted by this beam on the wall is
View SolutionA parallel beam of white light is incident normally on surface which absorbs 70% of the incident light and reflects the rest. If incident beam has power of 40 W , then what will be the magnitude of force exerted by the light on this surface ?
View SolutionA parallel beam of molecules moving with velocity v impinges on a wall at an angle θ to its normal. Find the pressure exerted by the beam on the wall assuming perfect elastic collisions. The concentration of the molecules in the beam is n.
View Solution