Given that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Product of possible values of k is
A
10
B
12
C
36
D
none of these
Video Solution
Text Solution
The correct Answer is:B
(x−2)(y+3)=k−6
(x−2)28+(y+3)212=1
x−2=X,y+3−Y
Xy=k−6 …..(1)
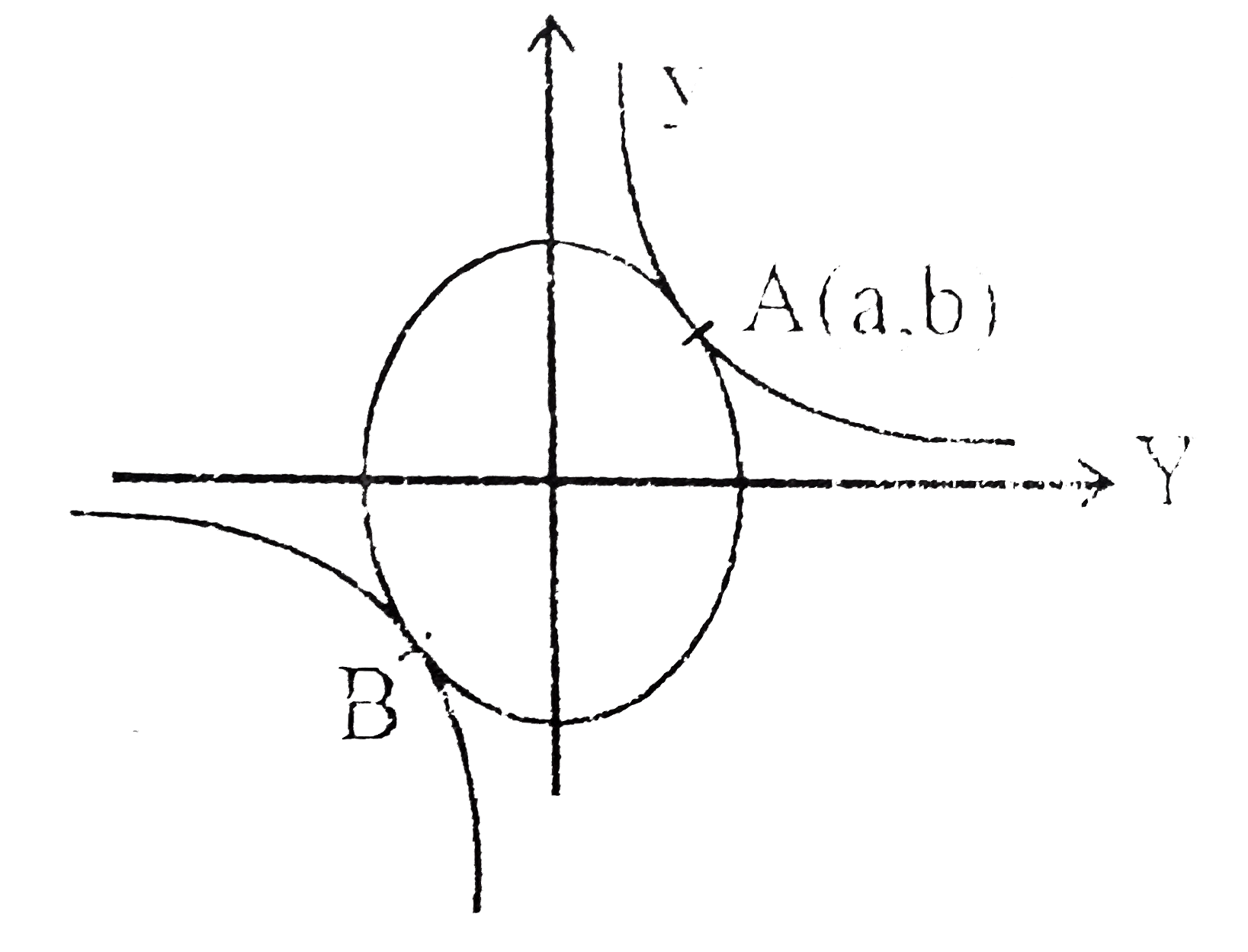
X28+Y212=1 ….(2)
At the point A and B, slopes of tangent to both curves must be equal
⇒y′=βα=−3α2β
2β2=3α2
since (α,β) satisfies equation (ii)
α28+β212=1
solving α=±2,β=±√6
α,β∈(2,√6)(−2,−√6)
α,β∈(2,−√6)(−2,√6)
k=6+2√6
k=6−2√6
coordinates of tangency
coordinates of tangency
(4,√6−3)(0,−√6−3)
(4,−√6−3)(0,√6−3)
|
Topper's Solved these Questions
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
48 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
51 Videos
- TEST PAPERSBOOK - ALLENCHAPTER - TEST PAPERSEXERCISE - part-2 Mathematics
18 Videos
- TEST PAPERBOOK - ALLENCHAPTER - TEST PAPEREXERCISE - CHEMISTRY SECTION-II
8 Videos
- VECTOR ALGEBRABOOK - ALLENCHAPTER - VECTOR ALGEBRAEXERCISE - All Questions
1 Videos
Similar Questions
Knowledge Check
Given that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Product of ordinates of A and B for fixed 'k' isA−6B−4C3Dnone of theseGiven that hyperbola xy−2y+3x=k is tangent to the ellipse 3x2−12x+2y2+12y+6=0 at two points A and B On the bases of above answer the following
Length of chord AB of ellipse isA√22B√30C√40D5Centre of the ellipse 3x2+4y2−12x−8y+4=0
A(1,1)B(2,1)C(3,1)DNone of these
If 3x+4y−k is the equation of the normal to the curve x2+2x−3y+3=0 at the point (1,2) ,then the value of k=
View SolutionThe tangent to the ellipse 3x2+16y2=12, at the point (1,34) intersects the curves y2+x=0 at :
View SolutionThe tangent to the ellipse 3x2+16y2=12, at the point (1,34), intersects the curve y + x = 0 at:
View SolutionIf the line x−2y=12 is tangent to the ellipse x2a2+y2b2=1 at the point (3,−92), then the length of the latus rectum of the ellipse is
View SolutionIf x+y=k is a tangent to the hyperbola x2−2y2=18,then sum of squares of possible values of k is
View Solution
