A point lilke paticle of mass 'm' (very small) is projected in the vertically upward direction where already exists, a uniform horizontal electric field ¯¯¯E. The field strength is such that qE=34mg, where q is the charge on the particle. After what time the radius of curvature of the charged particle will be minimum.
V0g
8V025g
16V025g
25V016g
The correct Answer is:C
When radius of curvature is minimum ¯v is ⊥ to ¯a & it is at t=16V025g
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
A particle is projected at a speed of u = 40 m/s in vertically upward direction in a place where exists a horizontal uniform electric field E0. The specific charge of the particle is 4g3E0 . (a) Find the time after projection, when speed of the particle will be least. (b) Find the time (after projection) when displacement of the particle becomes perpendicular to its acceleration. (c) Assuming that the particle has been projected from a great height and the electric field is present in large region, what angle the velocity of the particle will make with horizontal after a long time?
View SolutionA particle of mass m carries an electric positive charge Q and is subjected to the combined action of gravity g and a uniform horizontal electric field of strength E,it is projected with speed u to the vertical plane parallel to the electrical field and at an angle θto the horizontal .The maximum distance the particle travels horizontally when it is at the same level as its starting point is
View Solution
Knowledge Check
A particle of mass m and charge q is thrown in the vertical direction with a velocity v0 in a uniform horizontal electric field E. Assuming the gravity force to be negligible ,the equation of the path followed by the particle will be
Aparabola ,x=(qE2mv20)y2Bhyperbola , x=qE2mv20yCstraight line ,x=qEy2mv20Dnone of the aboveA pendulum bob of mass m charge q is at rest with its string making an angle θ with the vertical in a uniform horizontal electric field E. The tension in the string in
AmgsinθBmgCqEsinθDqEcosθa charged particle of mass 2 mg hang in air in an electric field of strength4NC directed upwards. the charge on the particle would be (g=10ms2)
A4×10−6 CB5×10−6 CC6×10−6 CD1.6×10−19 C
A particle of mass m and charge q is thrown in a region where uniform gravitational field and electric field are present. The path of particle:
View SolutionA charged particle is moving in a magnetic field of strength B perpendicular to the direction of the field. If q and m denote the charge and mass of the particle respectively, then the frequency of rotation of the particle is
View SolutionA charged particle of mass m and charge q is released from rest in an electric field of constant magnitude E. The kinetic energy of the particle after time t is
View SolutionA particle of mass m having negative charge −q is projected at an angle θ with x-axis. There exists uniform electric field E and a unifrom magnetic field B along x-axis. The particle will return to its intial point once if (n is some integer)
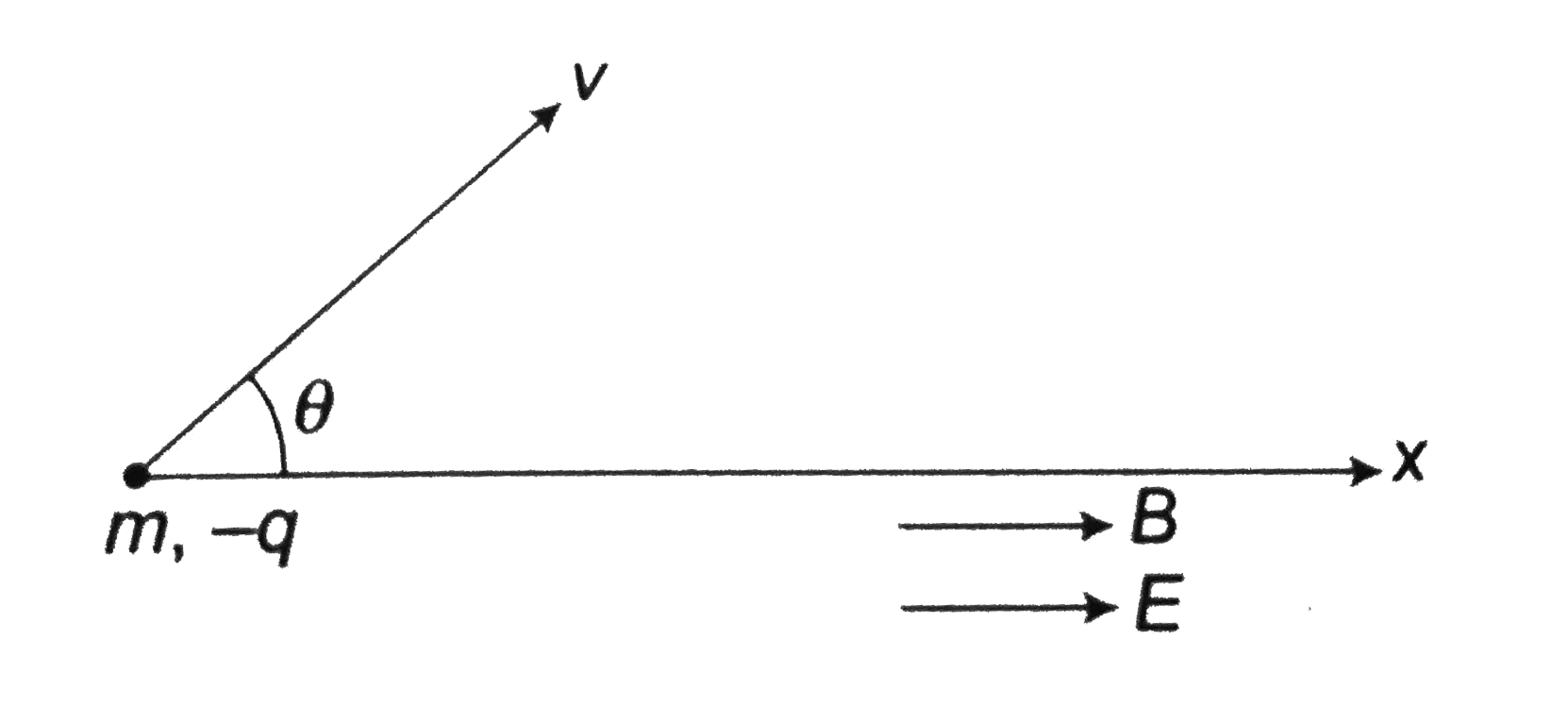
View SolutionA positively charged particle of charge q and mass m is suspended from a point by a string of length l. In the space a uniform horizontal electric field E exists . The particle is drawn aside so that it is projected horizontally with velocity v such that the particle starts to move along a circle with same constant speed v. Find the speed v.
View Solution
