A random variable X has the following probability distribution: Values of X:0 1 2 3 4 5 6 7 8 P(x):a 3a 5a 7a 9a 11a 13a 15a 17a Determine: (i). The value of a (ii) P(X<3), P(X≥3), P(0<X<5)
(i) here the sum of probabilities in a probability distribution is always equal to 1 .
∴P(X=0)+P(X=1)+P(X=2)+P(X=3)+P(X=4)+P(X=5)+P(X=6)+P(X=7)+P(X=8)=1
⇒a+3a+5a+7a+9a+11a+13a+15a+17a=1
⇒81a=1
⇒a=181
(ii)P(x<3)
=P(X=0)+P(X=1)+P(X=2)
=181+381+581
=981
=19
P(X≥3)
P(X=3)+P(X=4)+P(X=5)+P(X=6)+P(X=
=781+981+1181+1381+1581+1781
=7281
=89
P(0<X<5)
P(X=1)+P(X=2)+P(X=3)+P(X=4)
=381+581+781+981
=2481
=827
Topper's Solved these Questions
Similar Questions
A random variable X has the follwing probability distribution:
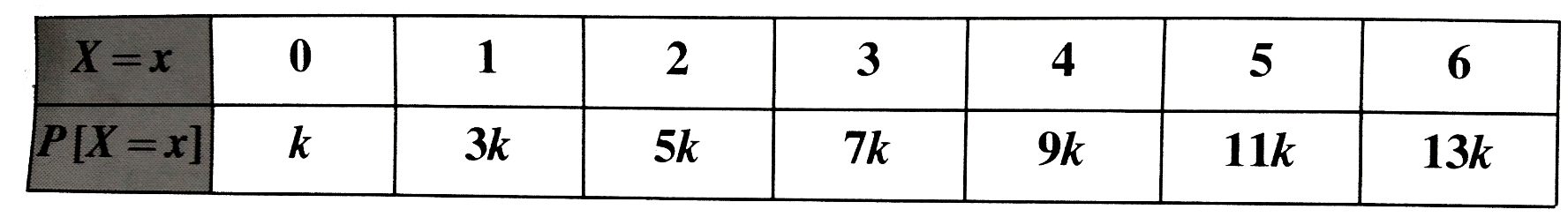
Find P(0<X<4)
View SolutionIf random variable X has the following probability distribution. Then the value of a is

View SolutionIf a random variable X has the following probability distribution values of X
Then P(X≥6) is equal to

View SolutionA random variable X has the following probability distribution: Values of X: −2 −1 0 1 2 3 P(X):0.1 k 0.2 2k 0.3 k
View SolutionA random variable X has the following probability distribution:Determine (i) k (ii) P(X<3)(iii) P(X>6) (iv) P(0<X<3)
View SolutionA random variable X has the following probability distribution:
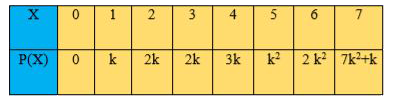
Determine : (i) k (ii) P(X<3) (iii) P(X>4)
View SolutionA random variable 'X' has the following probability distribution :

Find : (i) the value of K (ii) P(X≤1)
(iii) P(X≥0).
View SolutionA random variable X has the following probability distribution: X=xi: 1 2 3 4 P(X=xi): k 2k 3k 4k Write the value of P(X≥3) .
View SolutionA random variable X has the following probability distribution:
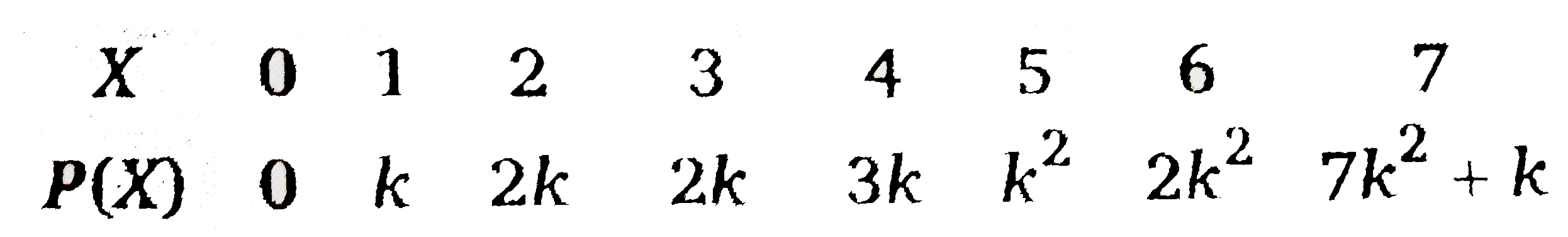
Determine :
(i) k
(ii) P(X<3)
(iii) P(X>6)
(iv) P(0<X<3)
View SolutionA random variable X has the following probability distribution :
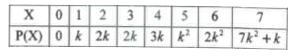
Determine
(i) k (ii) P(X<3)
(iii) P(X>6) (iv) P(0<X<3).
View Solution
