A number of capacitors each of capacitance 1μF and each one of which get punctured if a potential difference just exceeding 500 volt is applied, are provided. Then an arrangement suitable for giving a capacitor of 2μF across which 3000 volt may be applied requires at least-
18 component capacitors
36 component capacitors
72 component capacitors
144 component capacitors
The correct Answer is:C
To solve the problem, we need to determine how many capacitors of 1 µF each are required to create a capacitor of 2 µF that can withstand a voltage of 3000 volts without puncturing. Each capacitor can only handle a maximum voltage of 500 volts before it gets damaged.
1. Understanding the Voltage Requirement:
- We have a total voltage of 3000 volts that we want to apply across the arrangement of capacitors.
- Each capacitor can withstand a maximum of 500 volts.
2. Calculating the Number of Capacitors in Series:
- Since the voltage across capacitors in series adds up, we can calculate the number of capacitors needed in series to handle 3000 volts.
- The number of capacitors in series (n) can be calculated as:
n=Total VoltageVoltage per Capacitor=3000 volts500 volts=6
- Therefore, we need 6 capacitors in series to safely handle 3000 volts.
3. Calculating the Equivalent Capacitance of Capacitors in Series:
- The equivalent capacitance (C_eq) of capacitors in series is given by:
1Ceq=1C1+1C2+…+1Cn
- For n identical capacitors (1 µF each), this simplifies to:
Ceq=Cn=1μF6=16μF
4. Arranging Capacitors in Parallel:
- To achieve a total capacitance of 2 µF, we need to connect several branches of these series capacitors in parallel.
- If we denote the number of branches as n1, the total capacitance from these branches in parallel is:
Ctotal=n1⋅Ceq=n1⋅16μF
- We want this to equal 2 µF:
n1⋅16μF=2μF
- Solving for n1:
n1=2μF⋅6=12
5. Calculating the Total Number of Capacitors:
- Since we need 6 capacitors in series for each branch and we have 12 branches:
Total Capacitors=n1⋅6=12⋅6=72
Final Answer:
Therefore, the minimum number of capacitors required is 72.
- We have a total voltage of 3000 volts that we want to apply across the arrangement of capacitors.
- Each capacitor can withstand a maximum of 500 volts.
- Since the voltage across capacitors in series adds up, we can calculate the number of capacitors needed in series to handle 3000 volts.
- The number of capacitors in series (n) can be calculated as:
n=Total VoltageVoltage per Capacitor=3000 volts500 volts=6
- Therefore, we need 6 capacitors in series to safely handle 3000 volts.
- The equivalent capacitance (C_eq) of capacitors in series is given by:
1Ceq=1C1+1C2+…+1Cn
- For n identical capacitors (1 µF each), this simplifies to:
Ceq=Cn=1μF6=16μF
- To achieve a total capacitance of 2 µF, we need to connect several branches of these series capacitors in parallel.
- If we denote the number of branches as n1, the total capacitance from these branches in parallel is:
Ctotal=n1⋅Ceq=n1⋅16μF
- We want this to equal 2 µF:
n1⋅16μF=2μF
- Solving for n1:
n1=2μF⋅6=12
- Since we need 6 capacitors in series for each branch and we have 12 branches:
Total Capacitors=n1⋅6=12⋅6=72
Therefore, the minimum number of capacitors required is 72.
Topper's Solved these Questions
Similar Questions
The minimum number of capacitors (each of capacitance 2 μ F ) required to get an effective capacitance of 9 μ F is?
View SolutionThree capacitors of capacities 8μF,8μFand4μF are connected in a series and a potential difference of 120 volt is maintained across the combination. Calculate the charge on capacitor of capacity 4μF.
View Solution
Knowledge Check
A capacitor of capacitance 500μF is charged at the rate of 100μC/s. The time in which the potential difference will become 20V, is :
A100sB50 sC20 sD10 sMinimum number of capacitors of 2μF capacitance each required to obtain a capacitor of 5μF will be
AThreeBFourCFiveDSixThe capacitor of capacitance 4μF and 6μF are connected in series. A potential difference of 500 volts applied to the outer plates of the two capacitor system. Then the charge on each capacitor is numerically
A6000CB1200CC1200μCD6000μC
Three capacitors of 10,15 and 30 μF are connected in series and on this combination a potential difference of 60 V is applied. Calculate the charge, potential difference and energy stored on each capacitor.
View SolutionThree capacitors of capacitance 1μF,2μF and 3μF are connected in series and a potential difference of 11V is applied across the combination. Then, the potential difference across the plates of 1μF capacitor is
View SolutionMinimum number of capacitors of 4μF capacitance each required to obtain a capacitor of 10μF will be
View SolutionTwo capacitors A and B of capacitances 2μF and 5μF are connected to two battery as shown in figure The potential difference in volt between the plate of A is
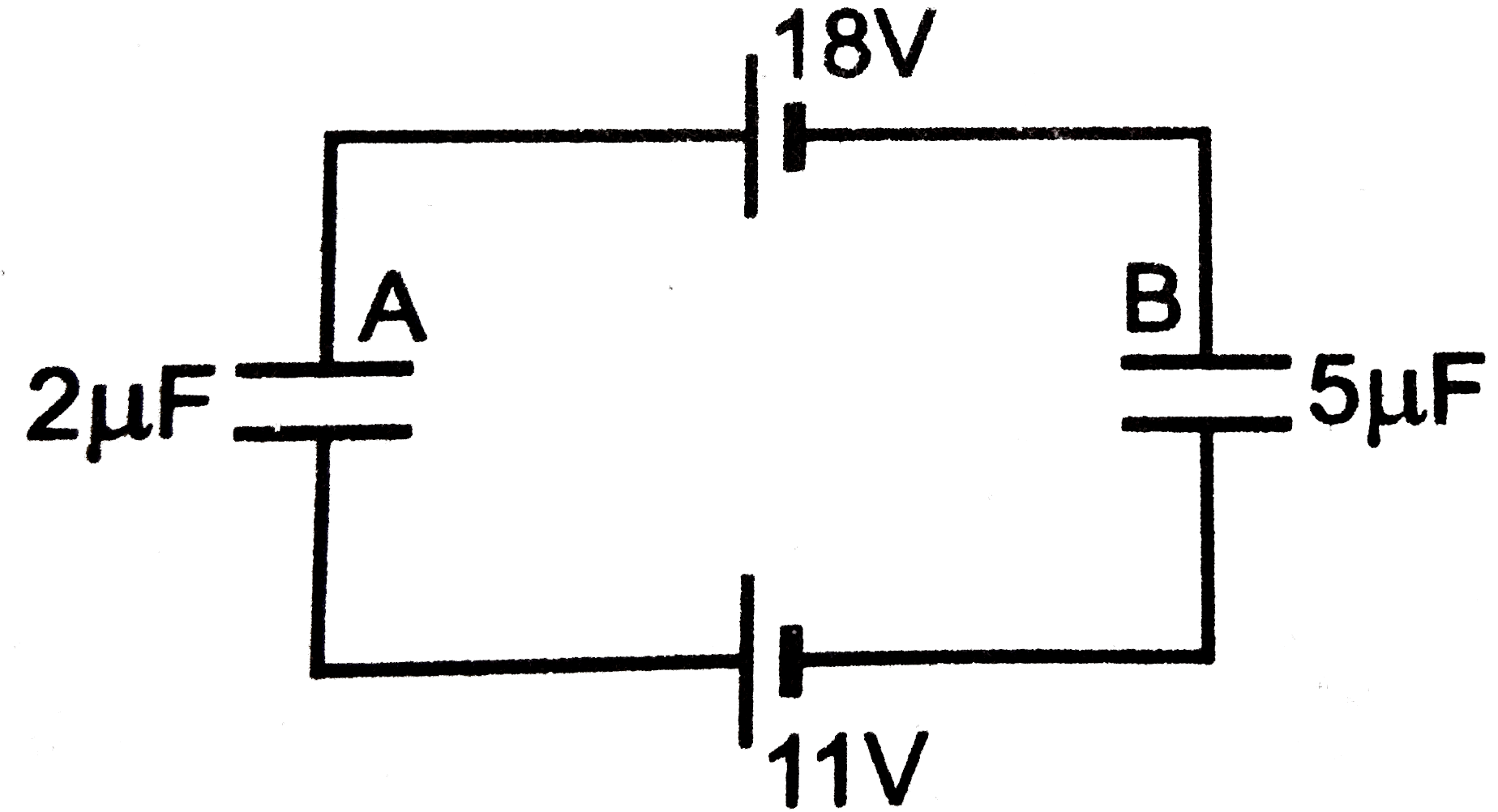
View SolutionTwo capacitors of capacitances 10μFand20μF are connected in series across a potential difference of 100V. The potential difference across each capacitor is respectively
View Solution
