A 15 kg mass is accelerated from rest with a force of 100 N. As it moves faster, friction and air resistance create an oppositively directed retarding force given by FR = A + Bv, where A = 25 N and B = 0.5 N/m/s. At what velocity does the acceleration equal to one half of the initial acceleration?
25ms−1
50m/s
75m/s
100m/s
The correct Answer is:C
To solve the problem step by step, we will follow the process of calculating the initial acceleration, then determining the velocity at which the acceleration is half of the initial acceleration.
Step 1: Calculate the Initial Acceleration
The initial acceleration can be calculated using Newton's second law of motion. The net force acting on the mass is the applied force minus the retarding force.
1. Given:
- Mass (m) = 15 kg
- Applied force (F) = 100 N
- Retarding force (FR) at rest = A = 25 N (since v = 0)
2. Calculate the net force:
Fnet=F−FR=100N−25N=75N
3. Using Newton's second law (F = ma):
a=Fnetm=75N15kg=5m/s2
Step 2: Set Up the Equation for Half of the Initial Acceleration
We need to find the velocity at which the acceleration equals half of the initial acceleration.
1. Calculate half of the initial acceleration:
a2=5m/s22=2.5m/s2
2. Set up the equation for acceleration when the object is moving with velocity v:
The retarding force now includes both A and the term Bv:
FR=A+Bv=25N+0.5N/m/s⋅v
3. Using Newton's second law again:
F−FRm=a2
Substituting the values:
100N−(25N+0.5N/m/s⋅v)15kg=2.5m/s2
Step 3: Solve for Velocity v
1. Rearranging the equation:
100−25−0.5v=15⋅2.5
75−0.5v=37.5
2. Isolate v:
75−37.5=0.5v
37.5=0.5v
v=37.50.5=75m/s
Final Answer
The velocity at which the acceleration equals one half of the initial acceleration is v=75m/s.
---
The initial acceleration can be calculated using Newton's second law of motion. The net force acting on the mass is the applied force minus the retarding force.
- Mass (m) = 15 kg
- Applied force (F) = 100 N
- Retarding force (FR) at rest = A = 25 N (since v = 0)
Fnet=F−FR=100N−25N=75N
a=Fnetm=75N15kg=5m/s2
We need to find the velocity at which the acceleration equals half of the initial acceleration.
a2=5m/s22=2.5m/s2
The retarding force now includes both A and the term Bv:
FR=A+Bv=25N+0.5N/m/s⋅v
F−FRm=a2
Substituting the values:
100N−(25N+0.5N/m/s⋅v)15kg=2.5m/s2
1. Rearranging the equation:
100−25−0.5v=15⋅2.5
75−0.5v=37.5
75−37.5=0.5v
37.5=0.5v
v=37.50.5=75m/s
The velocity at which the acceleration equals one half of the initial acceleration is v=75m/s.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A 5kg. mass is accelerated from rest to 60 m/s in 1 sec. what force acts on it:-
A5×60NB(5/60)×981NC602×52ND(52)×602×981NTwo forces 25 N and 16 N act on a body of mass 1.0 kg. the minimum acceleration will be
A25ms−2B16ms−2C9ms−2D41ms−2Two forces 25 N and 16 N act on a body of mass 1.0 kg. The minimum acceleration will be
A25ms−2B16ms−2C9ms−2D41ms−2
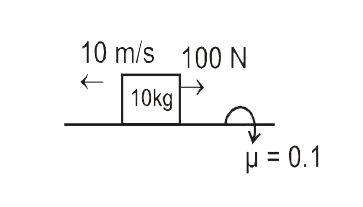
A block is given a velocity of 10 m/s and a force of 100 N in addition to friction force is also acting on the block. Find the retardation of the block?
View SolutionThe acceleration produced by a force of 5 N acting on a mass of 20 kg in m/s2 is :
View SolutionTwo forces 25 N and 16 N act on a body of mass 1.0 kg. The minimum acceleration will be
View SolutionThe acceleration produced by a force of 5N acting on a mass of 20 Kg in m/s2 is
View SolutionA body of mass 3 kg accelerates from 10 m/s to 20 m/s in 5 seconds due to the application of a force on it. Calculate the magnitude of this force (in N).
View Solution