What is the minimum value of f(x)=|2x−5|+6 ?
A
2
B
3
C
5
D
6
Video Solution
Text Solution
The correct Answer is:D
The minimum value of the absolute value expression is 0(when x=52) since it cannot have a negative value. f(52)=∣∣∣2(52)−5∣∣∣+6=6. So 6 is the minimum value of the function.
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
f(x)=x+4
g(x)=6−x2
What is the maximum value of g(f(x)) ?A−6B−4C2D6
If f(x)=|x+1|−1, what is the minimum value of f(x) ?
View SolutionFind the minimum value of
f(x)=|x+2|+|x−2|+|x|.
View SolutionFind the maximum and the minimum values of f(x)=−|x−1|+5 for all x∈R , if any.
View SolutionWrite the minimum value of f(x)=x+1x, x>0
View Solutionf(x)=33√x−5and g(x)=2px+q2. If f(g(2))=7,what is the minimum value of (p+q) if p is a positive integer?
View SolutionThe minimum value of y=5x2−2x+1 is
View Solution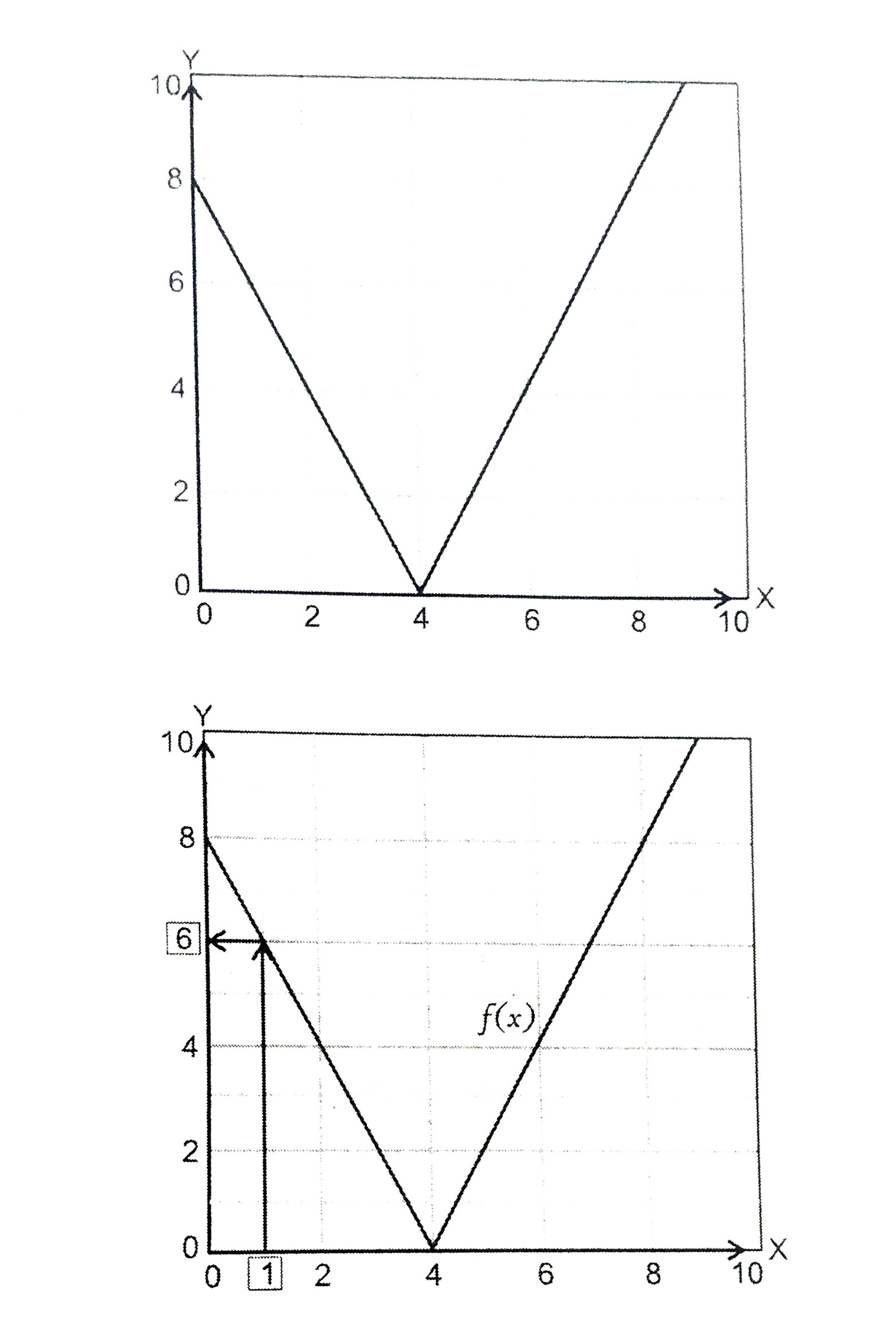
The graph of y=f(x) is shown below. If f(k)=6, then what is the minimum value of m so that f(m)=k?
View Solution