In Figure PQRS is a square. What is the slope of segment QR ?
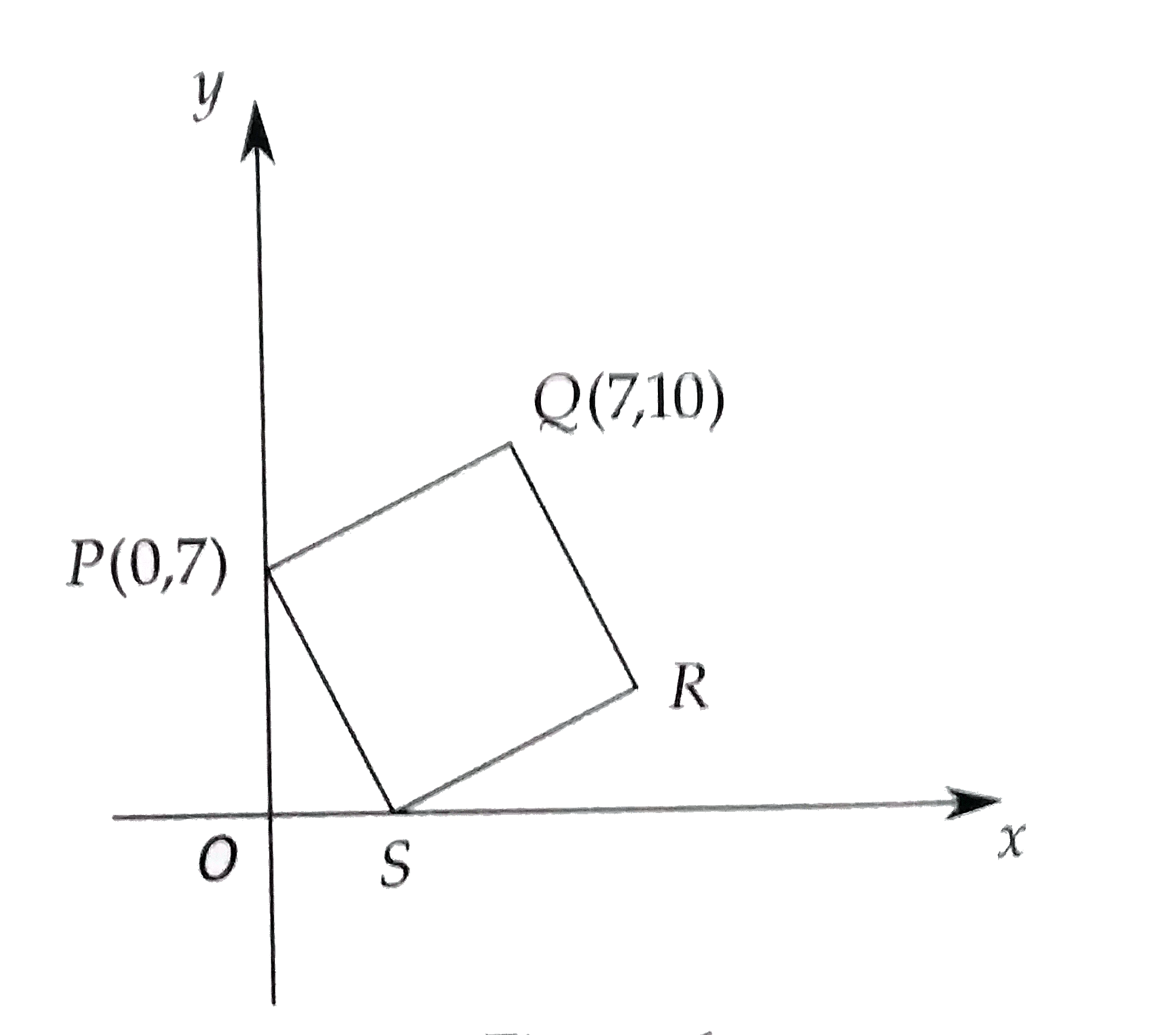
−103
−73
−43
37
The correct Answer is:B
The slope m of a line that goes through the points (x1,y1) and (x2,y2) is given by m=y2−y1x2−x1. The slope of PQ is 10−77−0=37. Since PQRS is a square, QR is perpendicular to PQ. When two lines are perpendicular and the lines are not parallel to the coordinate axes (the coordinate axes are the x - axis and the y - axis), the slopes of the lines are negative reciprocals. Since QR is perpendicular to PQ, the slope of QR is the negative reciprocal of the slope of PQ . The slope of QR is −1(37), which is −73.
Topper's Solved these Questions
Similar Questions
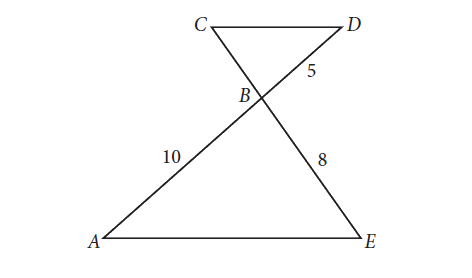
In the figure above, AE || CD and segment AD intersects segment CE at B. What is the length of segment CE ?
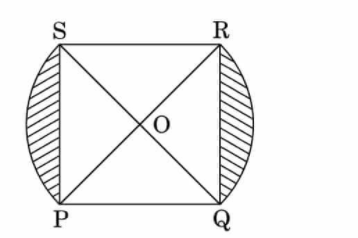
View SolutionIn the following figure, PQRS is square lawn with side PQ = 42 metres. Two circular flower beds are there on the sides PS and QR with centre at O, the intersections of its diagonals. Find the total area of the two flower beds (shaded parts).
View Solution
Knowledge Check
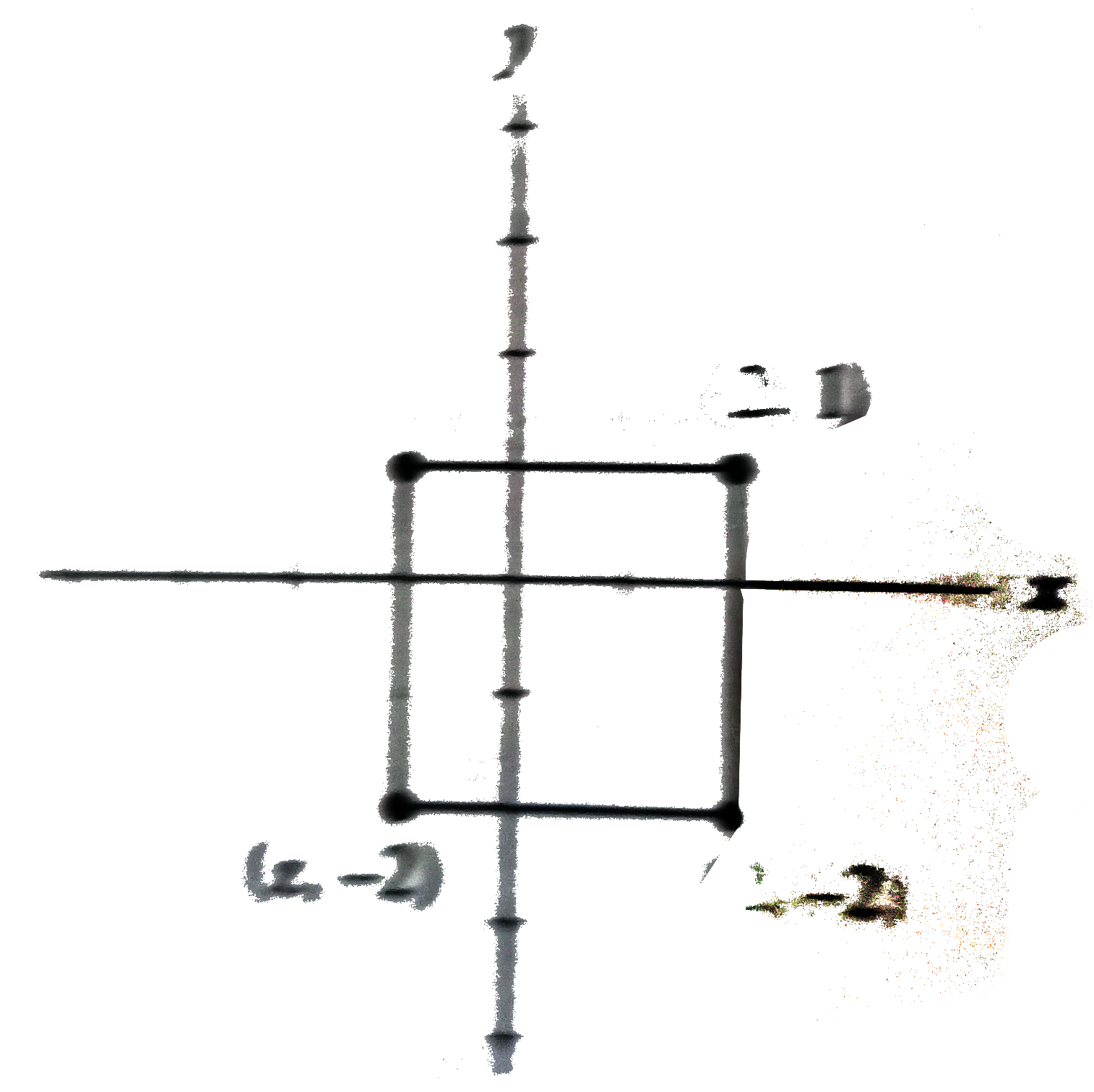
If the figure above is a square, what is the value of a?A−2B−1C1D2In the figure above, if VO=VY, what is the slope of segment VO?
A−√3B−√32C√32Dcannot be determined from the given information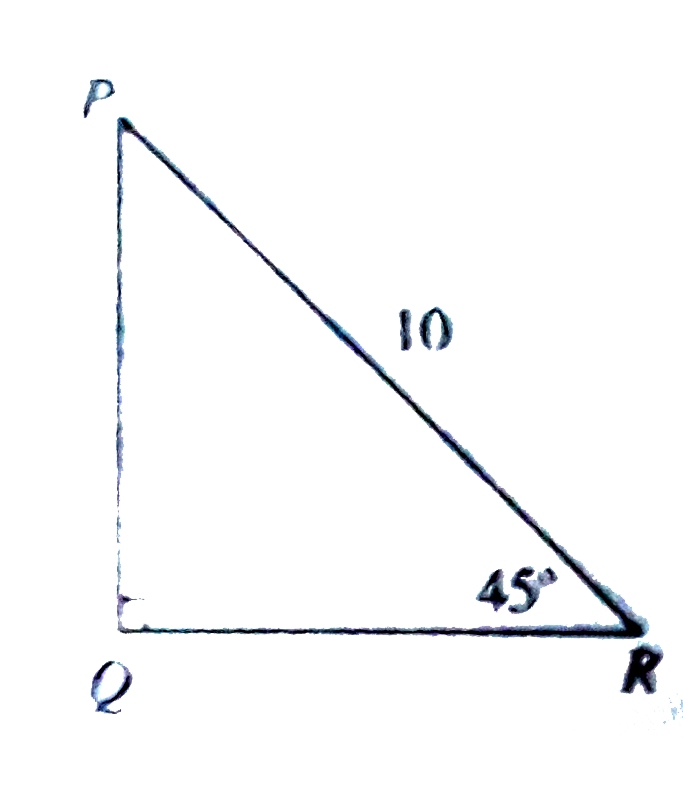
What is the length of ¯¯¯¯¯¯QR ?A5B5√22C10√2D5√2
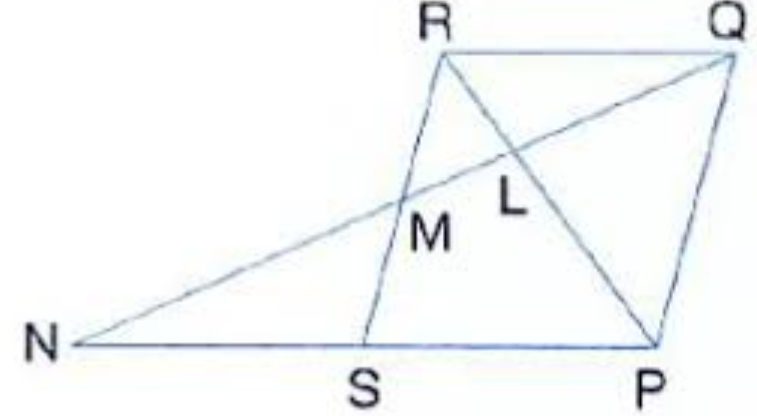
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm. L is a point on PR such that RL:LP=2:3. QL produced meets RS at M and PS produced at N.
Find the lengths of PN and RM.
View Solution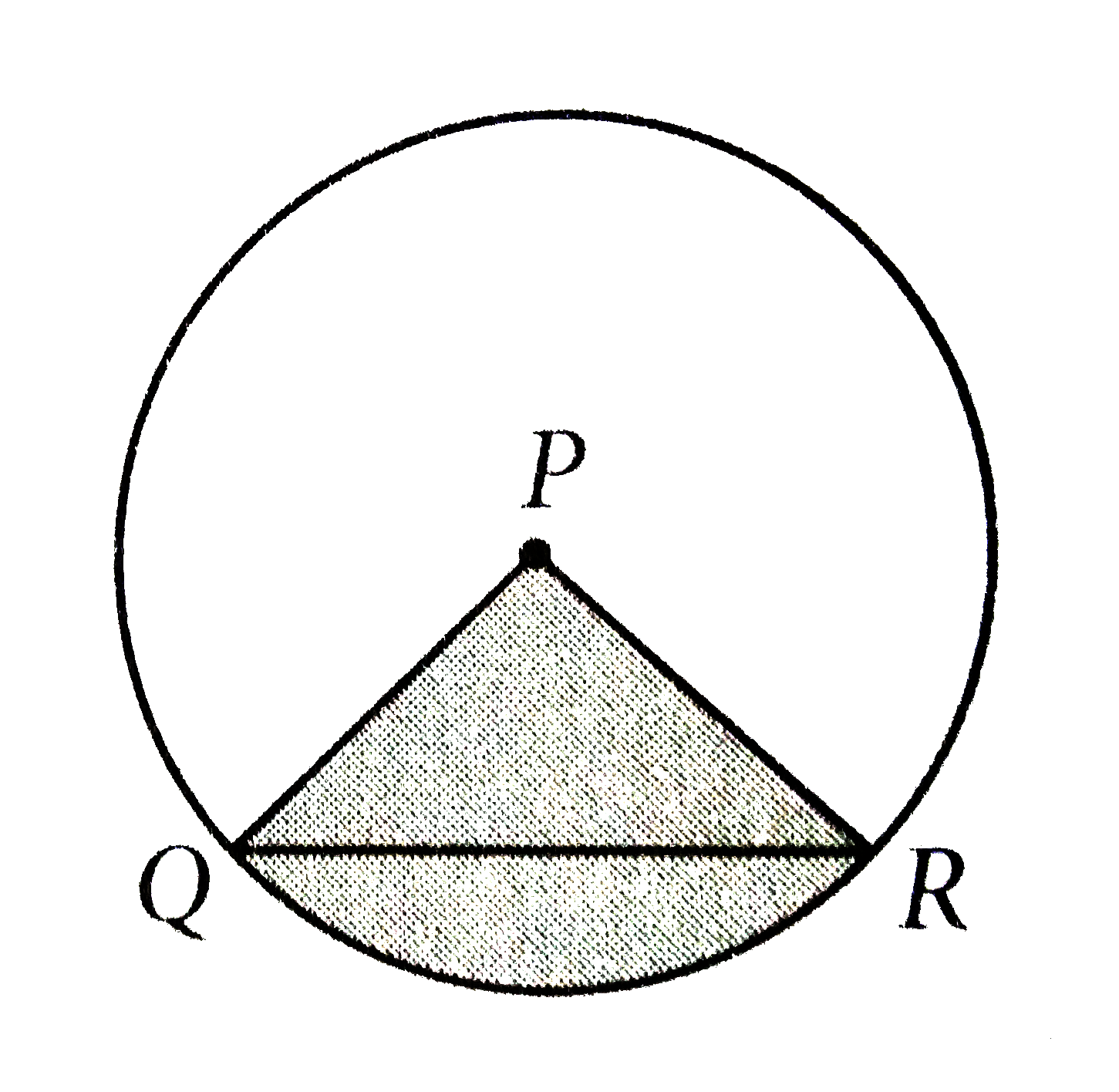
The area of the shaded sector in circle P above is 18π square units. If the measure of angle PQR is 452, what is the length of chord QR?
View Solution
In the figure below, rectangular LMNO has dimensions of 18 by 9. Segments PQ and RS are diagonals of the square shown. What is the area of the shaded region?
View Solution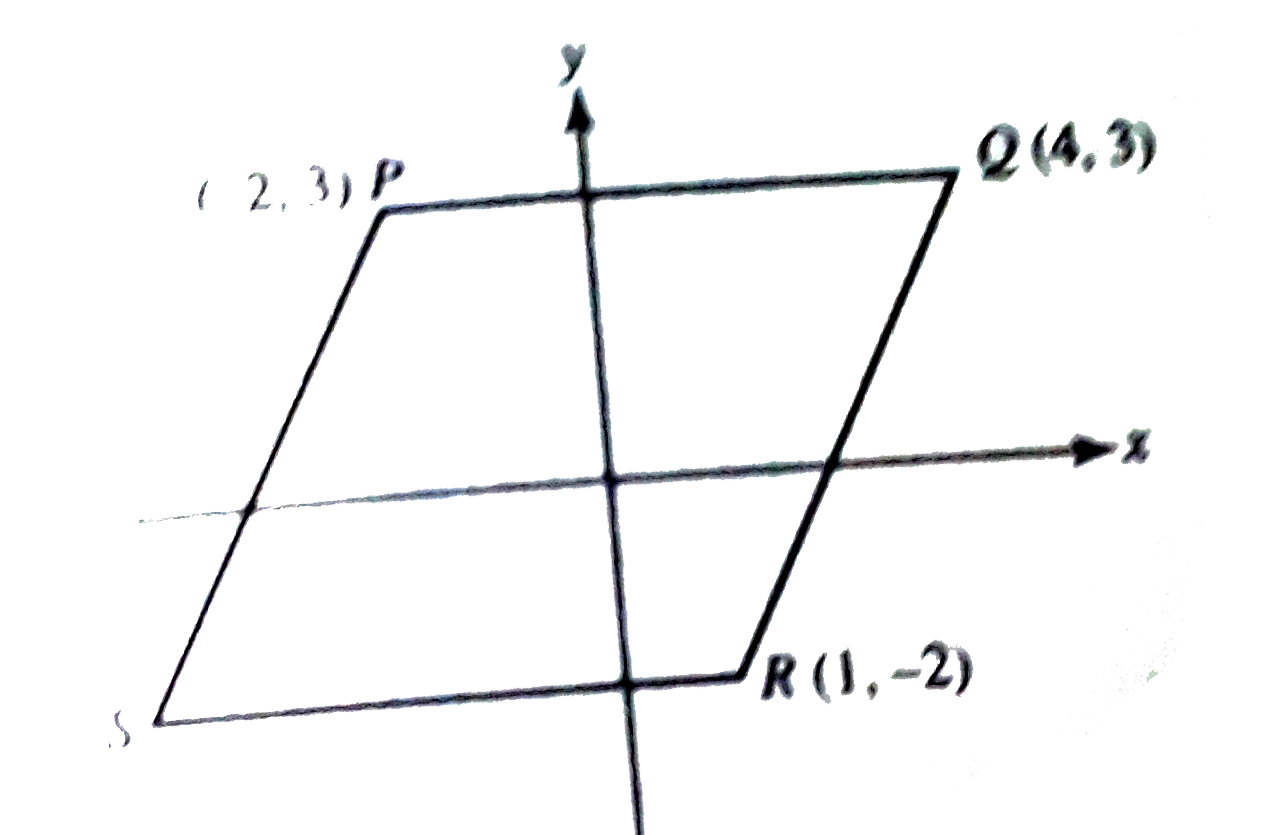
In the diagram above , PQRS is a parallelogram. What is the area of PQRS ?
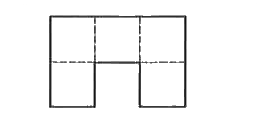
View SolutionThe 8-sided figure below is divided into 5 congruent squares. The total area of the 5 squares is 125 square inches. What is the perimeter, in inches, of the figure?
View Solution
