The lateral area of the right circular cone is $60 π$ . If the radius of the base is 6, what is the volume of the cone

A

$96 π$

B

$108 π$

C

$120 π$

D

$184 π$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

The formula for the lateral surface area of a cone is in terms of c = circumference and l = slant height. You can use the given base radius to solve for the slant height :
Leteral area $= \frac{1}{2} c l = \frac{1}{2} (2 π r) l =$
$\frac{1}{2} (2 π \times 6) l = 6 π l = 60 π$
$6 l = 60$
$l = 10$
You can think of the slant height as the hypotenuse of a right triangle whose legs are the base radius and height of the cone.

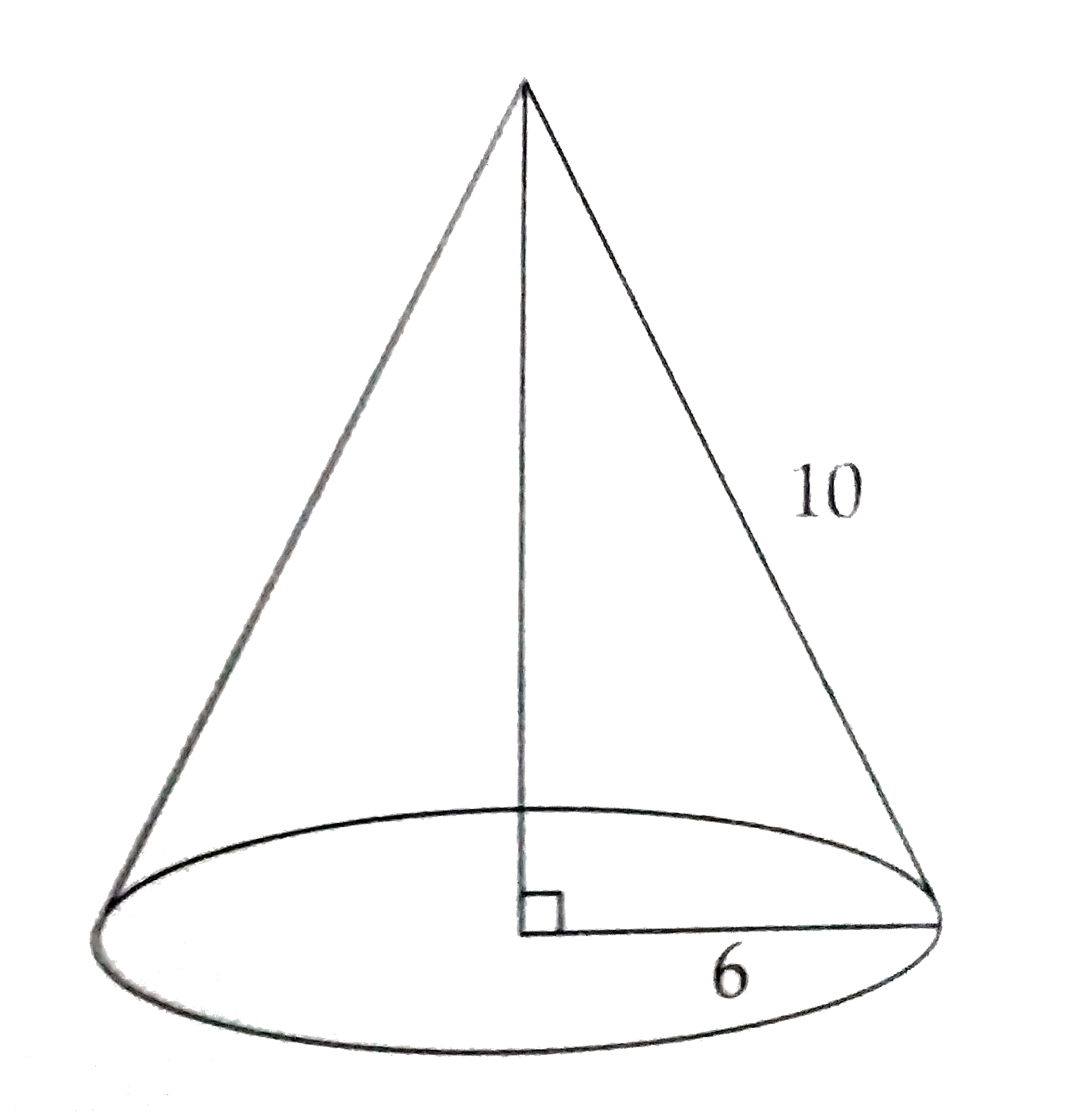
Now you can see that the triangle is a 3-4-5 and that h = 8. Plug r = 6 and h = 8 into the volume formula :
Volume $= \frac{1}{3} π r^{2} h = \frac{1}{3} π {(6)}^{2} (8) = 96 π$

|

Updated on:21/07/2023

Class 10 MATHS COORDINATE GEOMETRY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A formula for the volume of a right circular cone is $V = \frac{1}{3} π r^{2} h$ , where r is the radius of the base and h is the height of the cone. Using $\frac{22}{7}$ as an approximate value for $π$ , which of the following values is closest to the volume, in cubic inches, of a cone with height 28 inches and radius 6 inches?
A264
B352
C1056
D4224

KAPLAN-SOLID GEOMETRY -SOLID GEOMETRY FOLLOW - UP TEST

Home
Ask Doubt
Profile