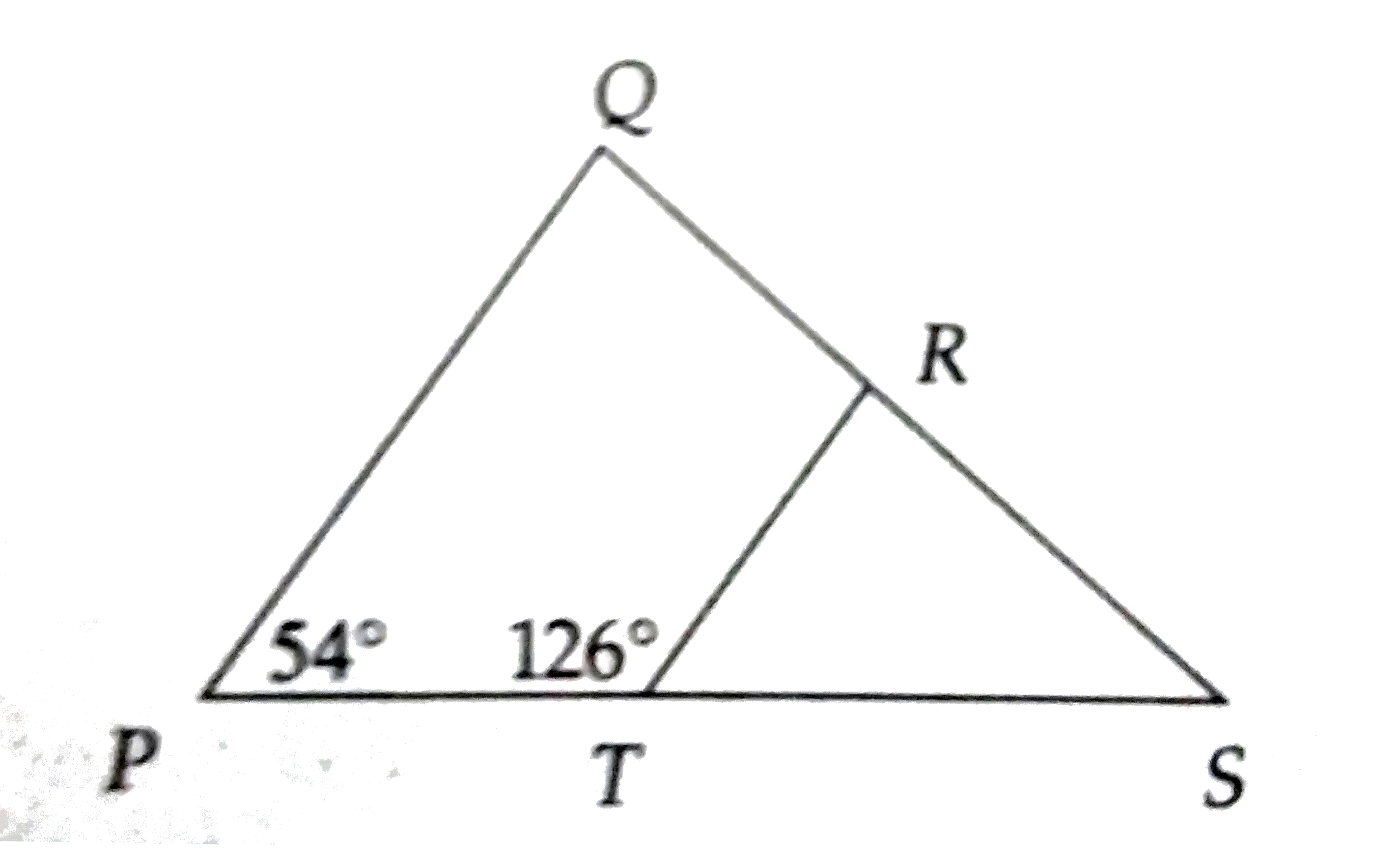
¯¯¯¯¯¯QR=¯¯¯¯¯RS. If the area of ΔRST is c2, what is the area of ΔQSP ?
A
c√2
B
c√3
C
c
D
2c
Video Solution
Text Solution
The correct Answer is:D
You might wonder here how you're supposed to find the area of ΔQSP when you're given no lengths you can for a base or an altitude. The only numbers you have are the angle measures. They must be there for some reason - the makers never provide superfluous information. In fact, because the two angle measures provided add up to 180∘, you know that ¯¯¯¯¯¯PQ and ¯¯¯¯¯¯TR are parallel. And that, in turn you that ΔRST is similar to ΔQSP, because they have the same three angles.
Similar triangles are triangles that have the same shape : Corresponding angles are equal and corresponding sides are proportional. In this case, because it's given that ¯¯¯¯¯¯QR=¯¯¯¯¯RS, you know that ¯¯¯¯¯¯QS is twice ¯¯¯¯¯¯QR and that corresponding sides are in a ratio of 2 : 1. Each side of the larger triangle is twice the length of the corresponding side of the smaller triangle. That doesn't mean, however, that the ratio of the areas is also 2 : 1. In fact, the area ratio is the square of the side ratio, and the larger triangle has four times the area of the smaller triangle.
area of ΔQSP=4× area of ΔRST=4×c2=2c.
|
Updated on:21/07/2023
Related Playlists
- BOOK - KAPLANCHAPTER - PLANE GEOMETRY EXERCISE - PLANE GEOMETRY FOLLOW - UP TEST6videos
PLANE GEOMETRY
- BOOK - KAPLANCHAPTER - MISCELLANEOUS TOPICS EXERCISE - MISCELLANEOUS TOPICS FOLLOW - UP TEST12videos
MISCELLANEOUS TOPICS
- BOOK - KAPLANCHAPTER - PRACTICE TEST 1 EXERCISE - PRACTICE TEST50videos
PRACTICE TEST 1
Similar Questions
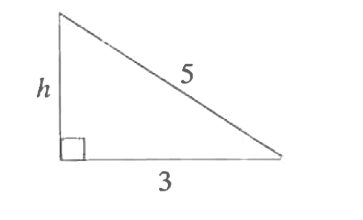
What is the area of the triangle?
 View Solution
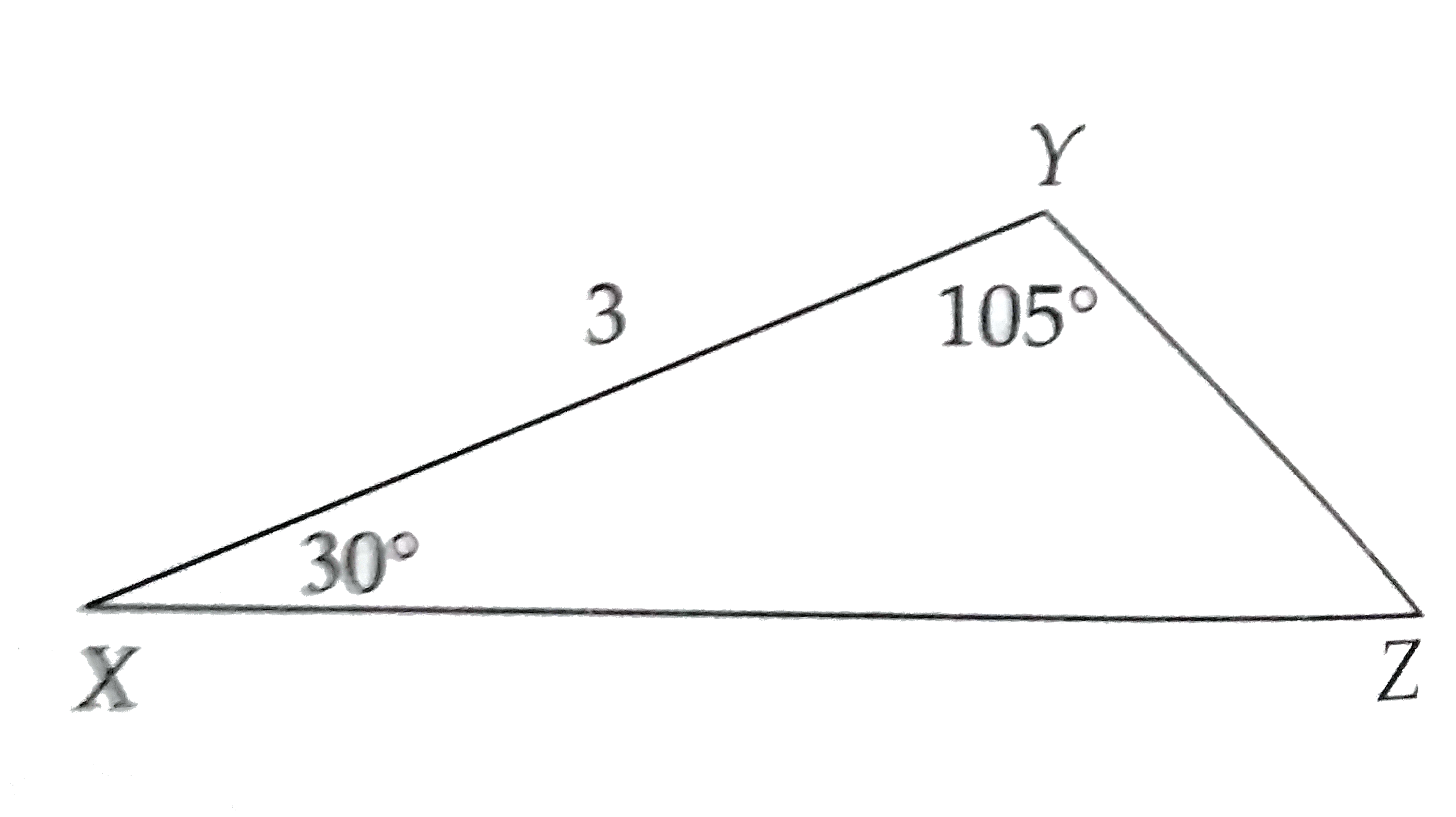
View SolutionIf ¯¯¯¯¯¯XY=3, what is the area of ΔXYZ ?
 View Solution
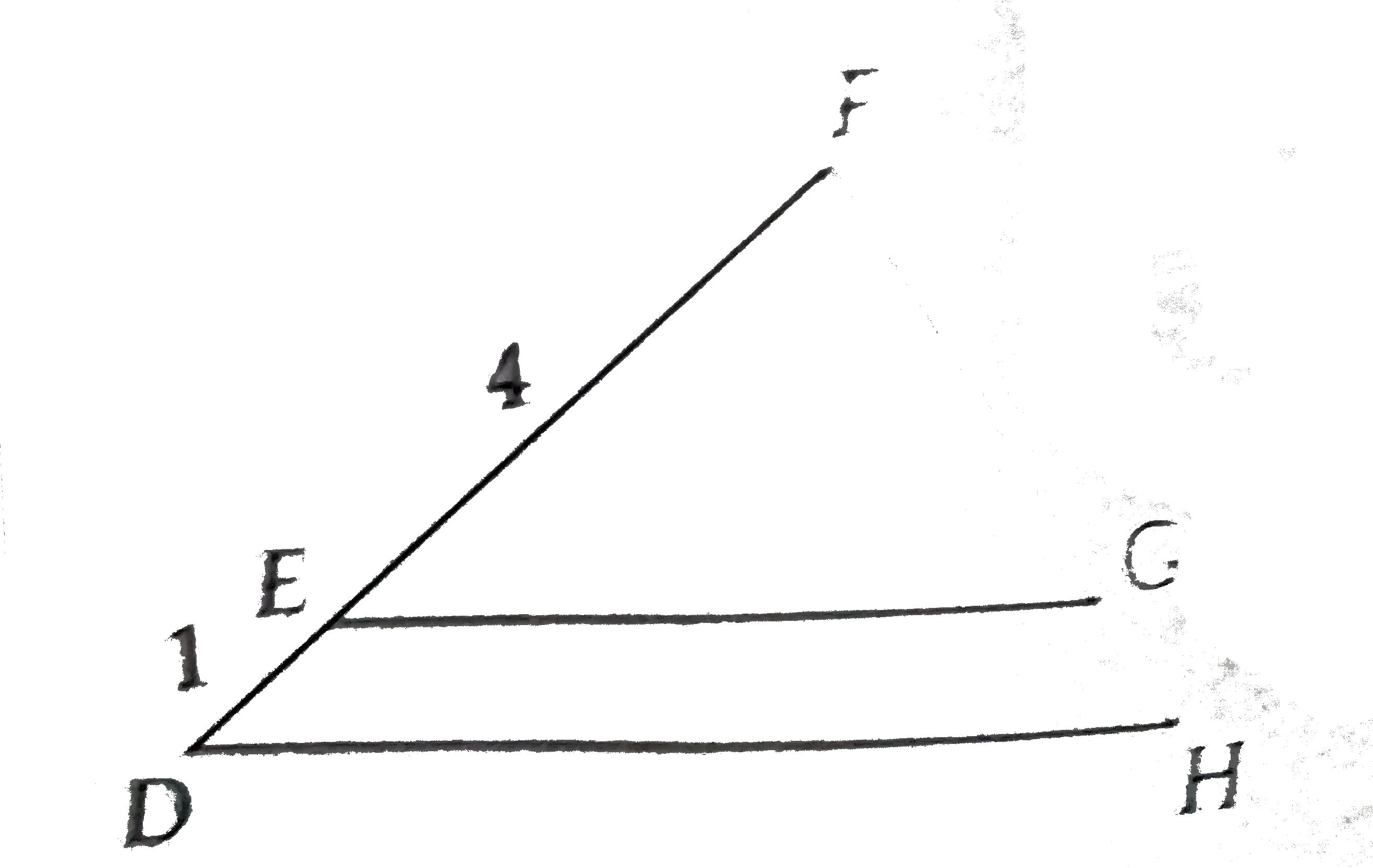
View Solution¯¯¯¯¯¯EG∣∣¯¯¯¯¯¯¯DH, and the length of segments ¯¯¯¯¯¯DE and ¯¯¯¯¯¯EF are as marked. If the area of ΔEFG is a, what is the area of ΔDFH in terms of a ?
 View Solution
View SolutionIn the figure, what is the area of ΔABC?
 View Solution
View SolutionIn the figure, what is the area of ΔABC?
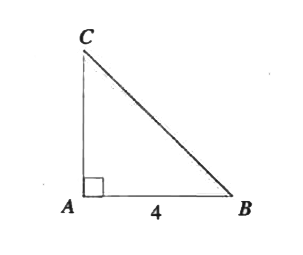 View Solution
View SolutionIf the area of triangle ABC is 15, what is the length of AC ?
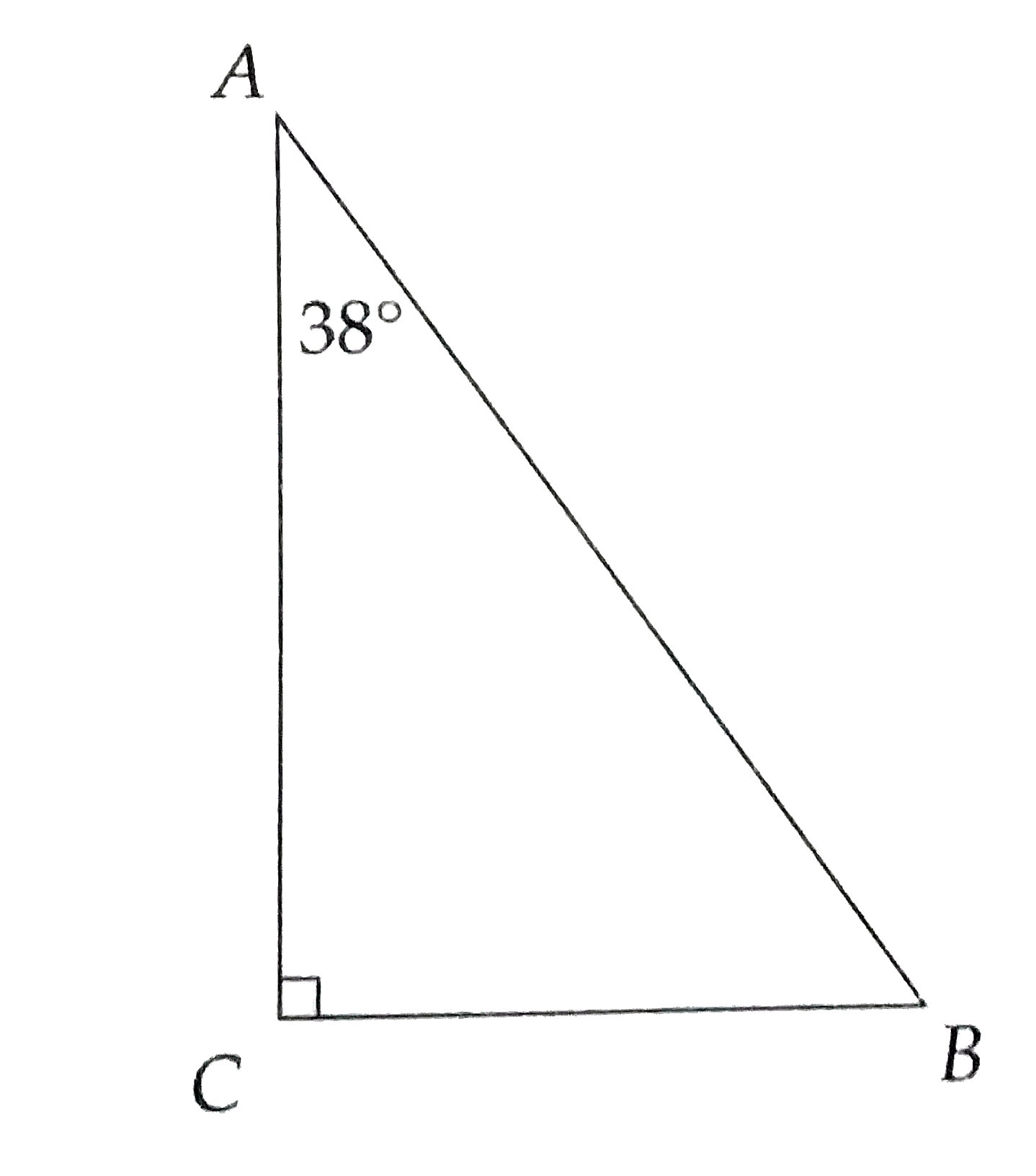 View Solution
View SolutionIf ABCD is a square and the area of ΔAFG is 10, then what is the area of ΔAEC ?
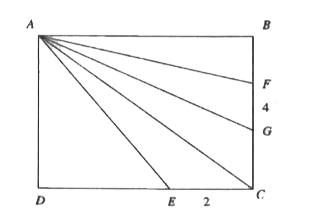 View Solution
View SolutionIf Δ=a2−(b−c)2,Δ is the area of the ΔABC then tanA= ?
View Solution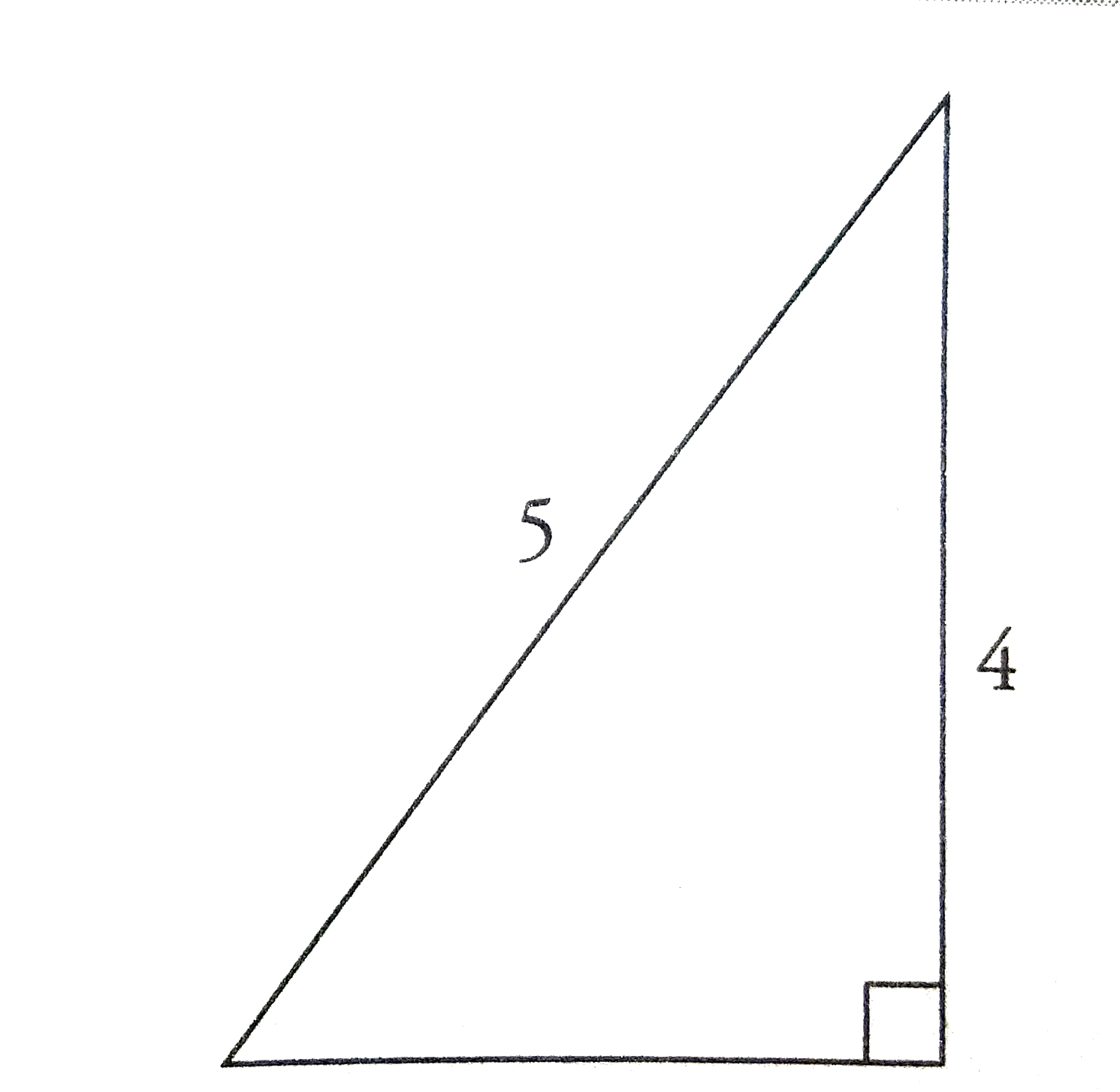
If the area of the triangle above is 6, what is its perimeter?View Solution
If the perimeter above is 18, what is the area of the triangle?View Solution


