If p=√q+zr2+z, what is the value of z in terms of p, q, and r ?
p−r2√qp−1
pr2−√qr2
√p+qrr2
√q−pr2p−1
The correct Answer is:D
To find the value of z in terms of p, q, and r from the equation
p=√q+zr2+z
we can follow these steps:
Step 1: Cross-multiply the equation
We start by cross-multiplying to eliminate the fraction:
p(r2+z)=√q+z
Step 2: Distribute p
Next, we distribute p on the left side:
pr2+pz=√q+z
Step 3: Rearrange the equation
Now, we rearrange the equation to isolate z:
pz−z=√q−pr2
Step 4: Factor out z
We can factor z out from the left side:
z(p−1)=√q−pr2
Step 5: Solve for z
Finally, we solve for z by dividing both sides by (p−1):
z=√q−pr2p−1
Thus, the value of z in terms of p, q, and r is:
z=√q−pr2p−1
We start by cross-multiplying to eliminate the fraction:
Next, we distribute p on the left side:
Now, we rearrange the equation to isolate z:
We can factor z out from the left side:
Finally, we solve for z by dividing both sides by (p−1):
Topper's Solved these Questions
- ALGEBRA Book:KAPLANChapter:ALGEBRA Exercise:ALGEBRA FOLLOW - UP TEST
Explore 10 Videos
- ADVANCED TECHINQUES FOR POLYNOMIALS AND RATIONAL EQUATIONSBook:KAPLANChapter:ADVANCED TECHINQUES FOR POLYNOMIALS AND RATIONAL EQUATIONSExercise:Multiple Choice Question
Explore 20 Videos
- CIRCLESBook:KAPLANChapter:CIRCLESExercise:Multiple Choice Question
Explore 12 Videos
Similar Questions
Knowledge Check
If p=4√3q, what is 3q, in terms of p?
Ap216Bp24C16p2Dp4If p+2√x−1=q, and q > p , what is x-1 in terms of p and q ?
A√q−p2B√q−p2C(q−p)22D(q−p)24If 1p+q=randp≠−q. What is p in terms of r and q?
Arq−1qB1+rqqCr1+rqD1−rqr
If p and q vary inversely, find the values of x,y and z.

View SolutionIf p and q vary inversely, find the values of x,y and z.

View SolutionFind the value of :
2p + 3q + 4r + por when p =-1,q = 2 and r = 3
View SolutionProve that the value of each the following determinants is zero: ∣∣ ∣ ∣∣a−bb−cc−ax−yy−zz−xp−qq−rr−p∣∣ ∣ ∣∣
View SolutionIn Figure 1, y=√3xandz=2x. What is the ratio p:q:r in figure 2?
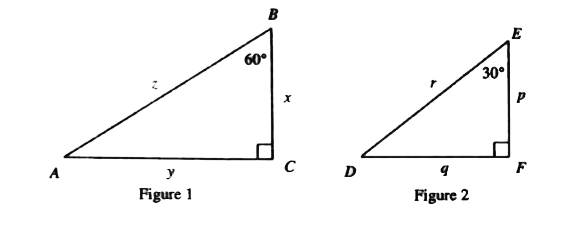
View Solution