A large number (n) of identical beads, each of mass m and radius r are strung on a thin smooth rigid horizontal rod of length L(L>>r) an dare at rest at random positions. The rod is mounted between two rigid supports (see figure). If one of the beads is now given a speed v, the average force experienced by each support after a lone time is (assume all collision are elastic) :-
mv22(L−nr)
mv2L−nr
zero
mv2L−2nr
The correct Answer is:D
Change In momentum in each collision with rigid support ΔP=2mv
time gap between two successive collision with same support Δt=2L−4nrv
Fave=ΔPΔt
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B19videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-IV B14videos
CENTRE OF MASS
- BOOK - ALLENCHAPTER - BASIC MATHEMATICS USED IN PHYSICS &VECTORS EXERCISE - EXERCISE-IV ASSERTION & REASON11videos
BASIC MATHEMATICS USED IN PHYSICS &VECTORS
- BOOK - ALLENCHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 5 B (Integer Type Questions)3videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
Similar Questions
Two identical spherical balls of mass M and radius R each are stuck on two ends of a rod of length 2R and mass M (see figure). The moment of inertia of the system about the axis passing perpendicularly through the centre of the rod is
 View Solution
View SolutionA rod of length / and radius r is held between two rigid walls so that it is not allowed to expand. If its temperature is increased, then the force developed in it is proportional to
View Solution
Knowledge Check
- Question 1 - Select One
Five identical balls each of mass m and radius r are string like beads at random and at rest along a smooth rigid horizontal thin rod of length L mounted between immovable supports Assume 10r≤L and that the collision between balls or between balls and supports are elastic if one ball is struck horizontally so as to acquire a speed v the average force felt by the support is
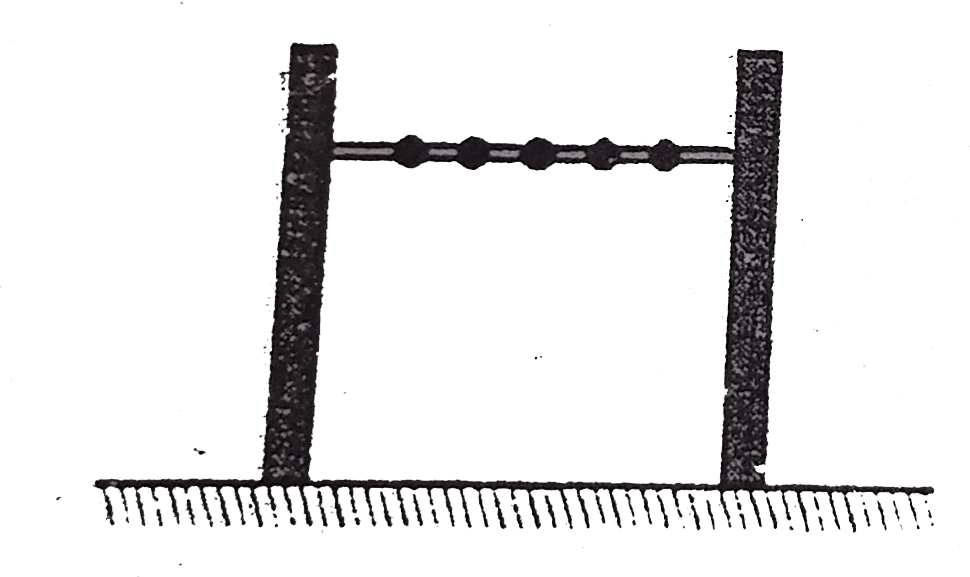 A5mv2L−5rBmv2L−10rC5mv2L−10rDmv2L−5r
A5mv2L−5rBmv2L−10rC5mv2L−10rDmv2L−5r - Question 2 - Select One
Three identical rods, each of mass m and length l, form an equaliteral triangle. Moment of inertia about one of the sides is
 Aml26Bml2C3ml24D2ml23
Aml26Bml2C3ml24D2ml23 - Question 3 - Select One
Two masses each of mass M are attached to the end of a rigid massless rod of length L. The moment of interia of the system about an axis passing centre of mass and perpendicular to its length is.
AML24BML22CML2D2ML2
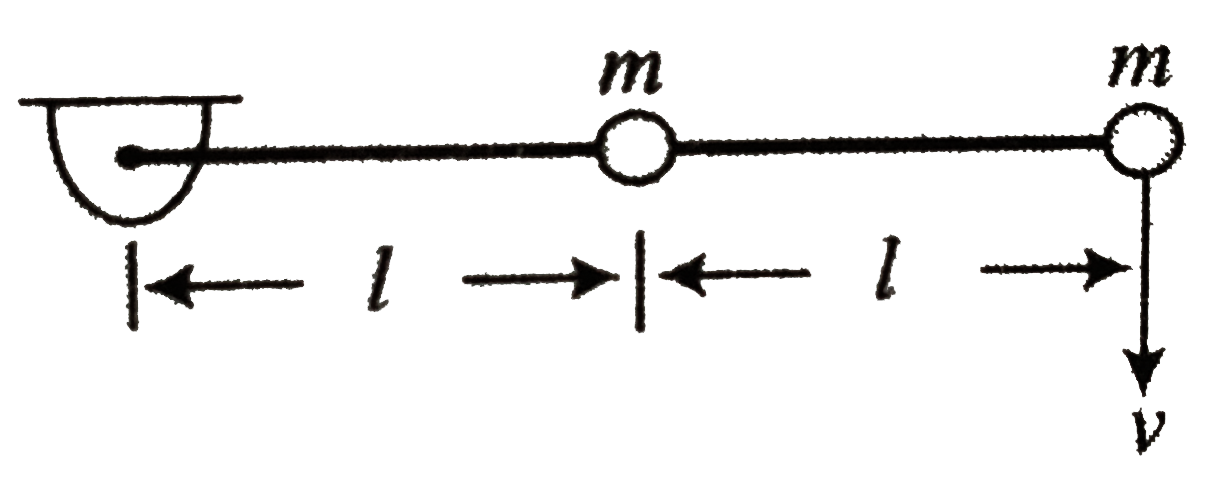
Two beads each of mass m are welded at the ends of two light rigid rods each of length l. if the pivots are smooth, find the ratio of translational and rotational kinetic energy of system.
 View Solution
View SolutionTwo identical beads each of mass 'm' can slide freely on a ring of mass M lying on smooth horizontal surface. The masses are brought to diametrically opposite positions and both are imparted with same tangential speed v in same direction. Find the kinetic energy of the ring when beads are about to collide.
View SolutionA metal bar of length L and area of cross-section A is clamped between two rigid supports. For the material of the rod. It Young's modulus is Y and Coefficient if linear expansion is α. If the temperature of the rod is increased by Δt∘C, the force exerted by the rod on the supports is
View SolutionA uniform rod of length L (in between the supports) and mass m is placed on two support A and B. The rod breaks suddenly at length L/10 from the support B. Find the reaction at support A immediately after the rod breaks
 View Solution
View SolutionA metal bar of length L and area of cross-section A is clamped between two rigid supports. For the material of the rod. It Young's modulus is T and Coefficient if linear expansion is α. If the temperature of the rod is increased by Δt∘C, the force exerted by the rod on the supports is
View Solution

