Two particles A and B oscillates in SHM having same amplitude and frequencies fA and fB respectively. At t=0 the particle A is at positive extreme position while the particle B is at a distance half of the amplitude on the positive side of the mean position and is moving away from the mean position.
The minimum time after which the two particles will be in same phase is 56(fA−fB) if fA>fB
The minimum time after which the two particles will be in same phase is 16(fA−fB) if fA>fB
The minimum time after which the two particles will be in same phase in 16(fB−fA) if fA<fB
The minimum time after which the two particles will be in same phase is 56(fB−fA) if fA<fB
The correct Answer is:A, C
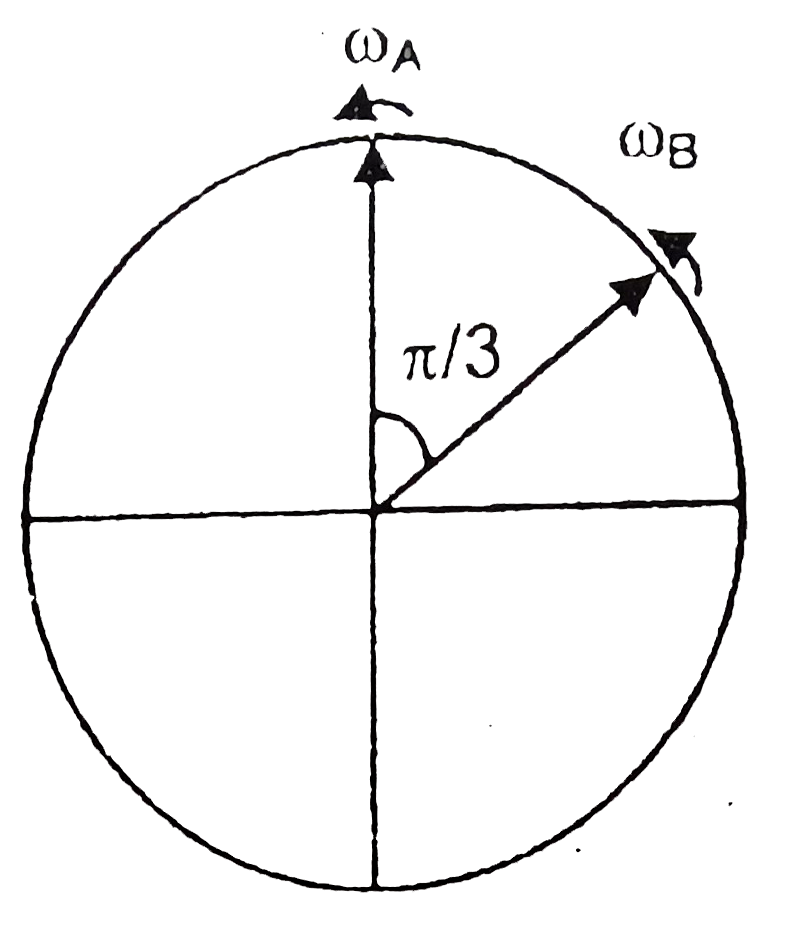
ωB>ωA⇒Δt=π/3ωB−ωA=π6(fB−fA)
ωB>ωA⇒Δt=2π−π3ωA−ωB=5π6(fA−fB)
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PT-01
30 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - Pt-02
30 Videos
- TEST PAPERSBOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS
107 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCECHAPTER - TEST SERIESEXERCISE - PHYSICS
127 Videos
Similar Questions
Knowledge Check
The phase of a particle performing S.H.M. when the particle is at a distance of amplitude from mean position is
Aπ/2BπC3π/2Dodd multiple of π/2Velocity at mean position of a particle executing SHM is v. Velocity of the particle at a distance equal to half of the amplitude will be
Av2Bv√2C√32vD√34 vParticle moves from extreme position to mean position, its
Akinetic energy increases, potential energy decreasesBkinetic energy decreases, potential increasesCboth remains constantDpotential energy becomes zero and kinetic energy remains contant
Velocity at mean position of a particle executing S.H.M. is v , they velocity of the particle at a distance equal to half of the amplitude
View SolutionWhen a particle executing a linear S.H.M. moves from its extreme position to the mean position, its
View SolutionA particle executes SHM with periodic time of 6 seconds. The time taken for traversing a distance of half the amplitude from mean position is :
View SolutionA particle executes SHM with a time period of 4 s . Find the time taken by the particle to go from its mean position to half of its amplitude . Assume motion of particle to start from mean position.
View SolutionThe total energy of a particle in SHM is E. Its kinetic energy at half the amplitude from mean position will be
View Solution