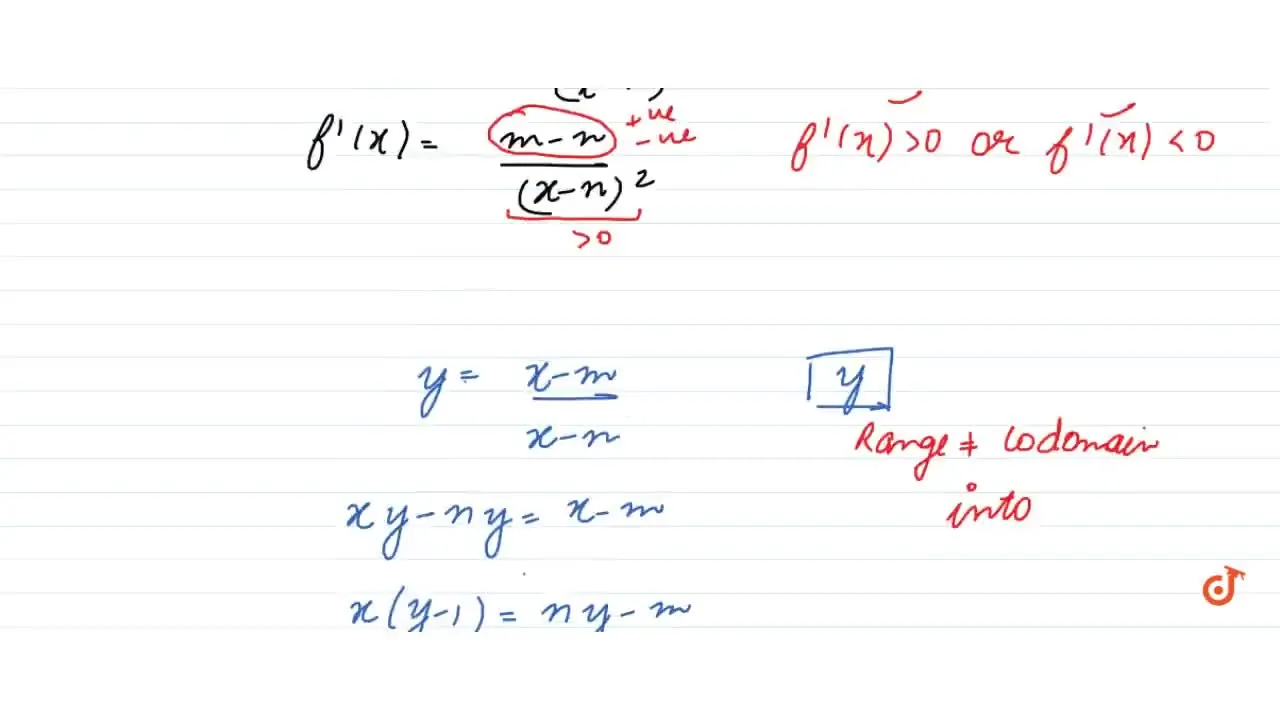
Let f:R−{n}→R be a function defined by f(x)=x−mx−n such that m≠n 1) f is one one into function2) f is one one onto function3) f is many one into funciton4) f is many one onto funcionn then
Correct option is B)
one−one test:
Let x1 and x2 be two elements in the domain
R−{n} such that
⇒f(x1)=f(x2)
⇒x1−mx1−m=x2−mx2−n
⇒(x1−m)(x2−n)=(x1−n)(x2−m)
⇒x1x2−nx1−mx2+mn=x1x2−mx1−nx2+mn
⇒(m−n)x1=(m−n)x2
⇒x1=x2
∴ f is one-one.
For onto :
Let y be an element in the co-domain R, such that
f(x)=y
⇒x−mx−mn=y
⇒x−m=xy−ny
⇒ny−m=xy−x
⇒ny−m=x(y−1)
⇒x=ny−my−1,
which is not defined for y=1
So, 1 has no pre-image in R−{n}
∴ f is not onto.
Related Playlists
Similar Questions
Let f:R→R be a function defined by f(x)=x2−8x2+2 . Then, f is (a) one-one but not onto (b) one-one and onto (c) onto but not one-one (d) neither one-one nor onto
View SolutionLet f:R→R be a function defined by f(x)=x2−3x+4x2+3x+4then f is (1) one-one but not onto (2) onto but not one -one (3) onto as well as one- one (4) neither onto nor one- one
View SolutionLet A=R−{2} and B=R−{1} if f:A→B is a function defined by f(x)=x−1x−2 show that f is one-one and onto. Hence find f−1.
View SolutionA function f:R→R be defined by f(x) = 5x + 6, prove that f is one-one and onto.
View SolutionShow that the function f(x)=3x+2 is one-one and onto
View SolutionA function f:R→R is defined by f(x)=4x3+5,x∈R.
Examine if f is one-one and onto.View SolutionLet f:(−π2,π2)→R be given by f(x)=(log(secx+tanx))3 then f(x) is an odd function f(x) is a one-one function f(x) is an onto function f(x) is an even function
View SolutionLet the function f:R−{−b}→R−{1} be defined by f(x)=x+ax+b , a≠b , then (a) f is one-one but not onto (b) f is onto but not one-one (c) f is both one-one and onto (d) none of these
View SolutionLet A=R−{3} and B=R−[1]. Consider the function f:A→B defined by f(x)=(x−2x−3). Show that f is one-one and onto and hence find f−1
View SolutionThe function f:x→Y defined by f(x)=x2−4x+5 is both one-one and onto if
View Solution