Force Exerted By A Light Beam On A Surface
Case I- a=1,r=0
Initial momentum of photon ( in downward direction at an angle θ with vertical)=hλ
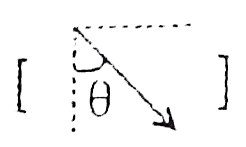
final momentum of photon =0
change in momentum ( in upward direction at an angle θ with vertical) =hλ
energy incident per unit time =IAcosθ
Intensity=power per unit normal area
I=PAcosθ,P=IAcosθ
No.of photons incident per unit time =IAcosθhc.λ
total change in momentum per unit time ( in upward direction at an angel θ with vertical)
−IAcosθλhc.hλ=IAcosθc
Force (F)= total change in momentum per unit time
F=IAcosθc ( direaction  on photon and
on photon and  on the plate)
on the plate)
Pressure=normal force per unit Area
Pressure=FcosθAPIAcos2θcA=Iccos2θ
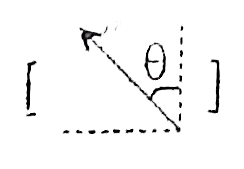
CaseII When r=1,a=0
∴ change in momentum of one photon
=2hλcosθ ( upward)

No.of photons incident per unit time
=energy incident per unit timehv
=IAcosθ.λhc
∴ total change in momentum per unit time
=IAcosθ.λhc×2hλcosθ=2IAcos2θc(upward)
∴ force on the plate=2IAcos2θc (downward)
Pressure =2IAcos2θcAP=2Icos2θc
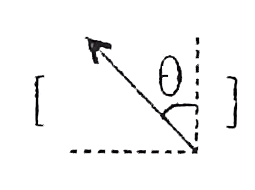
Case III 0<r<1,a+r=1
change in momentum of photon when it is reflected =2hλcosθ (downward)
change in momentum of photon when it is absorbed =hλ ( in the opposite direction of incident beam)
energy incident per unit time IAcosθ
no. of photns incident per unit time =IAcosθ.λhc
no. of reflected photon (nr)=IAcosθ.λhc
no.of reflected photon(nr)=IAcosθλhc(1−r)
force on plate due to absorbed photons Fa=na.ΔPa
=IAcosθλhc(1−r)hλ
=IAcosθc(1−r) ( at an angle θ with vertical) 
force on plate due to reflected photons Fr=nrΔPr
=IAcosθ.λhc×2hλcosθ(vertically downward)
=IAcos2θc.2r
now resultant force is given by FR=√(1−r)2+(2r)2cos2θ+4r(r−1)cos2θ
and pressure, P=Facosθ+FrA
=IAcosθ(1−r)cosθcA+IAcos2θ.2rcA
=Icos2θc(1−r)+Icos2θc=Icos2θc(1+r)
Topper's Solved these Questions
- ATOMIC PHYSICSBook:RESONANCEChapter:ATOMIC PHYSICSExercise:Solved miscellaneous problems
Explore 14 Videos
- ATOMIC PHYSICSBook:RESONANCEChapter:ATOMIC PHYSICSExercise:Exercise 1 Part-1 subjective questions
Explore 35 Videos
- ALTERNATING CURRENTBook:RESONANCEChapter:ALTERNATING CURRENTExercise:HIGH LEVEL PROBLEMS
Explore 11 Videos
- CAPACITANCEBook:RESONANCEChapter:CAPACITANCEExercise:High Level Problems
Explore 16 Videos
Similar Questions
A parallel beam of monochromatic light of wavelength 500nm is incident normally on a perfectly absorbing surface. The power through any cross- section of the beam is 10 W. Find (a) the number of photons absorbed per second by the surface and (b) the force exerted by the light beam on the surface.
View SolutionA parellel beam of monochromatic light of wavelength 500 nm is incident normally on a perfectly absorbing surface. The power through any cross section of the beam is 10 W. Find
(a) the number of photons absorbed per second by the surface and
(b) the force exerted by the light beam on the surface.
View Solution
Knowledge Check
Light of intensity 50W/m2 is incident on a arae of 1m2 in such a way that 25% of light is reflected back. Find the force exerted by light on surface if light incident perpendicularly
A10.8×10−8NB15.8×10−8NC20.8×10−8ND25.8×10−8N
A parallel beam of monochromatic light of frequency v is incident on a surface. Intensity of the beam is I and area of the surface is A. Find the force exerted by the light beam on the surface for following cases–
(i) the surface is perfectly absorbing and the light beam is incident normally on it.
(ii) the surface is perfectly reflecting and the light beam is incident normally.
(iii) the surface is perfectly absorbing and the light beam is incident at an angle of incidence θ.
(iv) the surface is perfectly reflecting and the light beam is incident at an angle of incidence θ.
View SolutionA parallel beam of white light is incident normally on surface which absorbs 70% of the incident light and reflects the rest. If incident beam has power of 40 W , then what will be the magnitude of force exerted by the light on this surface ?
View SolutionA plate of mass 10g is in equilibrium in air due to the force exerted by a light beam on the plate. Calculate power of the beam. Assume that the plate is perfectly absorbing.
View SolutionThere is a parallel beam of light of larger aperture . Let I be the intensity of light . Perfectly reflecting sphere of radius r is kept in the path of this parallel beam . Find the force exerted by the light beam on sphere .
View Solution(a) A parallel beam of monochromatic light of wavelength 500 nm is incident normally on a perfectly absorbing surface. The power through any cross section of the beam is 10 W. Find
(i) the number of photons absorbed per second by the surface and
the force exerted by the lilght beam on the surface.
(a) Radiation of wavelength 200 nm, propagating in the form of a parallel beam, fall normally on a plane metallic surface. The intensity of the beam is 5 mW and its cross-sectional area is 1.0mm2. Find the pressure exerted by the radiation on the metallic surface if the radiation is completeley reflected.
(c) A parallel beam of monochromatic light of wavelength 663 nm is incident on a totally reflecting plane mirror. The angle of incidence is 60∘ and the number of photons striking the mirror per second is 1.0×1019 . Calculate the force exerted by the light beam on the mirror.
(d) A beam of white light is incident normally on a plane surface absorbing 70%) of the light and reflecting the rest. If the incident beam carries 10 W of power, find the force exerted by it on the surface.
View SolutionLight with intensity 20 W / cm2 is falling along the normal on a surface , which completely abssorbs the light area of the surface is 25 cm2
find the average force exerted y light on this surface in one hour .
View SolutionA parallel beam of monochromatic light of wavelength 663 nm is incident on a totally reflecting plane mirror. The angle of incidence is 60∘ and the number of photons striking the mirror per second is (1.0×(1019)). Calculate the force exerted by the light beam on the mirror.
View Solution