A metal rod of length L is placed normal to a magnetic field and rotated through one end of rod in circular path with frequency f. The potential difference between it ends will be-
πL2Bf
BL/f
πL2B/f
fBL
The correct Answer is:A
To solve the problem of finding the potential difference (induced EMF) across the ends of a rotating metal rod in a magnetic field, we can follow these steps:
1. Understand the Setup:
We have a metal rod of length L that is placed perpendicular (normal) to a magnetic field B. The rod is rotated about one of its ends with a frequency f.
2. Identify the Relevant Formula:
The induced electromotive force (EMF) in a rotating rod in a magnetic field can be calculated using the formula:
E=12BωL2
where ω is the angular frequency in radians per second.
3. Convert Frequency to Angular Frequency:
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
4. Substitute ω into the EMF Formula:
Substitute ω into the EMF formula:
E=12B(2πf)L2
5. Simplify the Expression:
Simplifying the expression gives:
E=BπfL2
6. Final Result:
Therefore, the potential difference (induced EMF) between the ends of the rod is:
E=πBfL2
Conclusion:
The potential difference between the ends of the rod is given by the formula:
E=πBfL2
We have a metal rod of length L that is placed perpendicular (normal) to a magnetic field B. The rod is rotated about one of its ends with a frequency f.
The induced electromotive force (EMF) in a rotating rod in a magnetic field can be calculated using the formula:
E=12BωL2
where ω is the angular frequency in radians per second.
The angular frequency ω is related to the frequency f by the formula:
ω=2πf
Substitute ω into the EMF formula:
E=12B(2πf)L2
Simplifying the expression gives:
E=BπfL2
Therefore, the potential difference (induced EMF) between the ends of the rod is:
E=πBfL2
The potential difference between the ends of the rod is given by the formula:
E=πBfL2
Topper's Solved these Questions
Similar Questions
A conducing rod of length l is falling with a velocity v perpendicular to a unifrorm horizontal magnetic field B . The potential difference between its two ends will be
View SolutionA metal rod of length 2 m is rotating with an angualr velocity of 100rads−1 in plane perpendicular to a uniform magnetic field of 0.3 T. The potential difference between the ends of the rod is
View Solution
Knowledge Check
A metal rod of length L is placed normal to a magnetic field and rotated through one end of rod in circular path with frequency f. The potential difference between it ends will be-
AπL2BfBBL/fCπL2B/fDfBLA conducting rod of length l is hinged at point O. It is a free to rotate in a verical plane. There exists a uniform magnetic field B in horizontal direction. The rod is released from the position shown. The potential difference between the two ends of the rod is proportional to
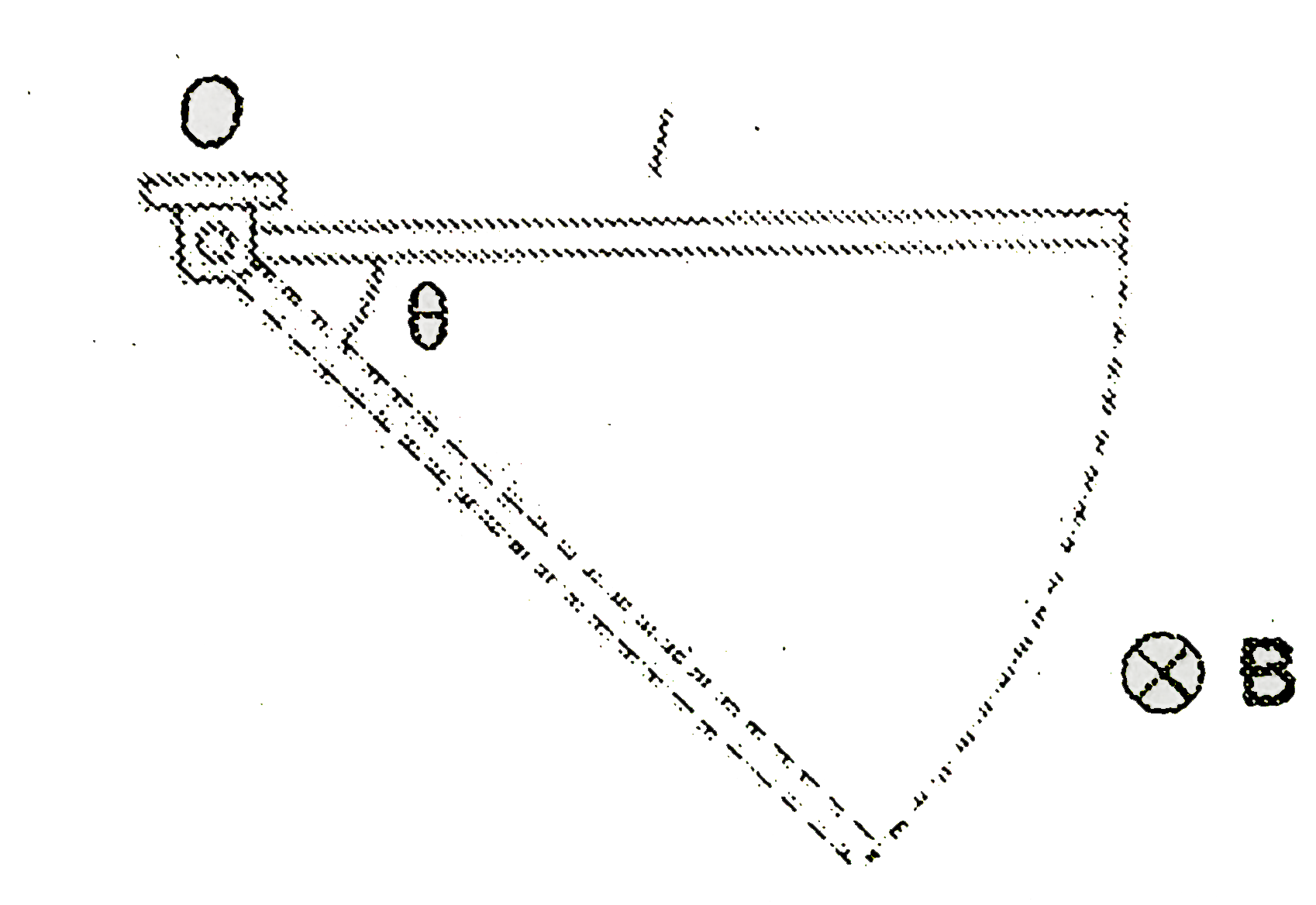 Al3/2Bl2CsinθD(sinθ)1/2
Al3/2Bl2CsinθD(sinθ)1/2A rod of length 20 cm is rotating with angular speed of 100 rps in a magnetic field of strength 0.5 T about it’s one end . What is the potential difference betweet two ends of the rod :
A2.28VB4.28VC6.28VD2.5V
A metal rod of length 2m is rotating with an angular velocity of 100rad/sec in a plane perpendicular to a uniform magnetic field of 0.3T. The potential difference between the ends of the rod is
View SolutionA conducting rod of length l is falling with a velocity v perpendicular to a uniform horizontal magnetic field B. The potential difference between the two ends will be
View SolutionA metallic rod of length L rotates at an angular speed ω, normal to a uniform magnetic field. If the resitance of rod is R the heat dissipated is:
View SolutionA mental rod AB of length l is rotated with a constant angular velocity ω about an axis passing through O and normal to its length . Potenial difference between ends of rod in absence of external magnetic field ( Where , e = electric change)
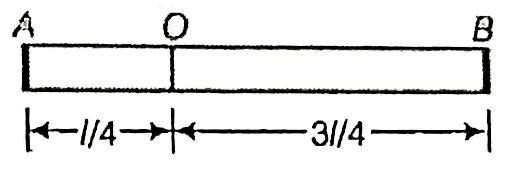
View SolutionA conducting rod of length l is hinged at point O. It is a free to rotate in a verical plane. There exists a uniform magnetic field B in horizontal direction. The rod is released from the position shown. The potential difference between the two ends of the rod is proportional to

View Solution
