A parallel plate capacitor of area A and separation d is charged to potential difference V and removed from the changing source. A dielectric slab of constant K =2, thickness d and are area A2 is inserted, as shown in the figure. Let σ1 be free charge density at the conductor-dielectric surface and σ2 be the charge density at the conductor-vacuum surface
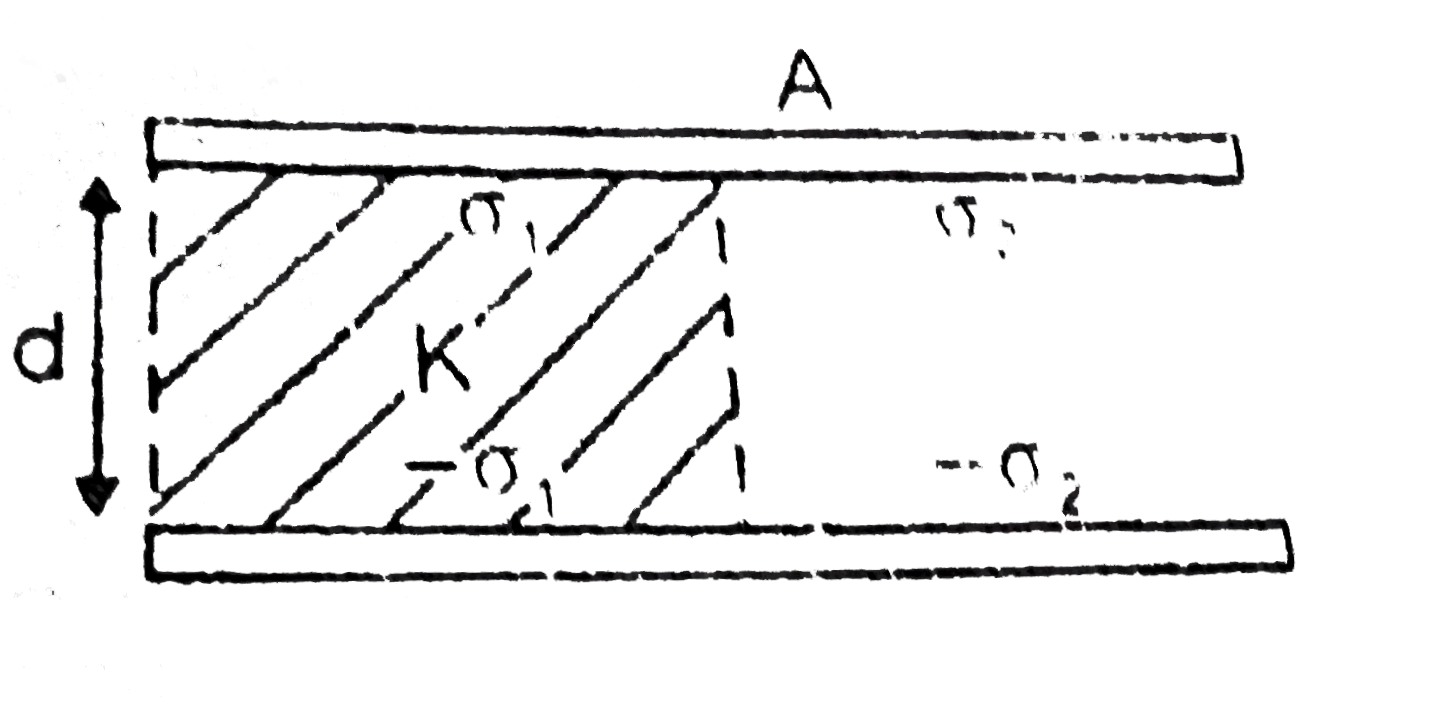
The electric field have same value inside the dielectric as in the free space between the plates
The ratio σ1σ2 is equal to 21
The new capcitance is 3∈0A2d
The new potential difference is 23V
The correct Answer is:A, B, C, D
Potential for each plate remain same over whole area. If potential difference between them is, say V' then V' = Ed
i.e. E is also same inside the plates to keep E same, free charge density is changed i.e charge redistributes itself
To find new capacitance two capacitors can be taken as connected in parallel. Then
−K.∈0A/2d+∈0A/2d=3∈0A2d
By Q=CV, as Q remains unchanged V is changed to 23V.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.55
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.56
20 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.53
20 Videos
- CURRENT ELECTRICITYBOOK - RESONANCECHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
21 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCECHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
A parallel plate capacitor having plate area A and separation between the plates d is charged to potential V.The energy stored in capacitor is ....???
View SolutionA parallel - plate capacitor of plate area Aand plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View Solution
Knowledge Check
A parallel plate capacitor is charged to a potential difference of 100V and disconnected from the source of emf. A slab of dielectric is then inserted between the plates. Which of the following three quantities change?
Aonly (i) and (ii)Bonly (i) and (iii)Conly (ii) and (iii)DAll (i),(ii) and (iii)A parallel plate capacitor of plate area A and plate separation d is charged to potential difference V and then the battery is disconnected. A slab of dielectric constant K is then inserted between the plates of the capacitor so as to fill the space between the plates. If Q, E and W denote respectively, the magnitude of charge on each plate, the electric field between the plates (after the slab is inserted), and work done on the system, in question, in the process of inserting the slab, then
A(a) Q=ε0AVdB(b) Q=ε0KAVdC(c) E=VKdD(d) W=ε0AV22d[1−1K]A parallel plate capacitor has plates with area A and separation d. A battery charges the plates to a potential difference V0. The battery is then disconnected and a dielectric slab of thikness d is introduced. The ratio of the enrgy stored in the capacitor before and after the slab is introduced, is
AKB1KCAd2KDd2KA
A parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
charge on plates ?
Justify your answer
View SolutionA parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
capacitance of the capacitor ? Justify your answer .
View SolutionA parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
electric filed intensity between the plates ? Justify your answer .
View SolutionA parallel plate capacitor each with plate area A and separation ‘d’ is charged to a potential difference V. The battery used to charge it is then disconnected. A dielectric slab of thickness d and dielectric constant K is now placed between the plates. What change if any, will take place in
(i) charge on the plates
(ii) electric field intensity between the plates,
(iii) capacitance of the capacitor
Justify your answer in each case
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dielectric constant K is placed in between the plates of the capacitor as shown.
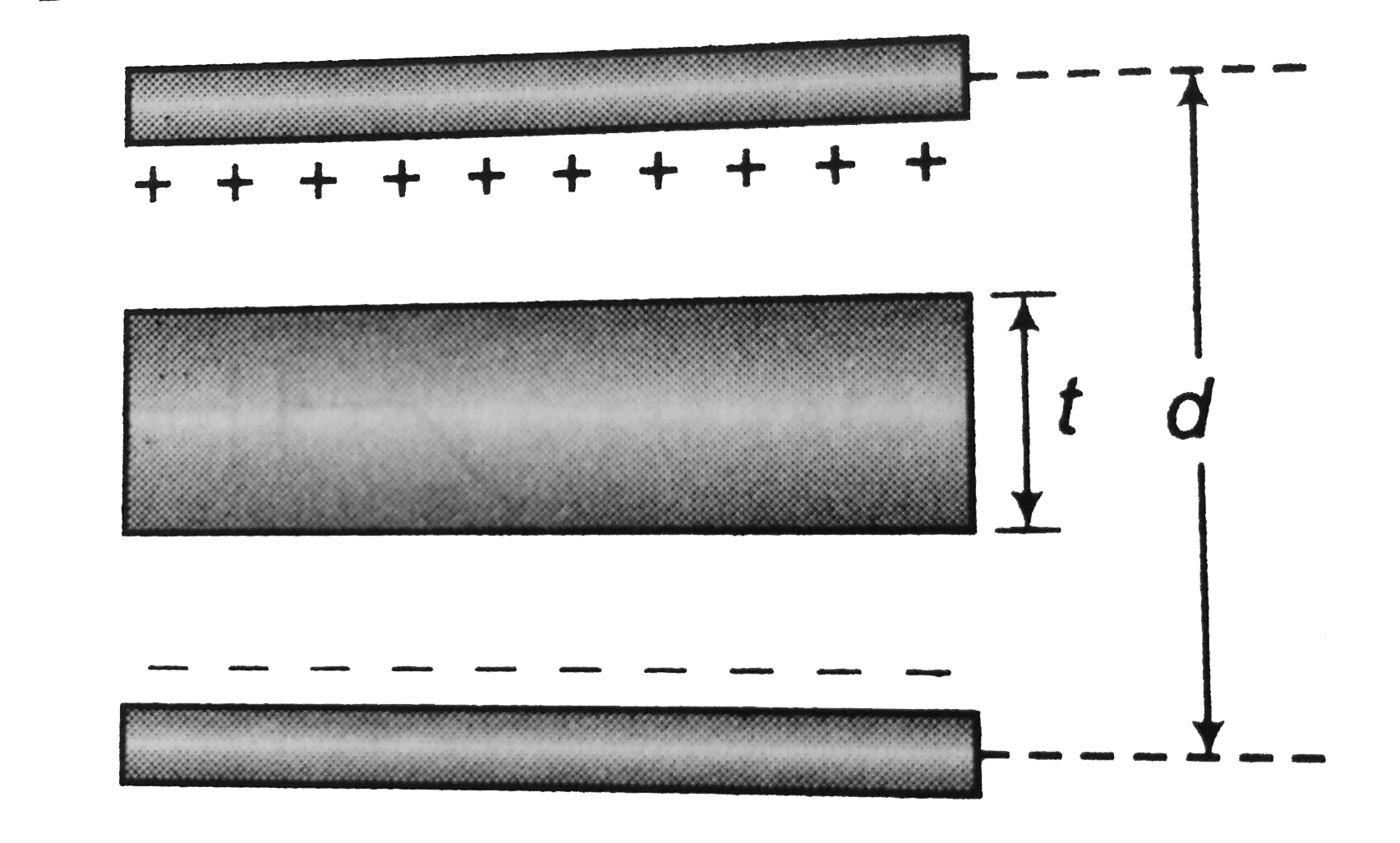
The electric field in the dielectric slab is
View Solution
