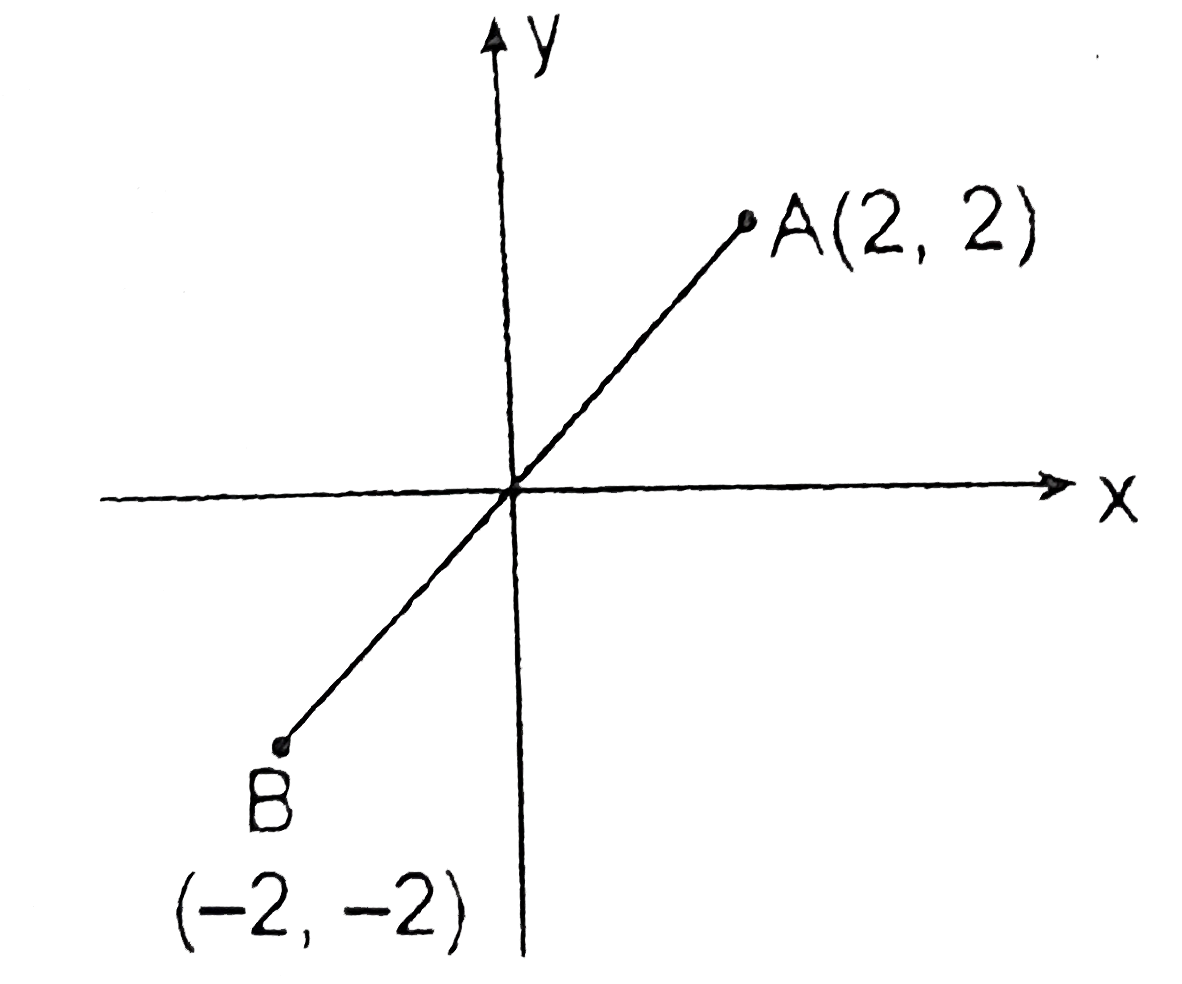
A particle of mass $m = 2 k g$ executes $S H M$ in $x y$ - plane between point A and B under action of force $\to F = F_{x} ˆ i + F_{y} ˆ j$ . Minimum time taken by particle to move from A to B is 1 sec. At $t = 0$ the particle is at $x = 2$ and $y = 2$ . Then $F_{x}$ as function of time t is

$- 4 π^{2} sin π t$

$- 4 π^{2} cos π t$

$4 π^{2} cos π t$

None of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

Updated on:21/07/2023

A particle of mass $2 k g$ moves in the $x y$ plane under the action of a constant force $\to F$ where $\to F = ˆ i - ˆ j$ . Initially the velocity of the particle is $2 ˆ j$ . The velocity of the particle at time $t$ is
A $\frac{1}{2} (t + 4) ˆ i - \frac{1}{2} t ˆ j$
B $t (ˆ i - ˆ j)$
C $\frac{1}{2} t (ˆ i - ˆ j)$
D $\frac{1}{2} t ˆ i + \frac{1}{2} (t + 4) ˆ j$
Particle moves from point A to point B along the line shown in figure under the action of force. $e v c F = - x ˆ i + y ˆ j$ . Determine the work done on the particle by $\to F$ in moving the particle from point A to point B.
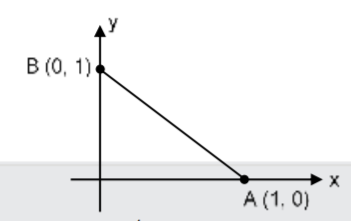A1 J
B1/2 J
C2 J
D3 J
A particle of mass m moves from A to C under the action of force $\to F = 2 x y ˆ i + y^{2} ˆ j$ , along different paths as shown in figure.

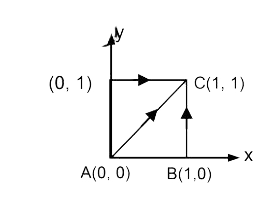AForce is conservative in nature
BWork done by force is $\frac{1}{3}$ when particle moves along path ABC
CForce is non conservative in nature and work done along path AC is 1 J
DWork done along path ADC is $\frac{14}{5} J$