A particle is placed at the origin of the coordinate system. Two forces of magnitude 20N&10N act on it as shown in figure. It is found that it starts moving towards the point (1,1) . The net unknown force acting on the particle at this position can be :
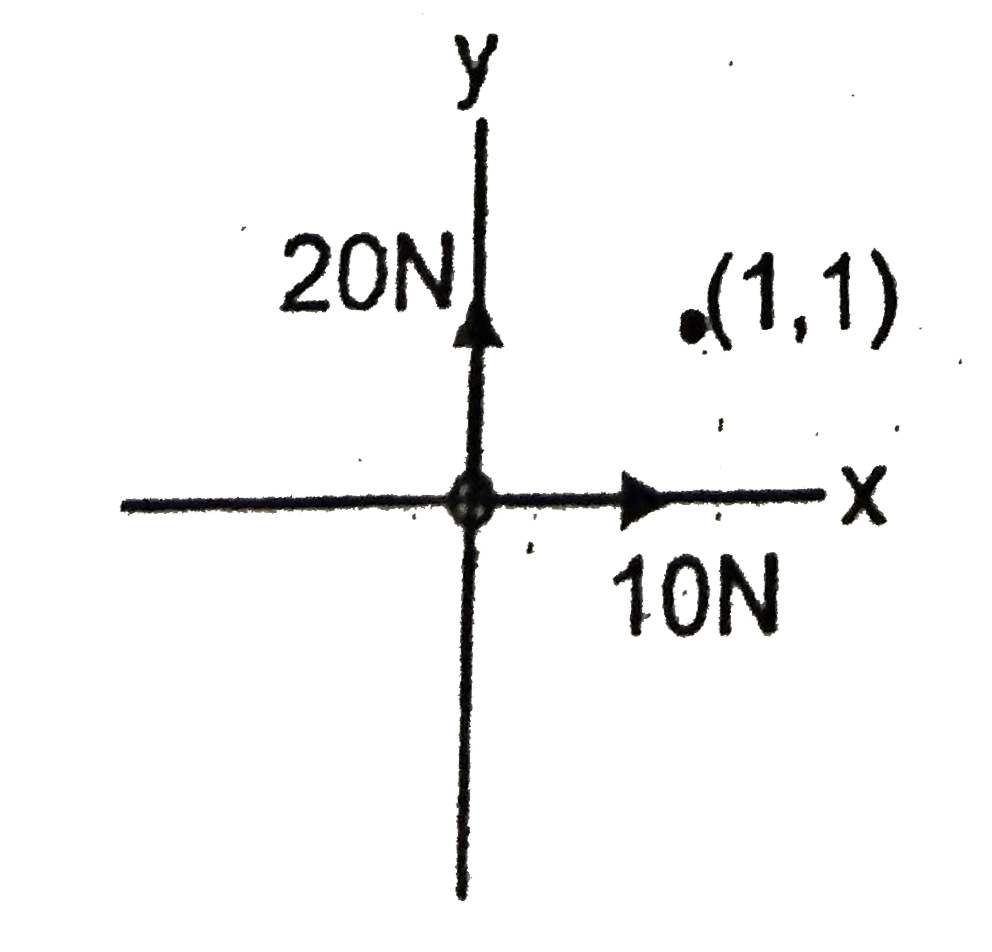
A particle is placed at the origin of the coordinate system. Two forces of magnitude 20N & 10N act on it as shown in figure. It is found that it starts moving towards the point (1,1) . The net unknown force acting on the particle at this position can be :

A

15√2 at angles 45∘ with positive x axis

B

5√2 at angle 135∘ with positive x axis

C

5√2 at angle −45∘ with positive x axis

D

None of these

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:C

Check the options so that the resultant force comes towards (1,1).i.e.,Fxnet=Fynet
( There exist inifnite solutions because the acceleratio is not given, for example
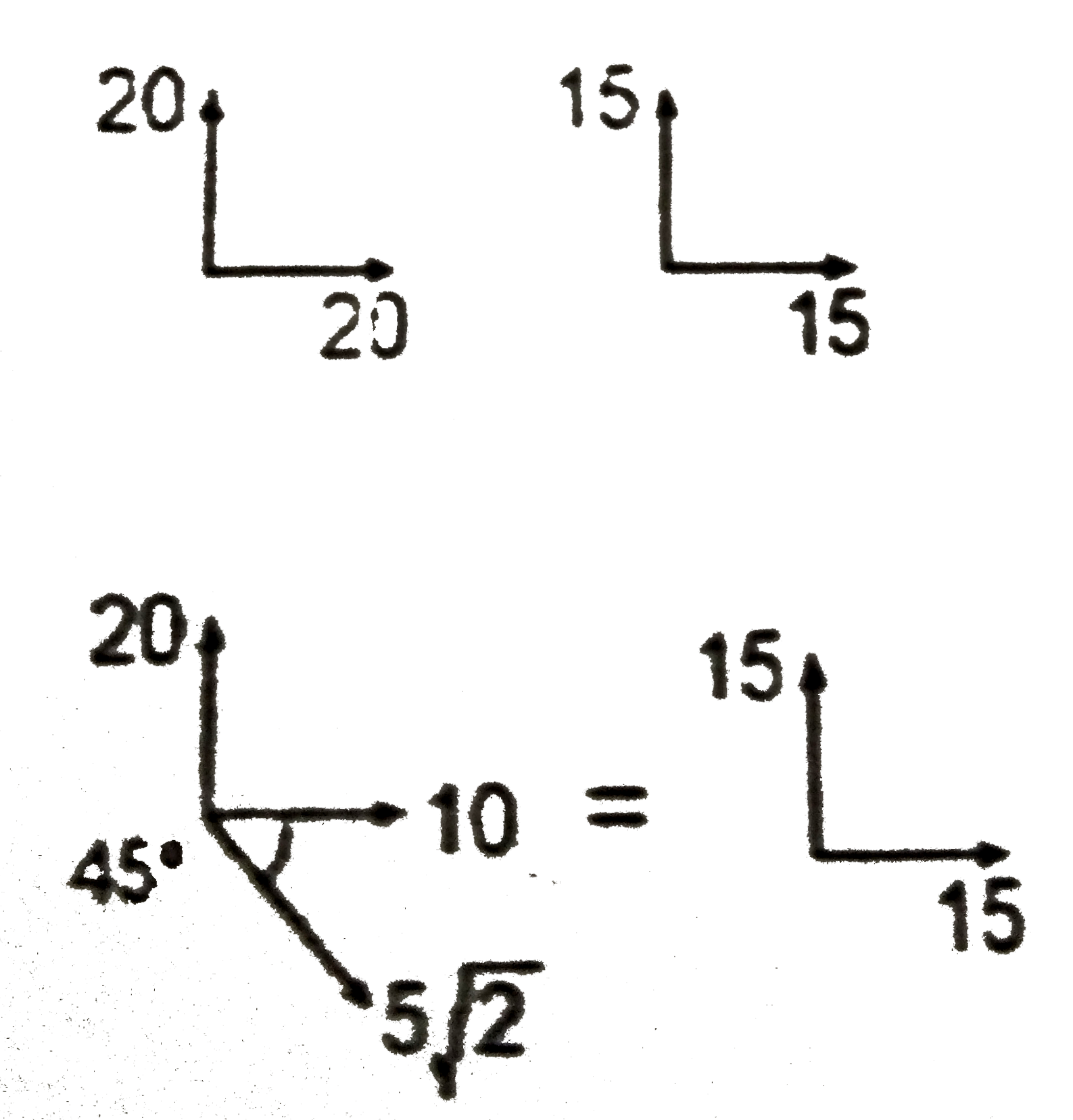
ocr_image

Updated on:21/07/2023
|
Class 11PHYSICSQUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A force of −4FˆK acts O, the origin of the coordinate system. The torque about the point (1,−1) is

    A−4F(ˆi−ˆj)
    B4F(ˆi−ˆj)
    C−4F(ˆi+ˆj)
    D4F(ˆi+ˆj)

  • A force of −F,ˆk acts on O, the origin of the coordinate system. The torque about the point (1,-1) is
    ocr_image

    AF(ˆi−ˆj)
    B−F(ˆi+ˆj)
    CF(ˆi+ˆj)
    D−F(ˆi−ˆj)

  • A force of −F,ˆk acts on O, the origin of the coordinate system. The torque about the point (1,-1) is
    ocr_image

    AF(ˆi−ˆj)
    B−F(ˆi+ˆj)
    CF(ˆi+ˆj)
    D−F(ˆi−ˆj)