An automobile enters a turn of radius $R$ . If the road is banked at an angle of $45^{\circ}$ and the coefficient of friction is 1, the minimum and maximum speed with which the automobile can negotiate the turn without skidding is $:$

A

$\sqrt{\frac{r g}{2}} and \sqrt{r g}$

B

$\frac{\sqrt{r g}}{2} and \sqrt{r g}$

C

$\frac{\sqrt{r g}}{2} and 2 \sqrt{r g}$

D

0 and inifinite

Video Solution

Text Solution

Verified by Experts

The correct Answer is:D

$F . B . D .$ for minimum speed $(w . r . t$ automobile $) :$

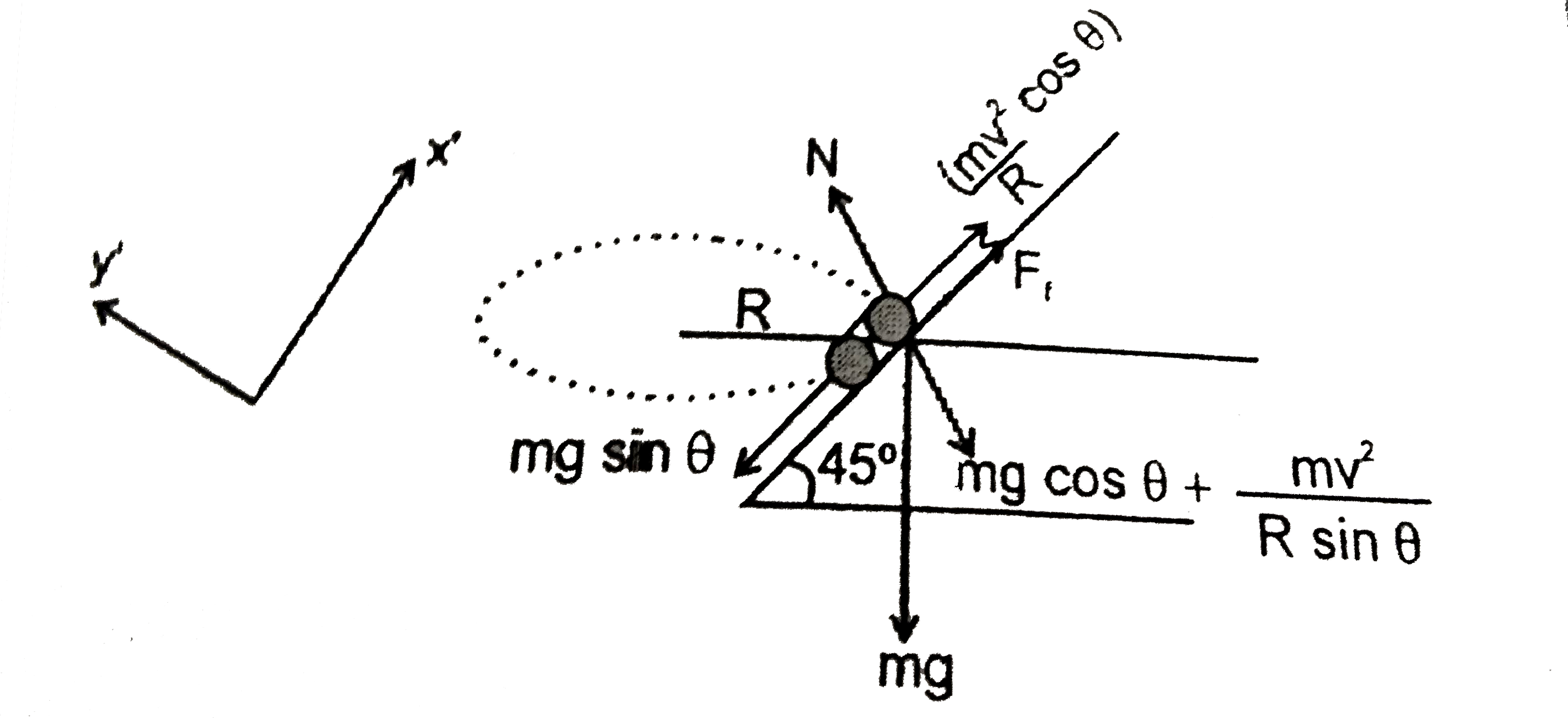
$Σ f_{y} = N - m g cos θ - \frac{m v^{2}}{R} sin θ = 0$
$Σ f_{x} = \frac{m v^{2}}{R} cos θ + μ N - m g sin θ = 0$
$\Rightarrow \frac{m v^{2}}{R} cos θ + μ (m g cos θ + \frac{m v^{2}}{R} sin θ) - m g sin θ = 0$
$\Rightarrow v^{2} = \frac{(μ R g cos θ - R g sin θ)}{(cos θ + μ sin g θ)}$
for $θ = 45^{\circ}$ and $μ = 1 :$
$v_{min} = \frac{R g - R g}{1 + 1} = 0$
$F . B . D$ for maximum speed $(w . r . t .$ automobile)

$Σ f_{x} = \frac{m v^{2}}{R} cos θ - m g sin θ - μ (m g cos θ + \frac{m v^{2}}{R} sin θ) = 0$
for $θ 45^{\circ}$ and $μ = 1$
$v_{max} = \infty ($ infinite $)$

|

Updated on:21/07/2023

Class 11 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A cyclist moves in a circular track of radius 100 m. If the coefficient of friction is 0.2 , then the maximum velocity with which the cyclist can take the turn with leaning inward is
A $140 m / s$
B $14 m / s$
C $1.4 m / s$
D $4.9 m / s$
Question 2 - Select One
A car is negotiating a curved road of radius R. The road is banked at an angle $θ$ . The coefficient of friction between the tyres of the car and the road is $μ_{s}$ . The maximum safe velocity on this road is
A $\sqrt{\frac{g}{R} \frac{(μ_{s} + tan θ)}{R (1 - μ_{s} tan θ)}}$
B $\sqrt{\frac{g}{R^{2}} \frac{(μ_{s} + tan θ)}{(1 - μ_{s} tan θ)}}$
C $g R^{2} \frac{(μ_{s} + tan θ)}{(1 - μ_{s} tan θ)}$
D $\sqrt{g R \frac{(μ_{s} + tan θ)}{(1 - μ_{s} tan θ)}}$
Question 3 - Select One
A car turns a corner on a slippery road at a constant speed of $10 m / s$ . If the coefficient of friction is 0.5, the minimum radius of the arc in meter in which the car turns is
A20
B10
C5
D4

RESONANCE-DAILY PRACTICE PROBLEMS-dpp 44

Home
Ask Doubt
Profile