Function f : R→R, f(x) =x+|x|, is
One-one
Onto
One-one onto
Many one
The correct Answer is:D
To determine the nature of the function f(x)=x+|x|, we will analyze it step by step.
Step 1: Understand the function definition
The function is defined as:
f(x)=x+|x|
The absolute value function |x| behaves differently depending on whether x is positive or negative.
Step 2: Break down the function based on the sign of x
1. Case 1: x≥0
- Here, |x|=x
- Thus, f(x)=x+x=2x
2. Case 2: x<0
- Here, |x|=−x
- Thus, f(x)=x−x=0
Step 3: Write the piecewise function
From the above cases, we can express f(x) as a piecewise function:
f(x)={2xif x≥00if x<0
Step 4: Analyze the function for injectivity (one-one)
To check if the function is one-one, we can look at the outputs for different inputs:
- For x≥0: The function f(x)=2x is clearly one-one since it is a linear function with a positive slope.
- For x<0: The function outputs f(x)=0 for all negative x.
Since multiple values of x<0 yield the same output f(x)=0, the function is not one-one.
Step 5: Analyze the function for surjectivity (onto)
To check if the function is onto, we need to compare the range of f(x) with its codomain (which is R):
- The range of f(x) is:
- For x≥0, f(x) takes values from 0 to +∞ (since 2x can take any non-negative value).
- For x<0, f(x)=0.
Thus, the range of f(x) is (0,+∞).
Since the codomain is R (which includes negative values), and the range does not cover all real numbers, the function is not onto.
Conclusion
Based on the analysis:
- The function is not one-one (many values of x<0 map to the same output).
- The function is not onto (the range does not cover all real numbers).
Therefore, we conclude that the function f(x)=x+|x| is a many-one function.
The function is defined as:
f(x)=x+|x|
The absolute value function |x| behaves differently depending on whether x is positive or negative.
1. Case 1: x≥0
- Here, |x|=x
- Thus, f(x)=x+x=2x
- Here, |x|=−x
- Thus, f(x)=x−x=0
From the above cases, we can express f(x) as a piecewise function:
f(x)={2xif x≥00if x<0
To check if the function is one-one, we can look at the outputs for different inputs:
- For x≥0: The function f(x)=2x is clearly one-one since it is a linear function with a positive slope.
- For x<0: The function outputs f(x)=0 for all negative x.
To check if the function is onto, we need to compare the range of f(x) with its codomain (which is R):
- The range of f(x) is:
- For x≥0, f(x) takes values from 0 to +∞ (since 2x can take any non-negative value).
- For x<0, f(x)=0.
Based on the analysis:
- The function is not one-one (many values of x<0 map to the same output).
- The function is not onto (the range does not cover all real numbers).
Topper's Solved these Questions
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - B) Objective Type Questions (one option is correct)
87 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - C) Objective Type Questions (More than one option are correct)
17 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTECHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Try Yourself
70 Videos
- PROBABILITYBOOK - AAKASH INSTITUTECHAPTER - PROBABILITYEXERCISE - ASSIGNMENT SECTION-J (aakash challengers questions)
13 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTECHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers
12 Videos
Similar Questions
Knowledge Check
If function f:R→R+,f(x)=2x, then f−1 (x) will be equal to
Alogx2Blog2(1/x)Clog2xDxThe function f:R→R,f(x)=x2 is
Ainjective but not surjectiveBsurjective but not injectiveCinjective as well as surjectiveDneither injective nor surjective.The function f:R→R defined by f(x)=x2+x is
Aone-oneBontoCmany-oneDNone of these
Define the function f:R→R by y=f(x)=x5,x∈R. What is the domain and range of this function. Draw the graph of f.
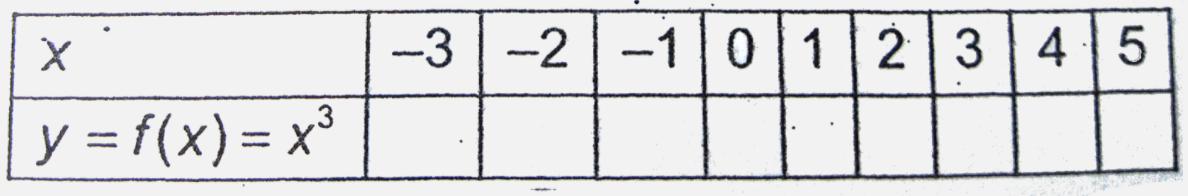
View SolutionDefine the function f:R→R by y=f(x)=x3,x∈R. Complete the table given below by using this definition. What is the domain and range of this function ? Draw the graph of f.
View SolutionIf f:R→R, then f(x)=|x| is
View SolutionIf R is the set of real numbers prove that a function f:R→R,f(x)=ex,x∈R is one to one mapping.
View SolutionFunction is said to be onto if range is same as co - domain otherwise it is into. Function is said to be one - one if for all x1≠x2⇒f(x1)≠f(x2) otherwise it is many one.
Function f:R→R,f(x)=x2+x, is
View Solution
