The function f:R→R,f(x)=x2 is
injective but not surjective
surjective but not injective
injective as well as surjective
neither injective nor surjective.
The correct Answer is:D
To determine the properties of the function f:R→R defined by f(x)=x2, we need to check if it is injective (one-to-one) and/or surjective (onto).
Step 1: Check if the function is injective
A function is injective if different inputs map to different outputs. In other words, if f(a)=f(b) implies that a=b.
Let’s assume f(a)=f(b):
a2=b2
This implies:
a=bora=−b
Since a can be equal to b or the negative of b, we can find different inputs that yield the same output (for example, f(1)=1 and f(−1)=1). Therefore, the function is not injective.
Step 2: Check if the function is surjective
A function is surjective if for every element y in the codomain R, there exists at least one x in the domain R such that f(x)=y.
Let’s consider y=−4:
f(x)=−4⟹x2=−4
This equation has no real solutions since the square of a real number cannot be negative. Therefore, there are values in R (like -4) that are not achieved by f(x). Thus, the function is not surjective.
Conclusion
Since the function f(x)=x2 is neither injective nor surjective, the final answer is that the function is neither injective nor surjective.
Final Answer
The function f(x)=x2 is neither injective nor surjective.
A function is injective if different inputs map to different outputs. In other words, if f(a)=f(b) implies that a=b.
a2=b2
This implies:
a=bora=−b
Since a can be equal to b or the negative of b, we can find different inputs that yield the same output (for example, f(1)=1 and f(−1)=1). Therefore, the function is not injective.
A function is surjective if for every element y in the codomain R, there exists at least one x in the domain R such that f(x)=y.
f(x)=−4⟹x2=−4
This equation has no real solutions since the square of a real number cannot be negative. Therefore, there are values in R (like -4) that are not achieved by f(x). Thus, the function is not surjective.
Since the function f(x)=x2 is neither injective nor surjective, the final answer is that the function is neither injective nor surjective.
The function f(x)=x2 is neither injective nor surjective.
Topper's Solved these Questions
- TIPSBOOK - FIITJEECHAPTER - TIPSEXERCISE - ASSERTION REASONING
8 Videos
- TIPSBOOK - FIITJEECHAPTER - TIPSEXERCISE - MCQ (MULTIPLE CORRECT)
37 Videos
- TIPSBOOK - FIITJEECHAPTER - TIPSEXERCISE - ASSIGNMENT (SECTION (I): MCQ (SINGLE CORRECT)
125 Videos
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - MATHEMATICS
328 Videos
- TRIGNOMETRIC RATIOS AND IDENTITIESBOOK - FIITJEECHAPTER - TRIGNOMETRIC RATIOS AND IDENTITIESEXERCISE - All Questions
1 Videos
Similar Questions
The function f:R→R,f(x)=ax2−b|x|,a,b∈R has a local minimum at x=0 then which of the following options must be CORRECT?
O a>0
O b<0
O |ab|+a|b|+b|a|=−ab
O |ab|+a|b|=b|a|+ab
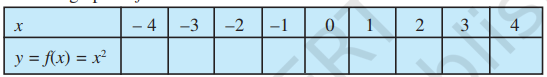
View SolutionDefine the function f : R →R by y = f(x) = x2, x∈R. Complete the Table given below by using this definition. What is the domain and range of this function?
View Solution
Knowledge Check
Function is said to be onto if range is same as co - domain otherwise it is into. Function is said to be one - one if for all x1≠x2⇒f(x1)≠f(x2) otherwise it is many one.
Function f:R→R,f(x)=x2+x, isAone - one ontoBone - one intoCmany one ontoDmany one intoconsider the function f:R→R,f(x)=x2−6x+4x2+2x+4
f(x) isAunbounded functionBone one functionConto functionDnone of theseconsider the function f:R→R,f(x)=x2−6x+4x2+2x+4
Range of fX() isA(−∞−23]∪[2,0)B[−13,5]C(−∞,2)∪[73,∞)D(20,∞)
Function f:R→R,f(x)=x|x| is
View SolutionThe function f:R→R satisfies f(x2).fx=f′(x).f′(x2) for all real x. Given that f(1)=1 and f1=8 , then the value of f′(1)+f1 is 2 b. 4 c. 6 d. 8
View SolutionIf f(x)=1x2+x+1, then the function f:R→R is
View Solutionconsider the function f:R→R,f(x)=x2−6x+4x2+2x+4
which of the following is not true about f(x)?
View SolutionThe function f:R→R defined as f(x)=x2−x+1x2+x+1 is
View Solution
