In a triangle ABC
sinA. sinB.sinC =Δ2R2
sinA.sinB.sinC =r2R(sinA+sinB+sinC)
acosA+bcosB+ccosC=abc2R2
sinA.sinB.sinC=R2r(sinA+sinB+sinC)
The correct Answer is:A, B
Step by step video, text & image solution for In a triangle ABC by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TRIGNOMETRIC FUNCTIONS Book:AAKASH INSTITUTEChapter:TRIGNOMETRIC FUNCTIONS Exercise:Section D (Linked Comprehension Type Questions)
Explore 24 Videos
- TRIGNOMETRIC FUNCTIONS Book:AAKASH INSTITUTEChapter:TRIGNOMETRIC FUNCTIONS Exercise:Section E (Assertion-Reason Types Questions)
Explore 18 Videos
- TRIGNOMETRIC FUNCTIONS Book:AAKASH INSTITUTEChapter:TRIGNOMETRIC FUNCTIONS Exercise:Section-B (Objective Type Questions One option is correct)
Explore 131 Videos
- THREE DIMENSIONAL GEOMETRY Book:AAKASH INSTITUTEChapter:THREE DIMENSIONAL GEOMETRY Exercise:ASSIGNMENT SECTION - J
Explore 10 Videos
- VECTOR ALGEBRABook:AAKASH INSTITUTEChapter:VECTOR ALGEBRAExercise:SECTION-J (Aakash Challengers Questions)
Explore 5 Videos
Similar Questions
Knowledge Check
There exists triangle ABC satisfying
AtanA+tanB+tanC=0BsinA2=sinB3=sinC7C(a+b)2=c2+aband√2(sinA+cosA)=√3DsinA+sinB=√3+12,cosAcosB=√34=sinAsinBIn △ABC,∠A=∠C,AC=5 and BC = 4. Then, the perimeter of △ ABC is ..........
A9B14C13D15In △ABC, AB=AC and AD⊥BC,BE⊥AC and CF⊥AB. Then △ABC is symmetrical about
AADBBECCFDNone of these
The centroid of a triangle ABC is G. The area of triangle ABC is 60 cm2. The area of triangle GBC is.
View SolutionBE and CF are two equal altitudes of a triangle ABC. Using RHS congruence rule, prove that the triangle ABC is isosceles.
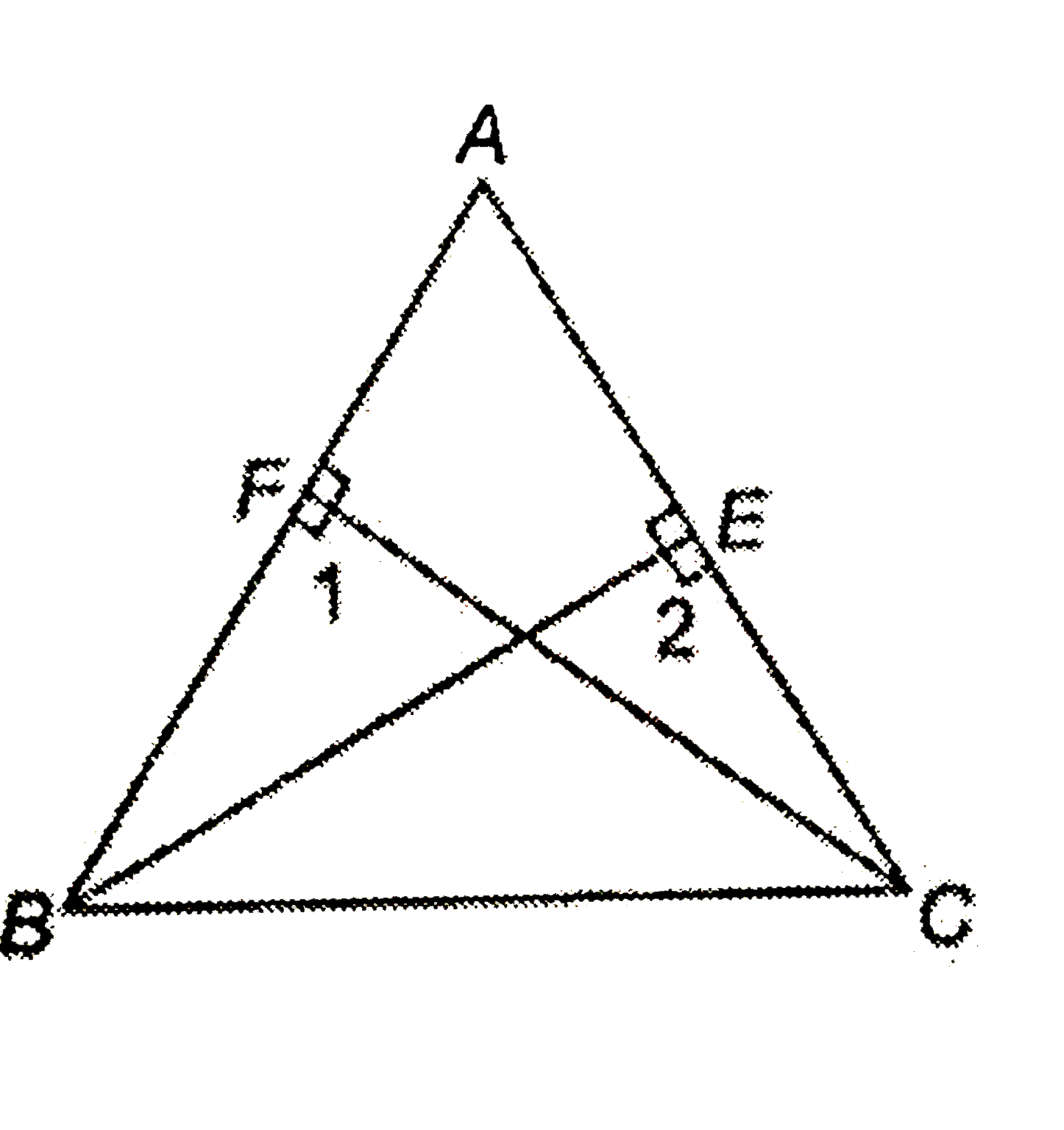
View SolutionThe mid points of sides of a triangle ABC are (2,1),(3,-1) and (0,0) .The area of triangle ABC is
View SolutionIn any triangle ABC, if the angle bisector of ∠Aand perpendicular bisector of BCintersect, prove that they intersect on the circumcircle of the triangle ABC
View SolutionIf I is the incentre of △ABC and ∠BIC=135∘, then △ABC is
यदि I त्रिभुज △ABC का अंतः केंद्र है और ∠BIC=135∘ तो △ABC है-
View Solution