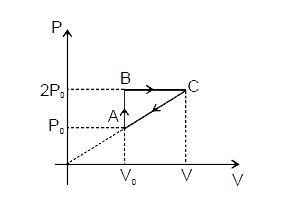
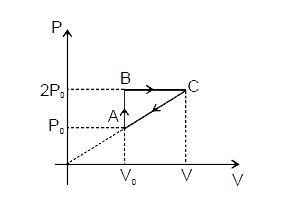
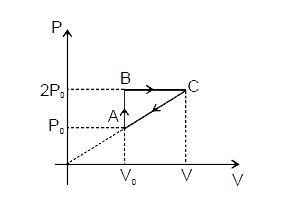
n moles of a diatomic gas has undergone a cyclic process ABC as shown in figure. Temperature at A is T0. Find
a. Volume at C ?
b. Maximum temperature ?
c. Total heat given to gas ?
d. Is heat rejected by the gas, if yes how much heat is rejected ?
e. Find out the efficiency
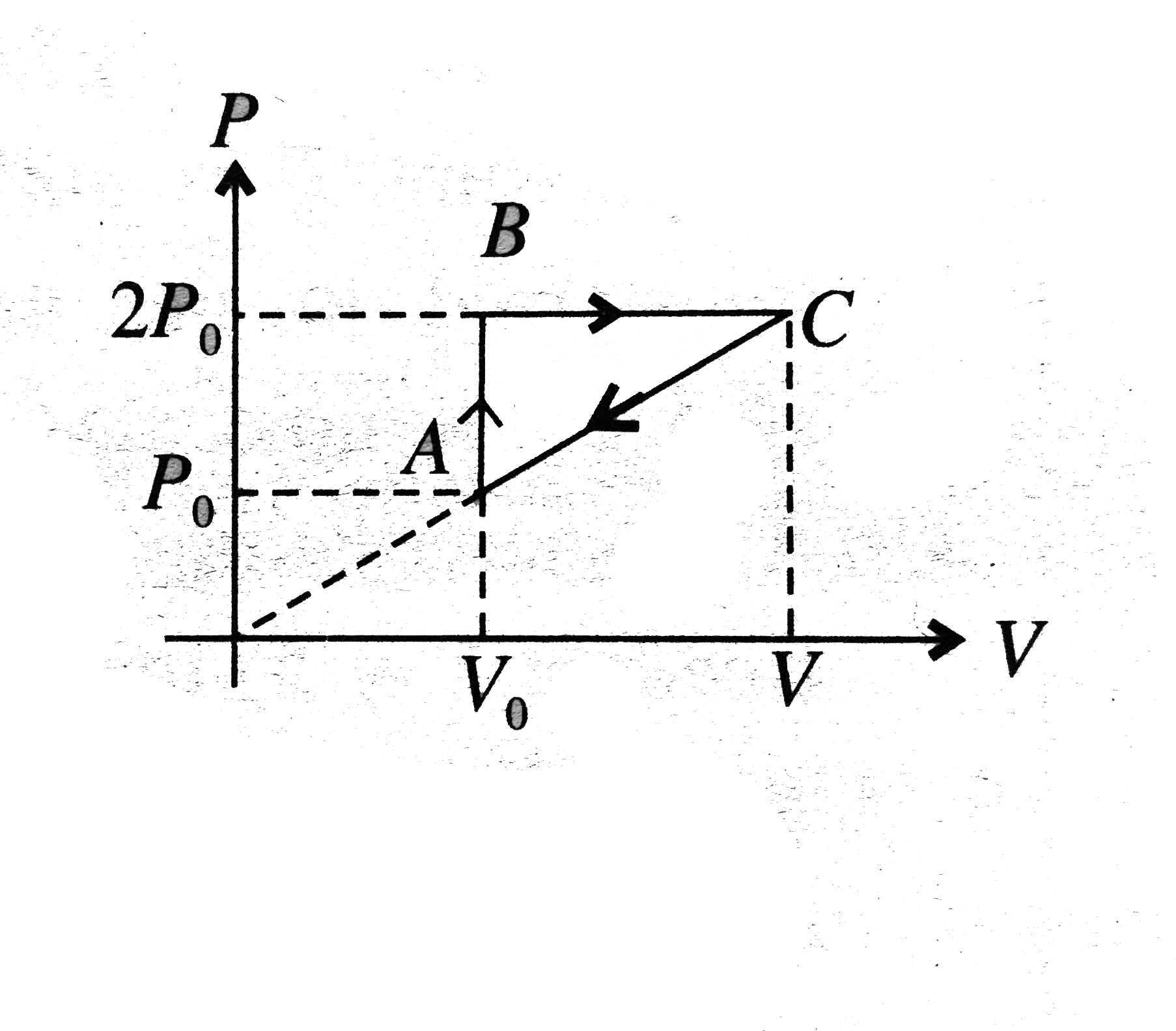
(i) For process AC,PαV
2P0Vc=P0V0⇒Vc=2V0
(ii) Since process AB is isochoric hence
PATA=PBTB⇒TB=2T0
since process BC is isobaric therefore TBVB=TCVC
⇒TC=2TB=4T0
(iii) Since process is cyclic therefore
ΔQ=W= area under the cycle =12P0V0.
(iv) Since ΔU and ΔW both are negative in process CA
∴ΔQ is negative in process CA and heat is rejected in process CA
ΔQCA=WCA+ΔUCA
=−12[P0+2P0]V0−52nR(TC−TA)
=−12[P0+2P0]V0−52nR(4P0V0nR−P0V0nR)
=−9P0V0 (Heat rejected)
(v) η= efficiency of the cycle =work done by the gasheat injected⇒η=P0V0/2Qinjected×100
ΔQ∈j=ΔQAB+ΔQBC
=[52nR(2T0−T0)]+[52nR(2T0)+2P0(2V0−V0)]=192P0V0.
η=10019%
Topper's Solved these Questions
- KTG & THERMODYNAMICSBOOK - RESONANCECHAPTER - KTG & THERMODYNAMICSEXERCISE - Solved problem
1 Videos
- KTG & THERMODYNAMICSBOOK - RESONANCECHAPTER - KTG & THERMODYNAMICSEXERCISE - Problem
3 Videos
- KTG & THERMODYNAMICSBOOK - RESONANCECHAPTER - KTG & THERMODYNAMICSEXERCISE - SUBJECTIVE QUESTIONS
27 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBOOK - RESONANCECHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Exercise
64 Videos
- MAGNETIC FIELD AND FORCESBOOK - RESONANCECHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Exercise
65 Videos
Similar Questions
Knowledge Check
n' moles of an ideal gas undergoes a process A→B as shown in the figure. The maximum temperature of the gas during the process will be:
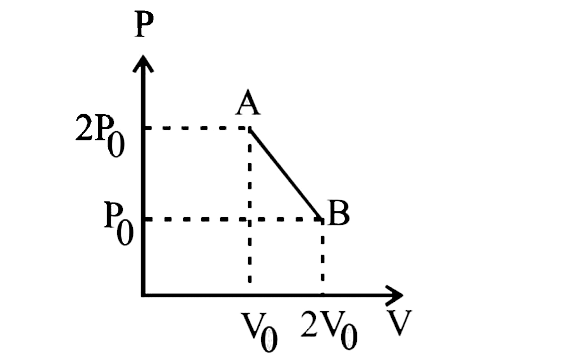 A9P0V02nRB9P0V0nRC9P0V04nRD3P0V02nR
A9P0V02nRB9P0V0nRC9P0V04nRD3P0V02nROne mole of a diatomic ideal gas undergoes a cyclic process ABC as shown in figure. The process BC is adiabatic. The temperature at A,B and C are 400K, 800K and 600K respectively. Choose the correct statement:
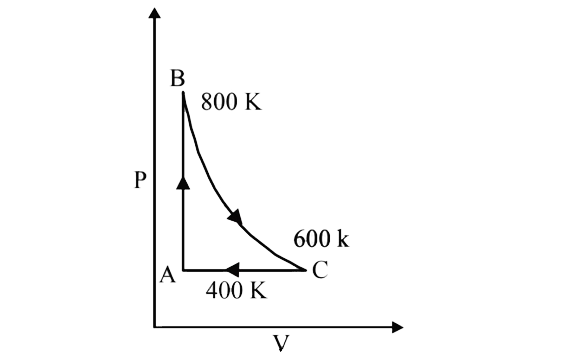 AThe change in internal energy in whole cyclic process is 250R.BThe change in internal process CA is 700R.CThe change in internal energy in the process AB is -350R.DThe chang in internal energy in the process BC is -500R.
AThe change in internal energy in whole cyclic process is 250R.BThe change in internal process CA is 700R.CThe change in internal energy in the process AB is -350R.DThe chang in internal energy in the process BC is -500R.Two moles of helium gas undergo a cyclic process as shown in figure. Assuming gas to be ideal, the net heat exchange in the process will be
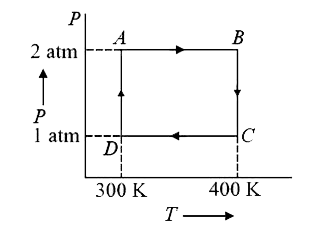 A1000 JB1152 JC1200 JD1500 J
A1000 JB1152 JC1200 JD1500 J
n moles of a diatomic gas has undergone a cyclic process ABC as shown in figure. Temperature at A is T0. Find
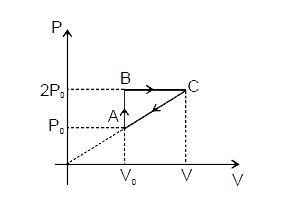 Total heat given to gas ?
Total heat given to gas ?
View Solutionn moles of a diatomic gas has undergone a cyclic process ABC as shown in figure. Temperature at A is T0. Find
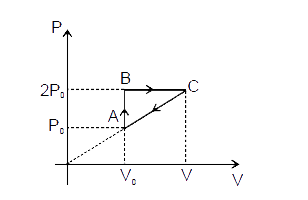
Find out the efficiency
View Solutionn moles of a diatomic gas has undergone a cyclic process ABC as shown in figure. Temperature at A is T0. Find
 is heat rejected by the gas, if yes how much heat is rrejected ?
is heat rejected by the gas, if yes how much heat is rrejected ?
View SolutionA sample of ideal gas is taken through the cyclic process shown in the figure. The temerauture of the gas in state A is TA =200 K. In states B and C the temperature of the gas is the same.
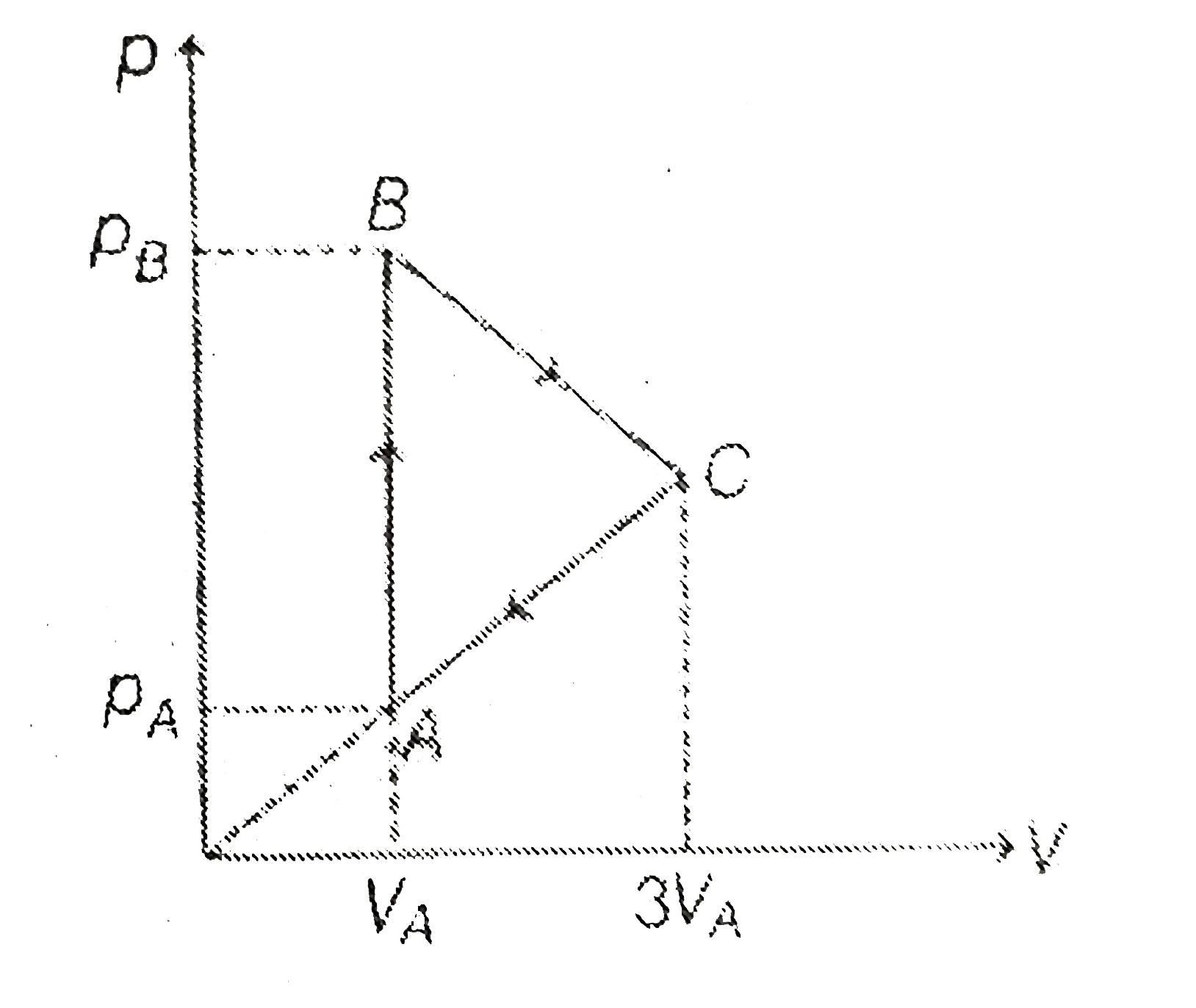
Net work done by the gas in the process is
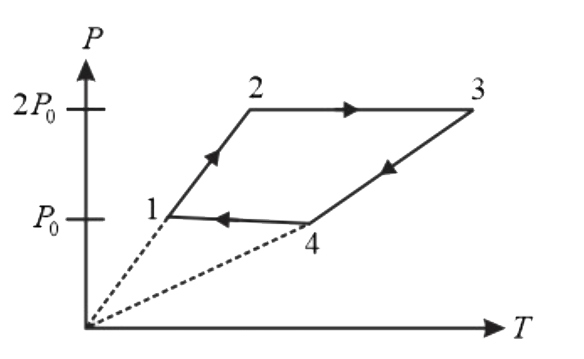
View SolutionOne mole of an ideal monoatomic gas undergoes a cyclic process, as shown in the figure. If the temperature of the gas at state 1 is 300 K and at state 4 is 500 K, then heat exchanged during process 2→3, is
View Solution