A point mass performs straight line motion along positive x-axis At t=0 point mass is at point A(x1,0) it moves such that its velocity is given by v=ax, where a is positive constant and x is the x-coordinate of position vector mass at a certain time t. Find the time required to move from A to B (x2,0)
x22−x212a
x22−x21a
2x22−x212a
2x22−x21a
The correct Answer is:A
To solve the problem, we need to find the time required for a point mass to move from point A at x1 to point B at x2 along the positive x-axis, given that its velocity is defined by the equation v=ax, where a is a positive constant.
1. Understand the Relationship Between Velocity and Position:
The velocity v can be expressed in terms of position x and time t as:
v=dxdt
Given that v=ax, we can equate the two expressions:
dxdt=ax
2. Rearranging the Equation:
We can rearrange the equation to separate variables:
xdx=adt
3. Integrating Both Sides:
Now, we integrate both sides. The left side will be integrated with respect to x from x1 to x2, and the right side will be integrated with respect to t from 0 to t:
∫x2x1xdx=∫t0adt
4. Calculating the Integrals:
The left side integral is:
∫xdx=x22∣∣x2x1=x222−x212
The right side integral is:
∫adt=at∣∣t0=at
5. Setting the Integrals Equal:
Now we set the results of the integrals equal to each other:
x222−x212=at
6. Solving for Time t:
Rearranging the equation to solve for t:
at=x22−x212
Thus, we can express t as:
t=x22−x212a
Final Answer:
The time required to move from point A to point B is:
t=x22−x212a
The velocity v can be expressed in terms of position x and time t as:
v=dxdt
Given that v=ax, we can equate the two expressions:
dxdt=ax
We can rearrange the equation to separate variables:
xdx=adt
Now, we integrate both sides. The left side will be integrated with respect to x from x1 to x2, and the right side will be integrated with respect to t from 0 to t:
∫x2x1xdx=∫t0adt
The left side integral is:
∫xdx=x22∣∣x2x1=x222−x212
The right side integral is:
∫adt=at∣∣t0=at
Now we set the results of the integrals equal to each other:
x222−x212=at
Rearranging the equation to solve for t:
at=x22−x212
Thus, we can express t as:
t=x22−x212a
The time required to move from point A to point B is:
t=x22−x212a
Topper's Solved these Questions
- TEST PAPERSBOOK - FIITJEECHAPTER - TEST PAPERSEXERCISE - PHYSICS
747 Videos
- SIMPLE HARMONIC MOTIONBOOK - FIITJEECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Assignment Problems (Objective) (Level-II)
20 Videos
- WORK, ENERGY AND POWER BOOK - FIITJEECHAPTER - WORK, ENERGY AND POWER EXERCISE - COMPREHENSIONS ( Comprehension-II)
5 Videos
Similar Questions
A particle located at position x=0, at time t=0, starts moving along the positive x-direction with a velocity v2=αx (where alpha is a positive constant). The displacement of particle is proportional to
View SolutionA particle moves along a straight line such that its positionx at any time t is x=3t2−t3, where x is in metre and t in second the
View Solution
Knowledge Check
A point moves in xy-plane according to equation x = at, y = at (l - bt) where a and b are positive constants and t is time. The instant at which velocity vector is at π/4 with acceleration vector is given by:
A(1a)B(1b)C(1a+1b)Da+ba2+b2The position x of a particle at time t is given by x=V0a(1−e−at), where V0 is constant and a>0. The dimensions of V0 and a are
AM−0LT−1andT−1BM0LT0andT−1CM0LT−1andLT−2DM0LT−1andTThe position x of a particle at time t is given by x=V0a(1−e−at), where V0 is constant and a>0. The dimensions of V0 and a are
A[M0LT−1] and [M0L0T−1]B[M0LT0] and [M0LT−1]C[M0LT−1] and [MLT−2]D[M0LT−1] and [M0LT]
An object is in unifrom motion along a straight line . What will be position-time graph for themotion of the object if
(a) x0=+ve,v=+ve (b) x0=+ve,v=−ve(c)x_(0) =- ve, v= + veand(d)⊥hx_(0) and v are negative ? The letters x0 and v position of theobject at time t=0 and v represent posituion of the object at time t=0 and uniform velocity of theobject respectively.
View SolutionAn object is in unifrom motion along a straight line . What will be position-time graph for themotion of the object if
(a) x0=+ve,v=+ve (b) x0=+ve,v=−ve(c)x_(0) =- ve, v= + veand(d)⊥hx_(0) and v are negative ? The letters x0 and v position of theobject at time t=0 and v represent posituion of the object at time t=0 and uniform velocity of theobject respectively.
View SolutionA disc of radius R start at time t=0 moving along the positive X-axis with linear speed b and angular speed ω. Find the x and y-coordinates of the bottommost point at any time t.
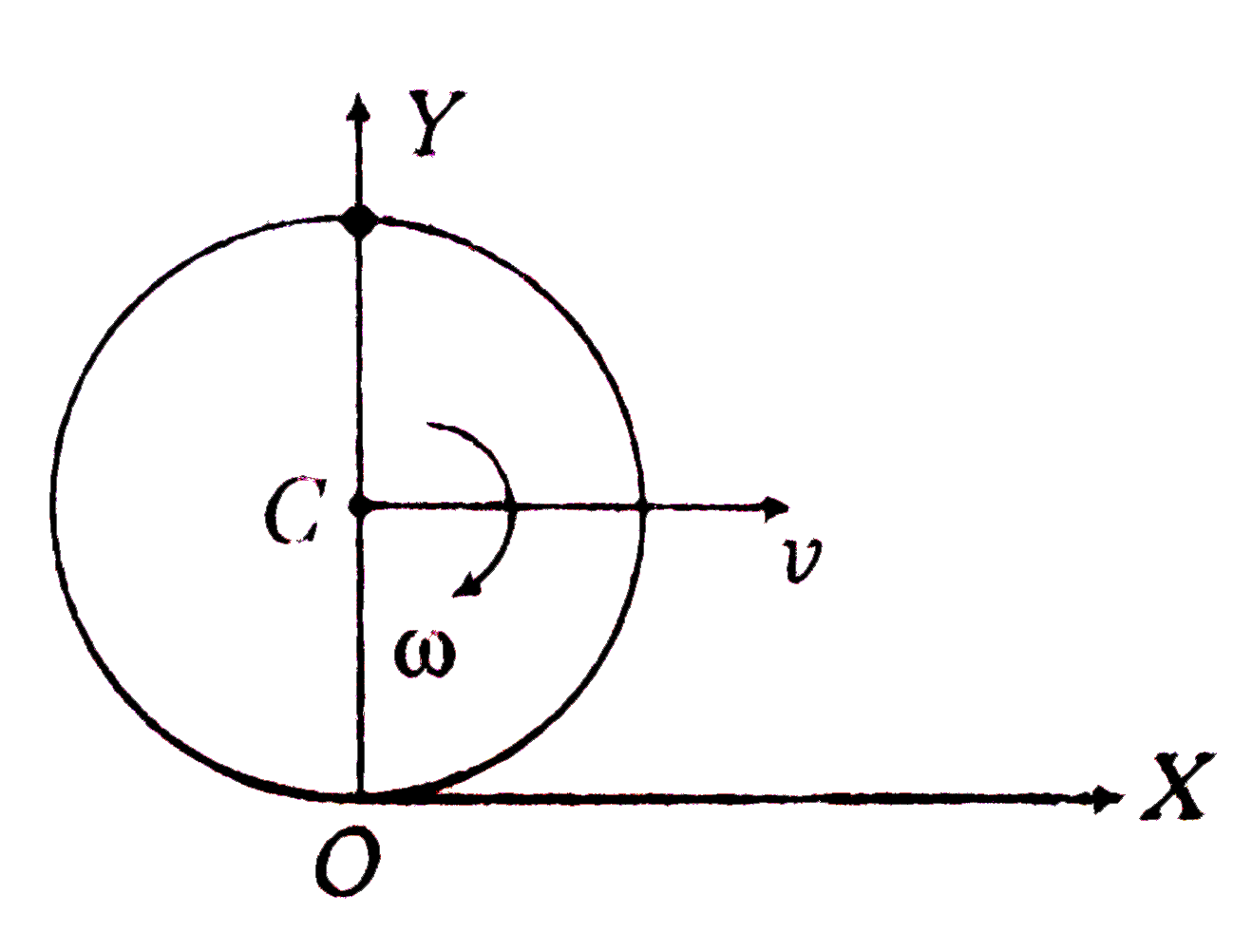
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionA particle of mass m moving along a straight line is acted on by (always directed against the motion) F−beαv where b and α are postive constants and x is the velocity. At t-0 it is moving with velocity V0. At what time does it come to rest?
View Solution