A convex lens is held 45cm above the bottom of an empty tank. The image of a point at the bottom of a tank is formed 36cm above the lens. Now, a liquid is poured into the tank to a depth of 40cm. It is found that the distance of the image of the same point on the bottom of the tank is 48cm above the lens. Find the refractive index of the liquid.
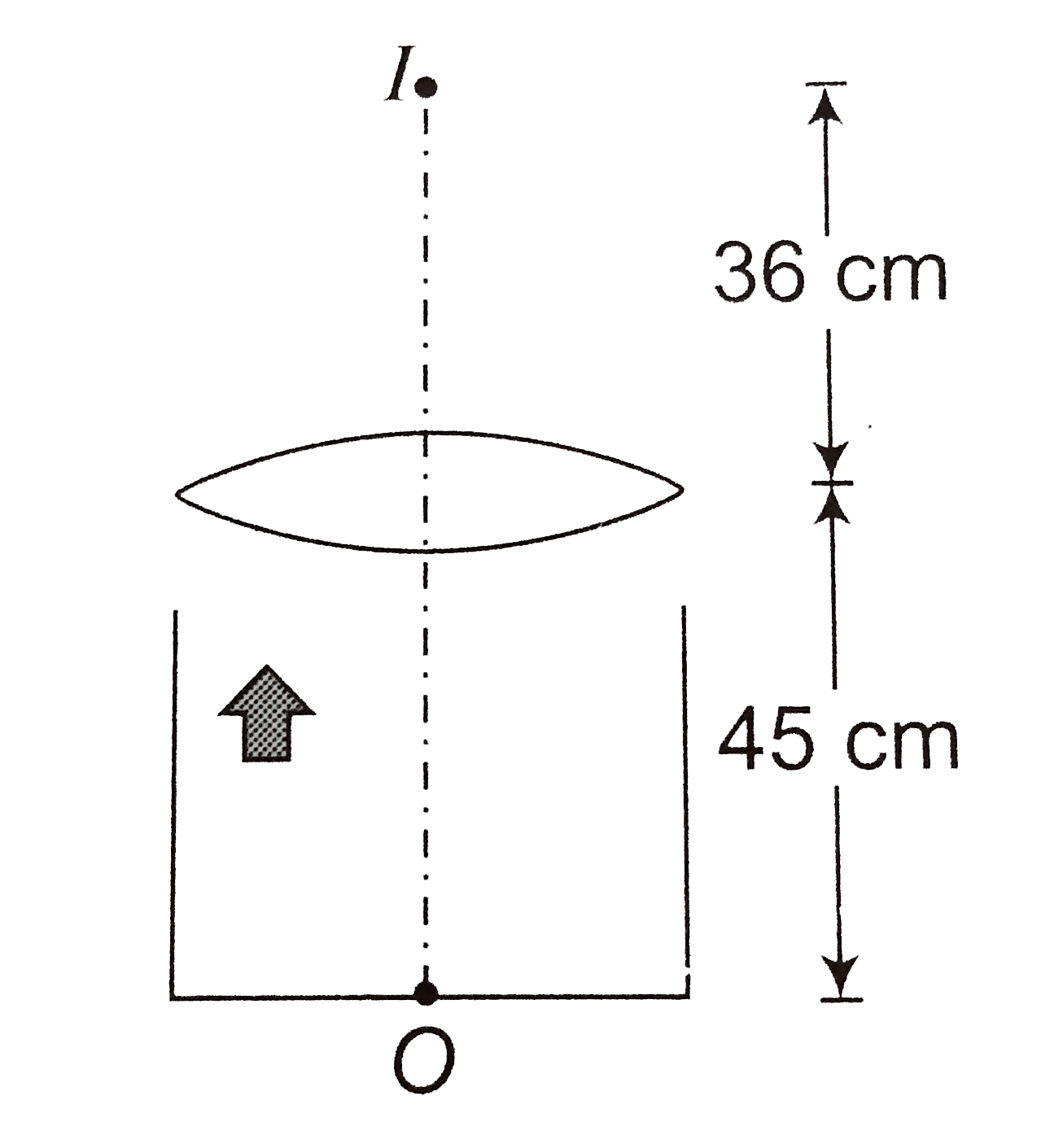
1f=1v−1u=136−1−45=3+4180
f=20cm
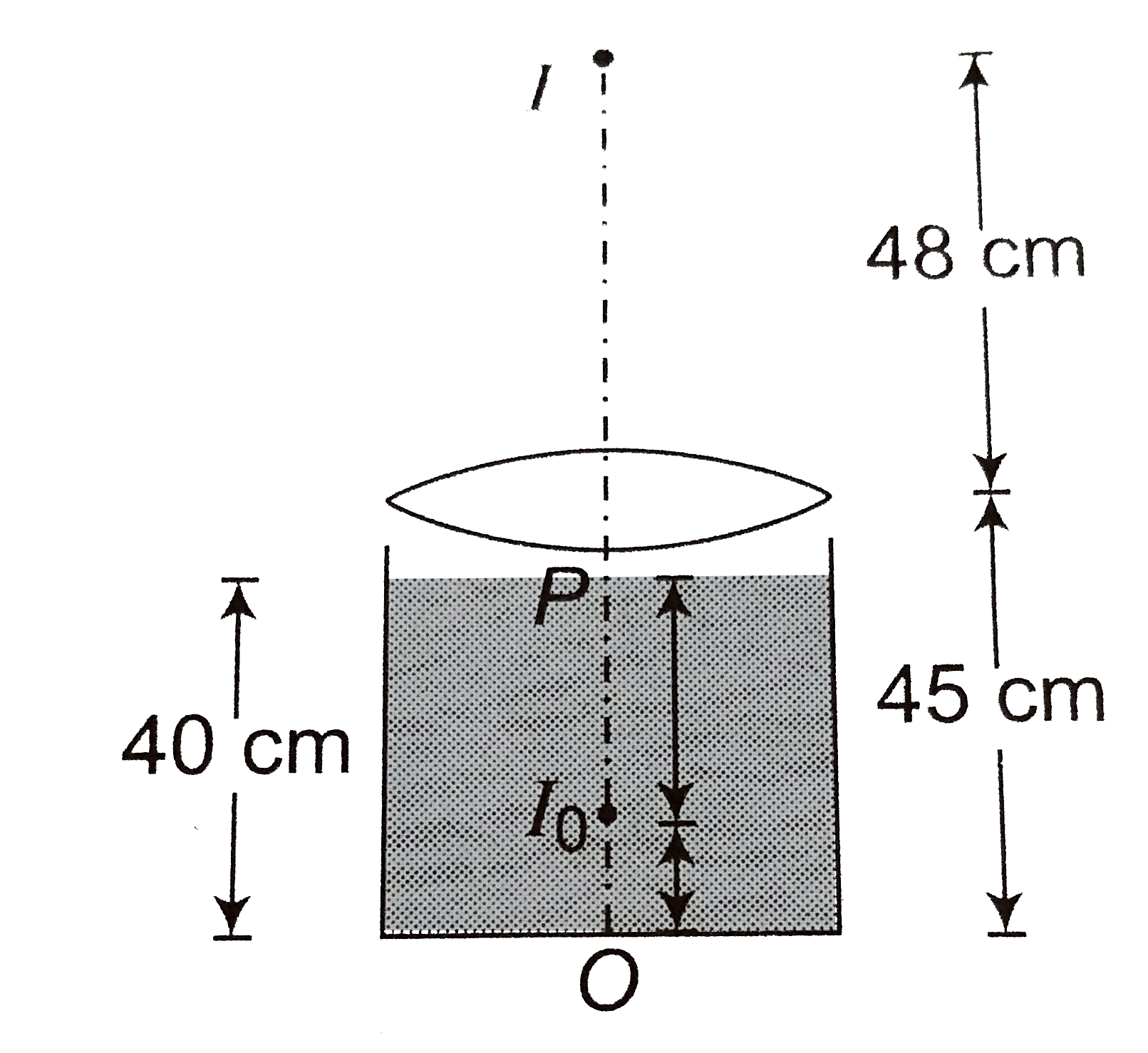
Shift of object due to liquid =40(1−1μ)=40−40μ
u=−[45−(40−40μ)]=−5−40μ
v=48cm,f=20cm
1v−1u=1f
1u=1v−1f=148−120=5−12240
u=−2407cm
u=−5−40μ
−2407=−5−40μ
40μ=−5+2407=2057
μ=280205=5641
Topper's Solved these Questions
- REFRACTION AT SPHERICAL SURFACESBOOK - CP SINGHCHAPTER - REFRACTION AT SPHERICAL SURFACESEXERCISE - EXERCISES
105 Videos
- REFLECTION OF LIGHTBOOK - CP SINGHCHAPTER - REFLECTION OF LIGHTEXERCISE - EXERCISES
67 Videos
- REFRACTION OF LIGHT BY PLANE SURFACESBOOK - CP SINGHCHAPTER - REFRACTION OF LIGHT BY PLANE SURFACESEXERCISE - Exercises
110 Videos
Similar Questions
A boy holds a convex lens 30 cm above the base of an empty vessel. The real image of the bottom of the vessel is formed 20 cm above the lens. The boy fills a liquid in the vessel up to a depth of 25 cm and finds that the real image of the bottom of the vessel is now 30 cm above the lens. Find the refractive index of the liquid.
View SolutionA coin placed at the bottom of a tank appears to be raised when water is poured into it. Explain.
View Solution
Knowledge Check
The pressure at the bottom of a liquid tank does not depend on :
AAcceleration due to gravityBdensity of the liquidCheight of the liquidDarea of the liquid surface
A real image of an object is formed by a conex lens at the bottom of an empty beaker. The beaker is now filled with a liquid of refractive index 1.4 to a depth of 7 cm. In order to get the image again at the bottom the beaker shoud be movedAdownward by 2 cmBupward by 2 cmCdownward by 3 cmDupward by 3 cmThe velocity of flow of a liquid through an orifice at the bottom of a tank depends on the :
Aarea of the cross - section of the orificeBheight of the liquid above the orificeCacceleration due to gravityDboth (b) and (c )
The bottom of a rectangular swimming tank is 50 cm x 20 cm. Water is pumped into the tank at the rate of 500 cc/mm. Find the rate at which at level of water in the tank is rising
View SolutionThe apparent depth of an object at the bottom of tank filled with a liquid of refractive index 1.3 is 7.7 cm. what is the actual depth of the liquid in the tank ?
View SolutionThe pressure at the bottom of a tank containing a liquid does not depend on
View SolutionThe velocity of efflux of a liquid through an orific in the bottom of the tank does not depend upon
View SolutionThe pressure at the bottom of a tank containing a liquid does not depend on
View Solution
