A spherical air lens of radii R1=R2=10cm
Is cut in a glass (μ=1.5) cylinder. Determine the focal length and nature of air lens. If a liquid of refractive index 2 is filled in the lens, what will happen to its focal length and nature?
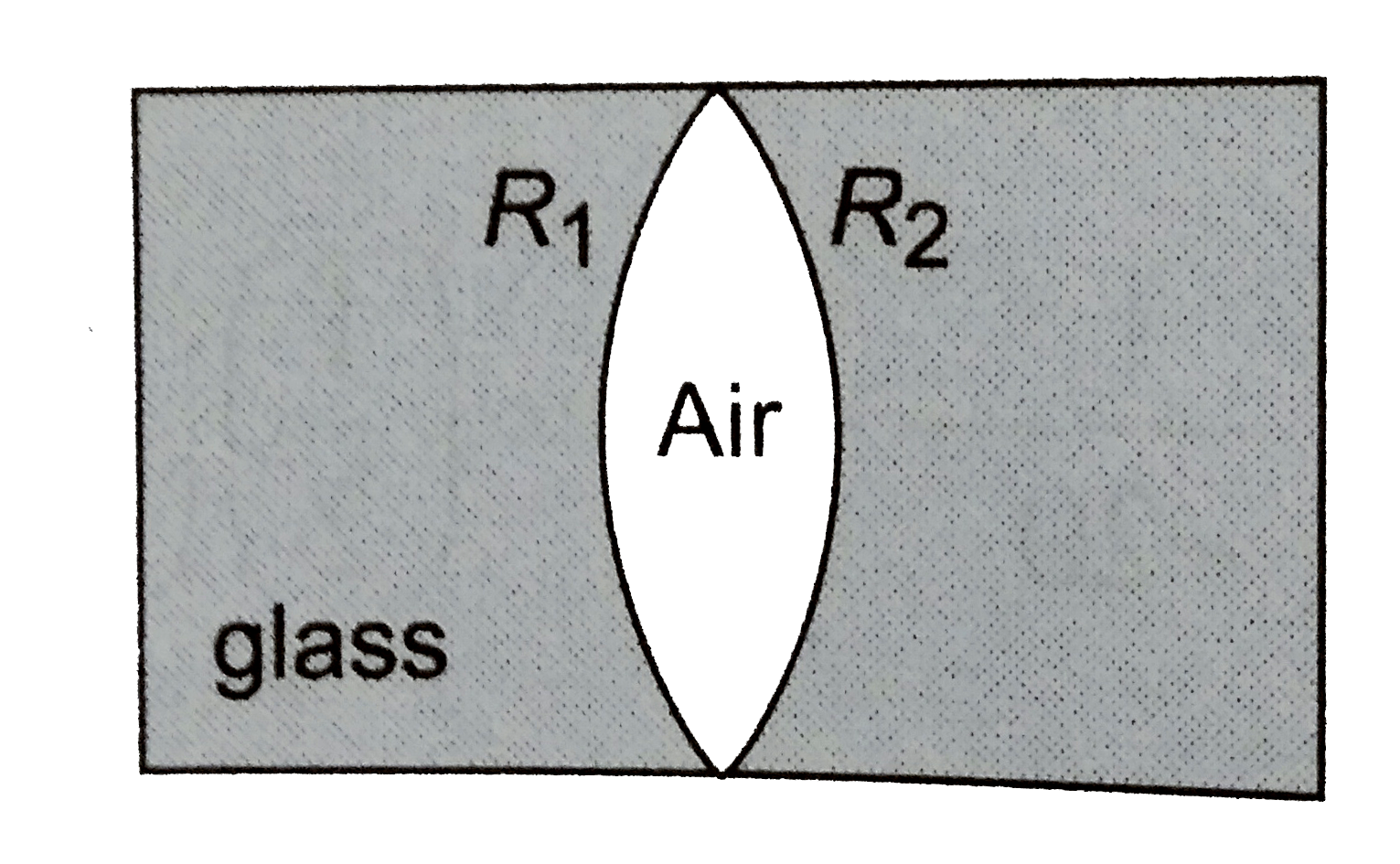
1fairlens=(_aμa−1)(1R1−1R2)
(1_aμg)−1)(1R1−1R2)
=⎛⎝132−1⎞⎠(110−1−10)=(−13)(210)
fairlens=−15cm
1fl=(_aμl−1)(1R1−1R2)
=((_aμg_l)_aμg)−1)(1R1−1R2)
=⎛⎝232−1⎞⎠(110−1−10)
=(13)(210)
f1=15cm
Nature of lens changes.
Topper's Solved these Questions
- REFRACTION AT SPHERICAL SURFACESBook:CP SINGHChapter:REFRACTION AT SPHERICAL SURFACESExercise:EXERCISES
Explore 105 Videos
- REFLECTION OF LIGHTBook:CP SINGHChapter:REFLECTION OF LIGHTExercise:EXERCISES
Explore 67 Videos
- REFRACTION OF LIGHT BY PLANE SURFACESBook:CP SINGHChapter:REFRACTION OF LIGHT BY PLANE SURFACESExercise:Exercises
Explore 110 Videos
Similar Questions
Knowledge Check
A lens of refractive index n is put in a liquid of refractive index n'. If focal length of lens in air is f, its focal length in liquid will be.
Afn'(n−1)n'−nBf(n'−1)n'(n−1)Cn'(n−1)f(n'−1)Dfn'nn−n'In the figure, an air lens is cut a cylinder of glass of refractive index 1.5 . The nature of the lens is
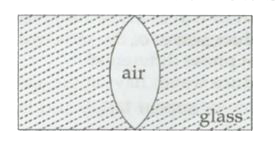 AdiverigingBconvergingCneither diverging nor convergingDnone of these
AdiverigingBconvergingCneither diverging nor convergingDnone of theseA lens of refractive index n is put in a liquid of refractive index n'. If focal length of lens in air is f, its focal length in liquid will be.
A−fn'(n−1)n'−nB−f(n'−n)n'(n−1)C−n'(n−1)f(n'−n)Dfn'nn−n'
A convex lens is dipped in a liquid whose refractive index is equal to the refractive index of the lens. Then its focal length will
View SolutionA thin equiconvex lens (μ=3/2) of focal length 10cm is cut and separated and a material of refractive index 3 is filled between them. What is the focal length of the combination?
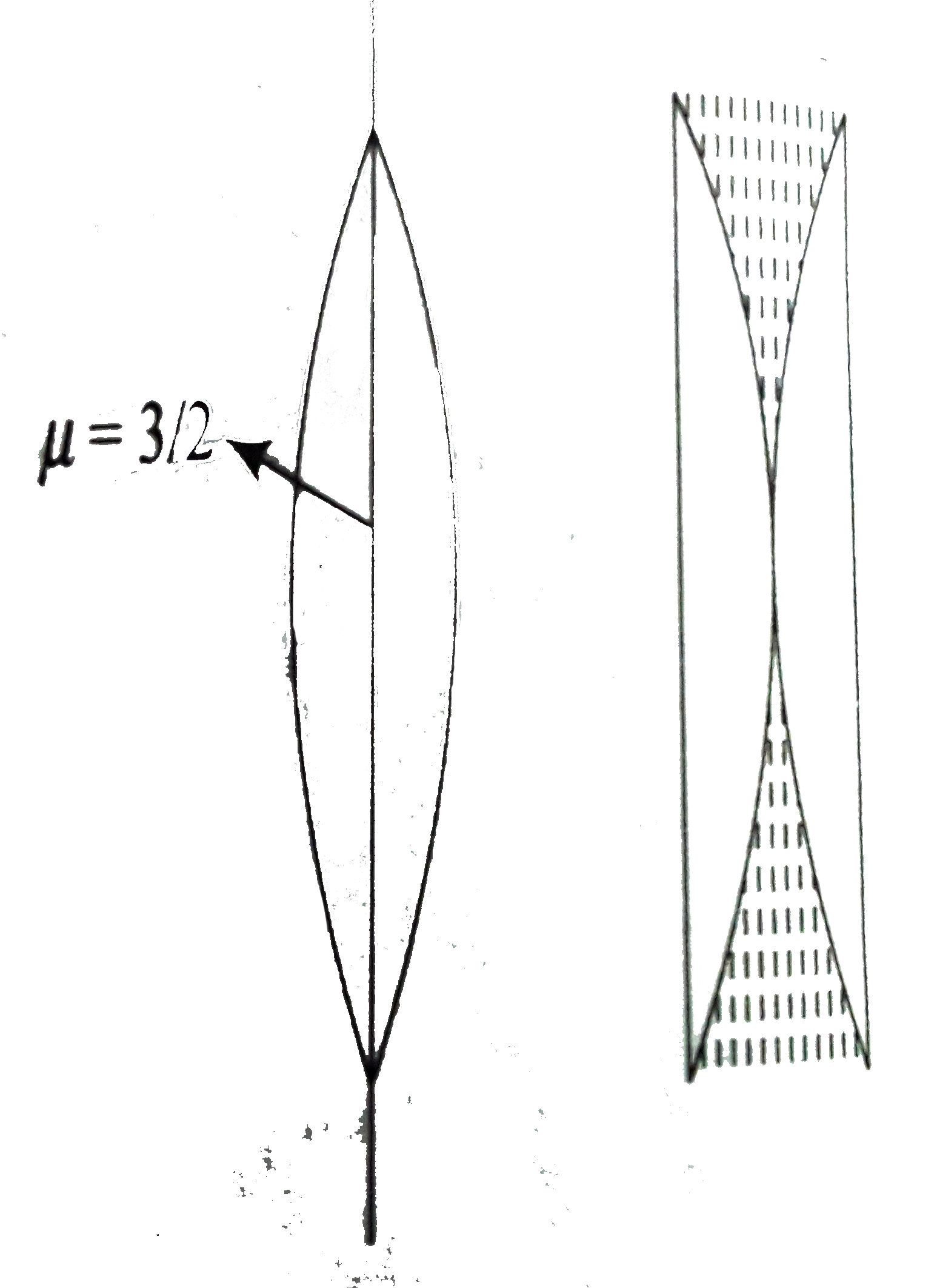
View SolutionA convex glass lens of focal length 20 cm and refractive index 1.5 is immersed in water of R.I. 4/3. What is the change in its focal length ?
View SolutionThe focal length of lens of refractive index 1.5in air is30cmWhen it is immersed in water of refractive index43,then its focal length will be
View SolutionA convex lens of focal length 20 cm made of glass of refractive index 1.5 is immersed in water having refractive index 1.33. The change in the focal length of lens is
View Solution