A mark of the surface of sphere is viewed from a diametrically opposite position. It appears to be at a distance 15 cm from its actual position.Find the radius of sphere.
To solve the problem of finding the radius of the sphere when a mark on its surface appears to be at a distance of 15 cm from its actual position, we will follow these steps:
Step 1: Understand the Setup
We have a sphere with a refractive index μ=32. A mark on the surface of the sphere is viewed from a diametrically opposite position, and it appears to be 15 cm away from its actual position.
Step 2: Define the Variables
- Let r be the radius of the sphere.
- The object (mark on the surface) is at a distance u from the center of the sphere, which is −2r (negative because it is on the left side of the reference point).
- The apparent distance of the image v is −2r+15 (also negative).
Step 3: Apply the Refraction Formula
Using the formula for refraction at spherical surfaces:
μ2v−μ1u=μ2−μ1r
where:
- μ1=32 (for the sphere)
- μ2=1 (for air)
Substituting the values:
1−2r+15−32−2r=1−32r
Step 4: Simplify the Equation
Rearranging the equation:
1−2r+15+3/22r=−12r
This simplifies to:
1−2r+15+34r=−12r
Step 5: Find a Common Denominator
The common denominator for the left side is 4r(−2r+15):
4r+3(−2r+15)4r(−2r+15)=−2(−2r+15)4r(−2r+15)
Step 6: Solve for r
Setting the numerators equal:
4r+3(−2r+15)=−2(−2r+15)
Expanding and simplifying:
4r−6r+45=4r−30
Combine like terms:
−2r+45=4r−30
Rearranging gives:
45+30=4r+2r
75=6r
Thus:
r=756=12.5 cm
Final Answer
The radius of the sphere is 12.5 cm.
We have a sphere with a refractive index μ=32. A mark on the surface of the sphere is viewed from a diametrically opposite position, and it appears to be 15 cm away from its actual position.
- Let r be the radius of the sphere.
- The object (mark on the surface) is at a distance u from the center of the sphere, which is −2r (negative because it is on the left side of the reference point).
- The apparent distance of the image v is −2r+15 (also negative).
Using the formula for refraction at spherical surfaces:
μ2v−μ1u=μ2−μ1r
where:
- μ1=32 (for the sphere)
- μ2=1 (for air)
1−2r+15−32−2r=1−32r
Rearranging the equation:
1−2r+15+3/22r=−12r
This simplifies to:
1−2r+15+34r=−12r
The common denominator for the left side is 4r(−2r+15):
4r+3(−2r+15)4r(−2r+15)=−2(−2r+15)4r(−2r+15)
Setting the numerators equal:
4r+3(−2r+15)=−2(−2r+15)
Expanding and simplifying:
4r−6r+45=4r−30
Combine like terms:
−2r+45=4r−30
Rearranging gives:
45+30=4r+2r
75=6r
Thus:
r=756=12.5 cm
The radius of the sphere is 12.5 cm.
Topper's Solved these Questions
- REFRACTION AT SPHERICAL SURFACESBook:CP SINGHChapter:REFRACTION AT SPHERICAL SURFACESExercise:EXERCISES
Explore 105 Videos
- REFLECTION OF LIGHTBook:CP SINGHChapter:REFLECTION OF LIGHTExercise:EXERCISES
Explore 67 Videos
- REFRACTION OF LIGHT BY PLANE SURFACESBook:CP SINGHChapter:REFRACTION OF LIGHT BY PLANE SURFACESExercise:Exercises
Explore 110 Videos
Similar Questions
A small object stuck on the surface of a glass sphere (μ=4/3) is viewed from the diametrically opposite position find transverse magnification.
View SolutionA small object stuck on the surface of a glass sphere (n=1.5) is viewed from the diametrically opposite position. Find transverse magnification.
View Solution
Knowledge Check
A sphere of radius 5 cm is cut by a plane at a distance of 4 cm from its centre. Find the radius of circular section hence formed.
A3 cmB5 cmC4 cmD6 cm
Find the surface area of sphere if radius is 9cm?
View SolutionA mark placed on the surface of glass sphere is viewed through glass from an oppositely directed position. If the diameter of the sphere is 30 cm, locate the image of the mark. For glass, μ=1.5
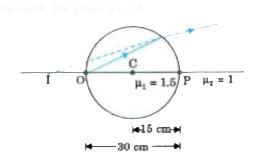
View SolutionA mark placed on the surface of a sphere is viewed through glass from a position directly opposite. If the diameter of the sphere is 10cm and refractive index of glass is 1.5, find the position of the image.
View SolutionFind the surface area of a sphere of radius 7cm.
View SolutionFind the surface area of a sphere of radius 7 cm.
View SolutionFind the surface area of a sphere of radius 14cm.
View SolutionFind the surface area of a sphere of radius 7 cm.
View Solution