A conducting loop of area A is placed in a magnetic field which varies sinusoidally with time as B=B0sin
(a) ϕ=Bacosθ=B0sinωtcos60∘=B∘Asinωt2
e=dϕdt=B0Aωcosωt2
e is maximum ,when cosωt=I
emax=I2B0Aω
(b) e=B0Aω2cosωt
At t=T/6
e=B0Aω2cos(ωT6)
=B0Aω2cos(π3)=B0Aω4(∴ω=2πT)
I=e/R=B0Aωcosωt2R
q=∫T0Idt=B0Aω2R∫T0cosωtdt=0
(d) H=∫T0e2Rdt=B20A2ω∘4R∫T0cos2ωtdt
=B20A2ω24RT2=B20A2ω24Rπω
=πB20A2ω4R
Topper's Solved these Questions
Similar Questions
A conducting loop of area 5.0cm2 is placed in a magnetic field which varies sinusodially with time as B=B0sinωt where B0=0.20T and ω=300s−1. The normal to the coil makes an angle of 60∘ with the field. Find (a) the maximum emf induced in the coil, (b) the emf induced at τ=(τ900)s and (c) the emf induced at t=(π600)s.
View SolutionA conducting metal circular-wire-loop of radius r is placed perpendicular to a magnetic field which varies with time as B=B0e−t/τ, where B0andτ are constants, at time = 0. If the resistance of the loop is R then the heat generated in the loop after a long time (t→∞) is :
View Solution
Knowledge Check
A conducting looop of area 5.0cm2 is placed in a magnetic field which varies sinusoidally with time as B=0.2sin300t . The normal to the coil makes an angle of 60∘ with the field. The emf induced at t=(π/900)s
A7.5×10−3VBzeroC15×10−3VD20×10−3VA stationary circular loop of radius a is located in a magnetic field which varies with time from t=0→t=T according to law B=B0t(T−t). If plane of loop is normal to the direction of field and resistance of the loop is R, calculate
amount of heat generated in the loop during this interval.Aπ2a4B20T33RBπ2a4B20T3RC3π2a4B20T3RDNone of theseA stationary circular loop of radius a is located in a magnetic field which varies with time from t = 0 to t = T according to law B=B0t(T−t). If plane of loop is normal to the direction of field and resistance of the loop is R, calculate
magnitude of charge flown through the loop from instant t = 0 to the instant when current reverses its direction.Aπ2a2B0T2RBπ2a2B0T24RC4π2a2B0T2RDNone of these
A circular brass loop of radius a and resistance R is placed with it plane perpendicular to a magnetic field, which varies with time as B=B0sinωt. Obtain the expression for the induced current in the loop.
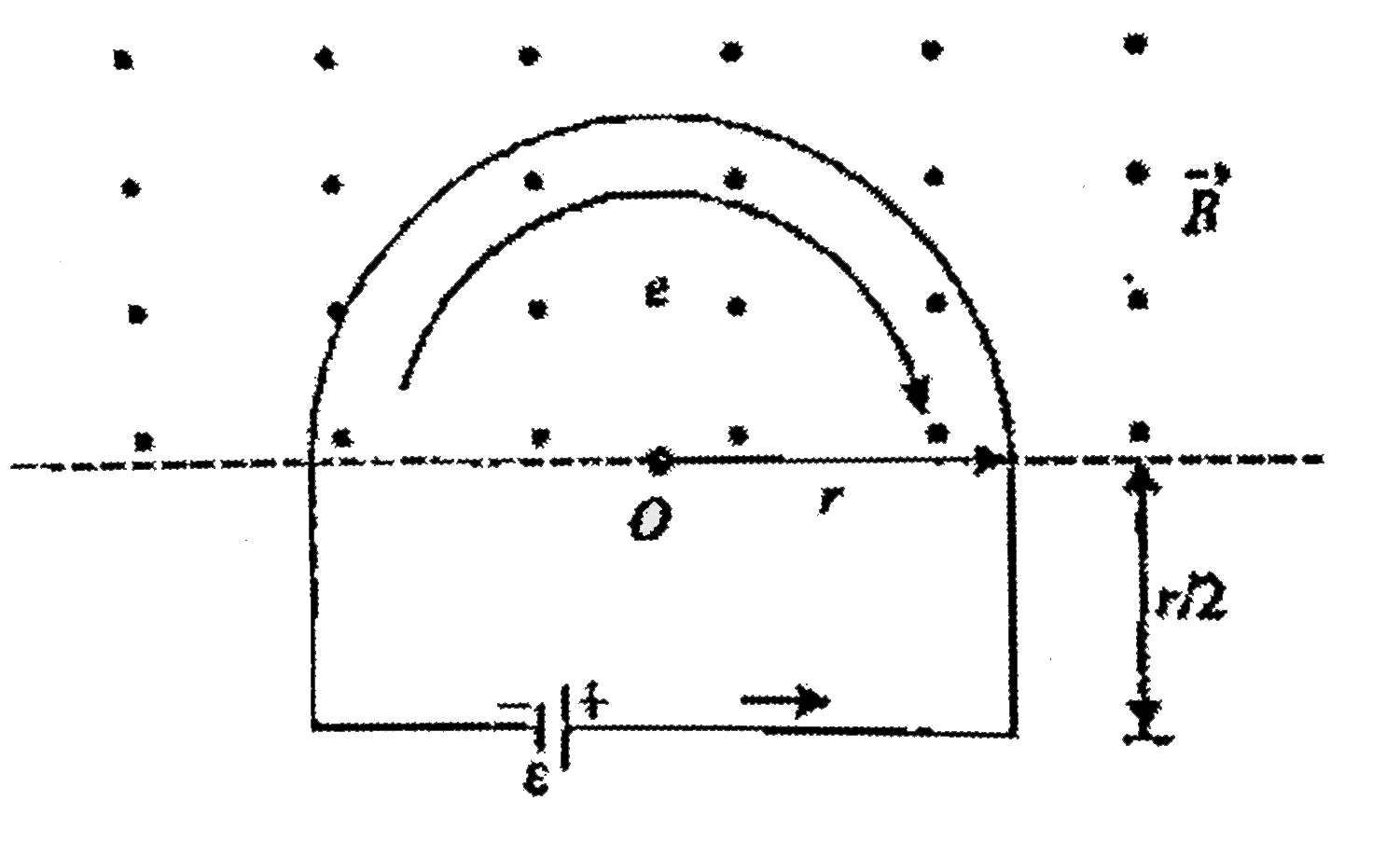
View SolutionFigure-5.72 shows a conducting loop of which semi-circular part lies in the magnetic induction B which varies with time, given as
B=at3+ct3+fT
The wire is having a resistance Rω/m. Find current in loop at time t=2s.
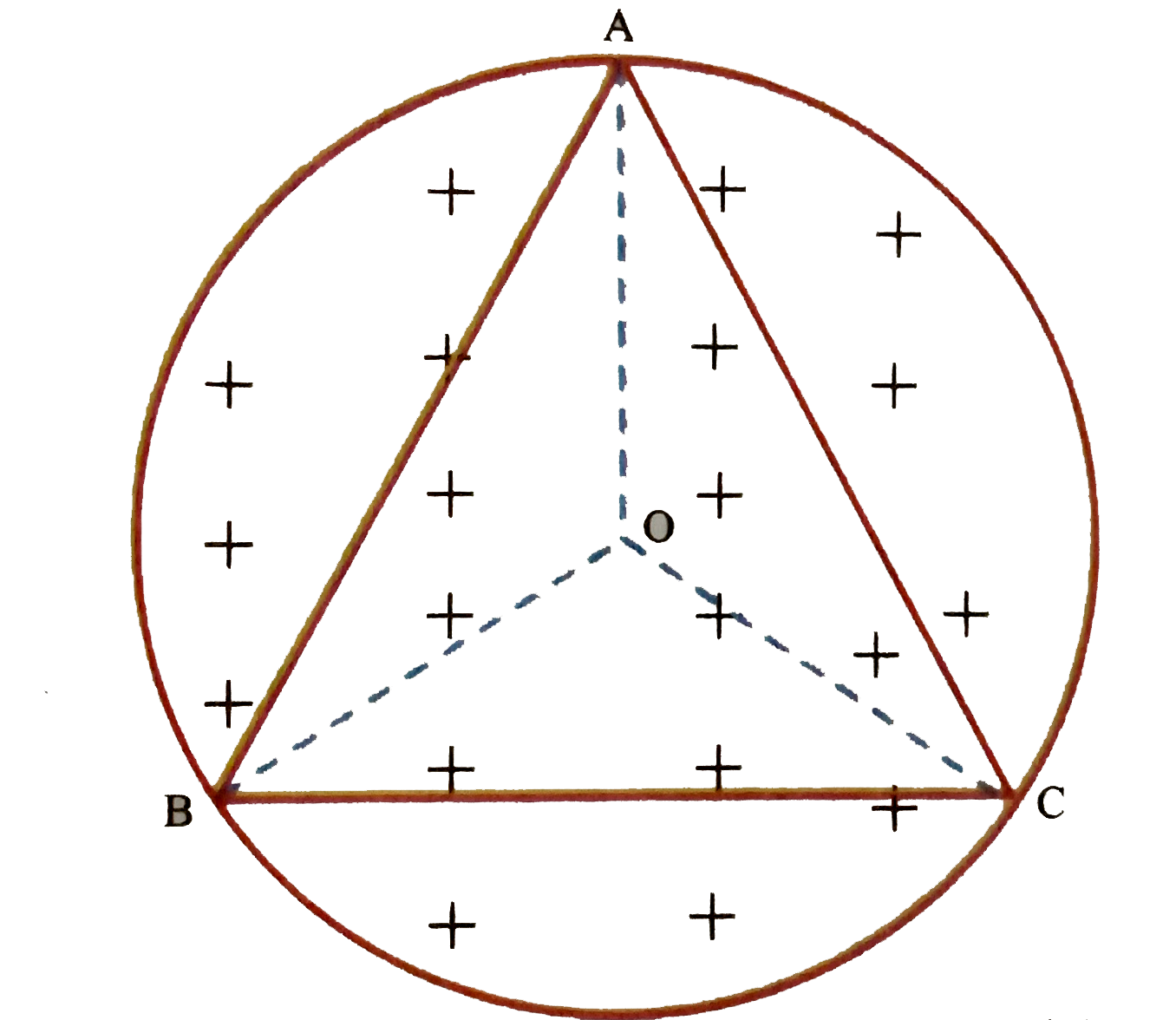
View SolutionIn the figure shown there exists a uniform time varying magnetic field B=[(4T/s)t+0.3T] in a cylinderical region of radius 4m. An equilateral traingualr conducting loop is placed in the magnetic field with its centroide on the axis of the field and its plane perpendicular to the field.
View SolutionA conducting square loop is placed in a magnetic field B with its plane perpendicular to the field. Now the sides of the loop start shrinking at a constant rate α. the induced emf in the loop at an instant when its side is a is
View SolutionA conducting square loop is placed in a magnetic field B with its plane perpendicular to the field. Now the sides of the loop start shrinking at a constant rate α. the induced emf in the loop at an instant when its side is a is
View Solution
