A spring of force constant k and natural length 6l is broken in three parts of length ratio 1:2:3 find the spring constant of each part.
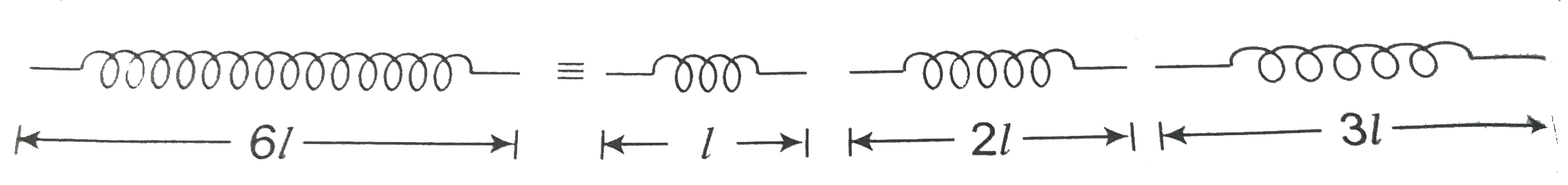
For the spring: spring constant × natural length=constant
k×6l=k1×l=k2×2l=k3×3l
k1=6k,k2=3k,k3=2k
Topper's Solved these Questions
Similar Questions
A spring of stiffness constant k and natural length l is cut into two parts of length 3l/4andl/4, respectively, and an arrangement is made as shown in figure . If the mass is slightly displaced , find the time period of oscillation.
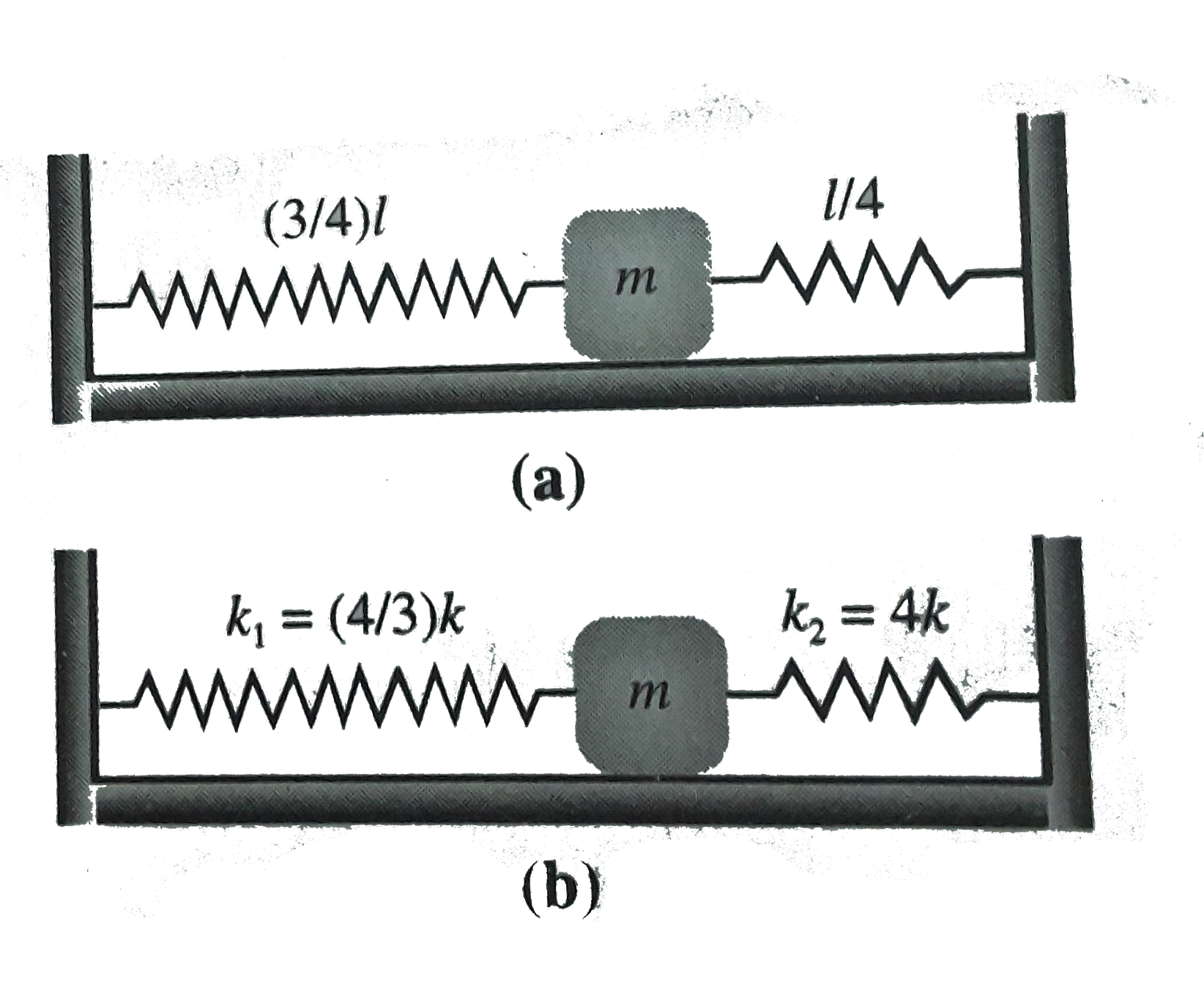
View Solution
A ring of mass m is attached to a horizontal spring of spring constant k and natural length l0. The other end of the spring is fixed and the ring can slide on a horizontal rod as shown. Now the ring is shifted to position B and released Find the speed of the ring when the spring attains its natural length.
View Solution
Knowledge Check
A spring fo spring constant k is broken in the length ratio 1:3. The spring constant of larger part will be
A4k/3B2k/3Ck/3D5k/3A spring of constant K is cut into two parts of length in the ratio 2:3 . The spring constant of large spring is
A53KB23KCkD35kA spring of certain length and having spring constant k is cut into two pieces of length in a ratio 1:2. The spring constants of the two pieces are in a ratio :
A1:1B1:4C1:2D2:1
A spring of force constant k is cut into two pieces whose lengths are in the ratio 1:2. The force constant of the longer piece?
View SolutionA spring of force constant k is cut into there equal part what is force constant of each part ?
View SolutionA spring of force constant k is cut into two parts whose lengths are in the ratio 1:2. The two parts are now connected in parallel and a block of mass m is suspended at the end of the combined spring. The period of oscillation of block is
View SolutionAssertion A spring of force constatn k is cut in to two piece having lengths in the ratio 1:2 The force constant of series combination of the two parts is 3k2
The spring connected in series are represented by k=k1+k2
View SolutionA spring of force constant k extends by a length X on loading . If T is the tension in the spring then the energy stored in the spring is
View Solution