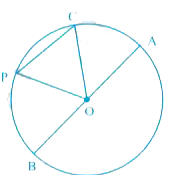
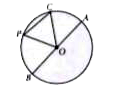
If O is the centre of a circle with radius r and AB is a chord of the circle at a distance r2 from O, then ∠BAO=
Video Solution
Text Solution
Let∠OAB=θ
sin θ=OLOA=r2/r=12=sin300
θ=300
∠BAO=300
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the given figure, O is the centre of a circle of radius 13 cm and AB is a chord perpendicular to OD. If CD = 8 cm, then what is the length (in cm) of AB?
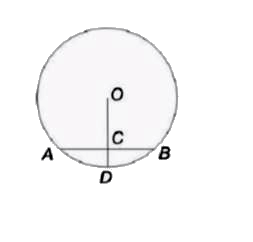 AA)6 cmBB)12 cmCC)24 cmDD)28cm
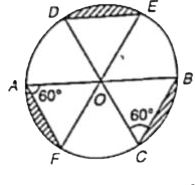
AA)6 cmBB)12 cmCC)24 cmDD)28cmIn the adjoining figure O is the centre of the circle with radius r’ AB, CD and EF are the diameters of the circle. angleOAF = angleOCB = 60^(@) . What is the area of the shaded region?
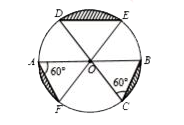 Ar22(π−3√32)Br22(π−3√34)Cr23(π−2√33)DData insuffincient
Ar22(π−3√32)Br22(π−3√34)Cr23(π−2√33)DData insuffincientO is the centre of a circle. AB is a chord of the circle but not its diameter. OC is perpendicular to AB. If OC = CB and radius of the circle be 7 cm, then the length of AB is
A7√2cmB14cmC7 cmD7√2 cm
In the adjoining figure O is the centre of the circle with radius 'r' AB, CD and EF are the diameters of the circle. ∠OAF=∠OCB=60∘. What is the area of the shaded region?
View SolutionIn given figure, O is the centre of the circle.
A chord, which is not the diameter of the circle is
View SolutionIn the adjoining figure, O is the centre of the circle. If the chord AB is equal to the radius of the circle, then find the value of ∠ADB.

View SolutionAB is the diameter of a circle whose centre is O. DC is a chord of that circle. If DC||AB and ∠BAC=20∘ then ∠ADC=?
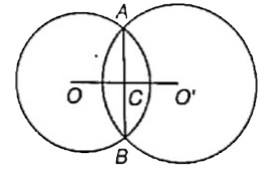
View SolutionO and O' are the centres of circle of radii 20cmand30cmAB=24cm. What is the distance O O' ?
View Solution