In ABC, ∠B=350,∠C=650
and the bisector of ∠BAC
meets BC
in P.
Arrange AP, BP and CP
in descending order.
Given : ∠B=35∘and∠C=65∘
Solution : We have to arrange AP, BP and CP in descending order.
In △ACP
∠ACP=65∘
∠CAP=40∘( As AP is the bisector of ∠CAB )
So AP > CP ( Sides in front or greater angle will be greater )________1
In △ABP
∠BAP>∠ABP
So BP > AP ______2
Hence, from 1 and 2
BP>AP>CP
Topper's Solved these Questions
Similar Questions
Knowledge Check
In ΔABC,∠B=35∘,∠C=65∘ and the bisector of ∠BAC meets BC in D? Then ∠ADB is :
A40∘B75∘C90∘D105∘
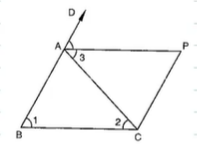
In figure AB=AC and CP∣∣BA and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA (ii) ABCP is a parallelogram
View SolutionIn Figure, ABC is an isosceles triangle in which AB=AC.CPparel≤lAB and AP is the bisector of exterior ∠CAD of ABC . Prove that ∠PAC=∠BCA and (ii) ABCP is a parallelogram.
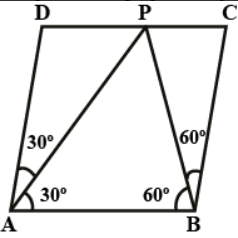
View SolutionIn Figure, ABCD is a parallelogram and ∠DAB=600. If the bisectors AP and BP of angles A and B respectively, meet at P on CD, prove that P is the mid-point of CD
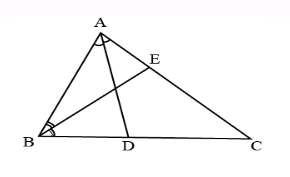
View SolutionIn Figure, ABC is a triangle in which ∠B=2∠C . D is a point on side BC such that AD bisects ∠BAC and AB=CD.BE is the bisector of ∠B . The measure of ∠BAC is
(a) 720
(b) 730
(c) 740
(d) 960
View SolutionAn exterior angle of a triangle is 1100 , and one of the interior opposite angles is 300. Find the other two angles of the triangle.
View SolutionABC and DBC are two isosceles triangles on the same bas BC and vertices A and D are on the same side of BC . If AD is extended to intersect BC at P, show that AP bisects \ ∠A as well as ∠D AP is the perpendicular bisector of BC
View SolutionP and Q are respectively the mid-points of sides AB and BC of a triangle ABC and Ris the mid-point of AP, show that (i) ar(PRQ)=12ar(ARC)(ii) ar(RQC)=38ar(ABC)(iii) ar(PBQ)=ar(ARC)
View Solution
