Question

${\displaystyle AD, BE and CF,}$
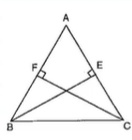
the altitudes of ${\displaystyle ABC}$
are equal. Prove that ${\displaystyle ABC}$
is an equilateral
triangle.

Explanation

To prove that triangle ABC is an equilateral triangle given that the altitudes AD, BE, and CF are equal, we will follow these steps:

Step 1: Establish the Given Information
We know that AD, BE, and CF are the altitudes from vertices A, B, and C to the opposite sides BC, AC, and AB respectively, and that these altitudes are equal:
\( AD = BE = CF \)

Step 2: Consider Triangles BEC and BFC
We will analyze triangles BEC and BFC.

- Angle E and Angle F: Both angles are right angles (90 degrees) since they are altitudes.
- Side EC and Side FC: We are given that \( EC = FC \) (as they are segments of the equal altitudes).
- Side BC: The side BC is common to both triangles.

Step 3: Apply the RHS Criterion
Using the Right angle-Hypotenuse-Side (RHS) criterion for congruence, we can conclude:
\( \triangle BEC \cong \triangle BFC \)

Step 4: Use CPCTC
From the congruence of triangles BEC and BFC, we can state:
\( \angle B = \angle C \) (by Corresponding Parts of Congruent Triangles are Congruent - CPCTC)

Step 5: Establish Relationship Between Sides
Since angles B and C are equal, the sides opposite these angles must also be equal:
\( AC = AB \) (sides opposite equal angles are equal)

Step 6: Consider Triangles ABD and ABE
Now, we will analyze triangles ABD and ABE.

- Angle D and Angle E: Both angles are right angles (90 degrees).
- Side AB: This side is common to both triangles.
- Side AD and Side BE: We are given that \( AD = BE \) (as they are equal altitudes).

Step 7: Apply the RHS Criterion Again
Using the RHS criterion again, we can conclude:
\( \triangle ABD \cong \triangle ABE \)

Step 8: Use CPCTC Again
From the congruence of triangles ABD and ABE, we can state:
\( \angle B = \angle A \) (by CPCTC)

Step 9: Establish Relationship Between Sides Again
Since angles A and B are equal, the sides opposite these angles must also be equal:
\( AB = BC \) (sides opposite equal angles are equal)

Step 10: Conclude the Proof
Now we have:
1. \( AC = AB \)
2. \( AB = BC \)

From these, we can conclude:
\( AC = AB = BC \)

Thus, triangle ABC is an equilateral triangle.