The impedance of a sereis RL circuit is same as the series RC circuit when connected to the same AC source separately keeping the same resistance. The frequency of the source is
1√LC
12π√LC
RL
1RC
The correct Answer is:B
The impedance in RL circuit is same as the RC circuit:
∴√R2+ω2L2=√R2+1ω2L2
⇒ω2=1LC or f=12π√LC
Topper's Solved these Questions
- ALTERNATING CURRENTBook:NARAYNAChapter:ALTERNATING CURRENTExercise:LEVEL - VI
Explore 120 Videos
- ALTERNATING CURRENTBook:NARAYNAChapter:ALTERNATING CURRENTExercise:LEVEL - I (H.W)
Explore 23 Videos
- ALTERNATING CURRENTBook:NARAYNAChapter:ALTERNATING CURRENTExercise:LEVEL - IV NCERT Based Questions
Explore 15 Videos
- ATOMIC PHYSICSBook:NARAYNAChapter:ATOMIC PHYSICSExercise:LEVEL-II (H.W)
Explore 14 Videos
Similar Questions
Knowledge Check
In a series L, R, C, circuit which is connected to a.c. source. When resonance is obtained then net impedance Z will be
AZ=RBZ=ωL−1ωCCZ=ωLDZ=1ωCThe power factor of an L-R series circuit is 0.5 and that of a C-R series circuit is 0.2. If the elements (L, C and R) of the two circuit are joined in series and connected to the same ac source, the power factor of this circuit is found to be 1. The ratio of the resistance in the L-R circuit to the resistance in the C-R circuit is
A2B√2C2√2D4In a series LCR circuit, impedance Z is same at two frequencies f1 and f2. Therefore, the resonant frequency of this circuit is
Af1+f22B2f1f2f1+f2C√f21+f222D√f1f2
Draw the phasor diagram for a series RC circuit connected to an a.c. source.
View SolutionResistor of resistance R and capacitor of capacitance C are connected in series to an AC source of angular frequency ω . Rms current in the circuit is I. When frequency of the source is changed to one - third of initial value keeping the voltage same then current is found to be halved. Find the ratio of reactance of capacitor to that with resistance at the original frequency ω .
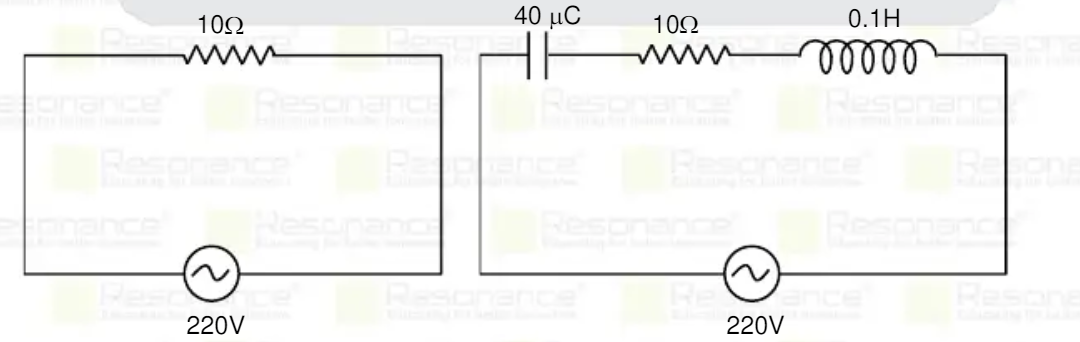
View SolutionPower in both the given circuit are same then find angular frequency of AC source
View SolutionAn a.c. voltage V=Vm sin ωt is applied across a
(i) series RC circuit in which the capacitive impedance is 'a' times the resistance in the circuit,
(ii) series RL circuit in which the inductive impedance is 'b' times the resistance in the circuit. Calculate the value of the power factor of the circuit in each case.
View SolutionIn an RL series circuit driven by an ac voltage source with fixed amplitude,
View Solution
