A 100km telegraph wire hasd capacity of 0.02μF/km, if it carries an alternating current of frequency 5kHZ. The value of an inductrance required to be connected in series so that the impedance is minimum.
50.7mH
5.07mH
0.507mH
507mH
The correct Answer is:3
To find the value of inductance L required to be connected in series with a telegraph wire to minimize the impedance when carrying an alternating current, we can follow these steps:
Step 1: Calculate the total capacitance C
The capacitance per kilometer is given as 0.02μF/km. For a length of 100km:
C=0.02μF/km×100km=2.0μF=2.0×10−6F
Step 2: Determine the angular frequency ω
The frequency f is given as 5kHz=5000Hz. The angular frequency ω is calculated as:
ω=2πf=2π×5000Hz≈31415.93rad/s
Step 3: Set up the resonance condition
At resonance, the inductive reactance XL equals the capacitive reactance XC:
XL=XC
Where:
XL=ωLandXC=1ωC
Thus, we have:
ωL=1ωC
Step 4: Solve for inductance L
Rearranging the equation gives:
L=1ω2C
Substituting the values of ω and C:
L=1(31415.93)2×(2.0×10−6)
Step 5: Calculate L
Calculating ω2:
ω2≈(31415.93)2≈9.8696×108
Now substituting back:
L=19.8696×108×2.0×10−6≈11.97392×103≈0.5066×10−3H
Thus, rounding gives:
L≈0.507mH=0.507×10−3H
Final Answer
The value of inductance required is approximately:
L≈0.507mH
---
The capacitance per kilometer is given as 0.02μF/km. For a length of 100km:
C=0.02μF/km×100km=2.0μF=2.0×10−6F
The frequency f is given as 5kHz=5000Hz. The angular frequency ω is calculated as:
ω=2πf=2π×5000Hz≈31415.93rad/s
At resonance, the inductive reactance XL equals the capacitive reactance XC:
XL=XC
Where:
XL=ωLandXC=1ωC
Thus, we have:
ωL=1ωC
Rearranging the equation gives:
L=1ω2C
Substituting the values of ω and C:
L=1(31415.93)2×(2.0×10−6)
Calculating ω2:
ω2≈(31415.93)2≈9.8696×108
Now substituting back:
L=19.8696×108×2.0×10−6≈11.97392×103≈0.5066×10−3H
Thus, rounding gives:
L≈0.507mH=0.507×10−3H
The value of inductance required is approximately:
L≈0.507mH
Topper's Solved these Questions
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - II (C.W)
11 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - LEVEL - III (C.W)
24 Videos
- ALTERNATING CURRENTBOOK - NARAYNACHAPTER - ALTERNATING CURRENTEXERCISE - Assertion & Reason
14 Videos
- ATOMIC PHYSICSBOOK - NARAYNACHAPTER - ATOMIC PHYSICSEXERCISE - LEVEL-II (H.W)
14 Videos
Similar Questions
A 200 km telephone wire has capacity of 0.014μFkm−1. If it carries an alternating current oif frequency 50kHz, what should be the value of an inductance required to be connected in series so that impedance is minimumn ?
View SolutionA 200km long telegraph wire has capacitance of 0.014μF/km If it carries an alternating current of 50×103 Hz what should be the value of an inductance required to be connected in series in series so that impedance isw minimum .
View Solution
Knowledge Check
A 200 km long telegraph wire has a capacitance of 0.025μF/km. It carries an alternating current of 50 KHz. What should be the value of an inductance required to be connected in series, so that the impedance is minimum. (take π2=10)
A1μHB2μHC5μHD8μHA telephone wire of length 200km has a capacitance of 0.014μFkm. If it carries an AC of frequency 5kHz what should be the value of an inductor required to be connected in series so that impedence of the circuit is minimum ?
A0.35mHB35mHC3.5mHDZeroThe diode used at a constant potential drop of 0.5 V at all currents and maximum power rating of 100 mW. What resistance must be connected in series diode, so that current in circuit is maximum?
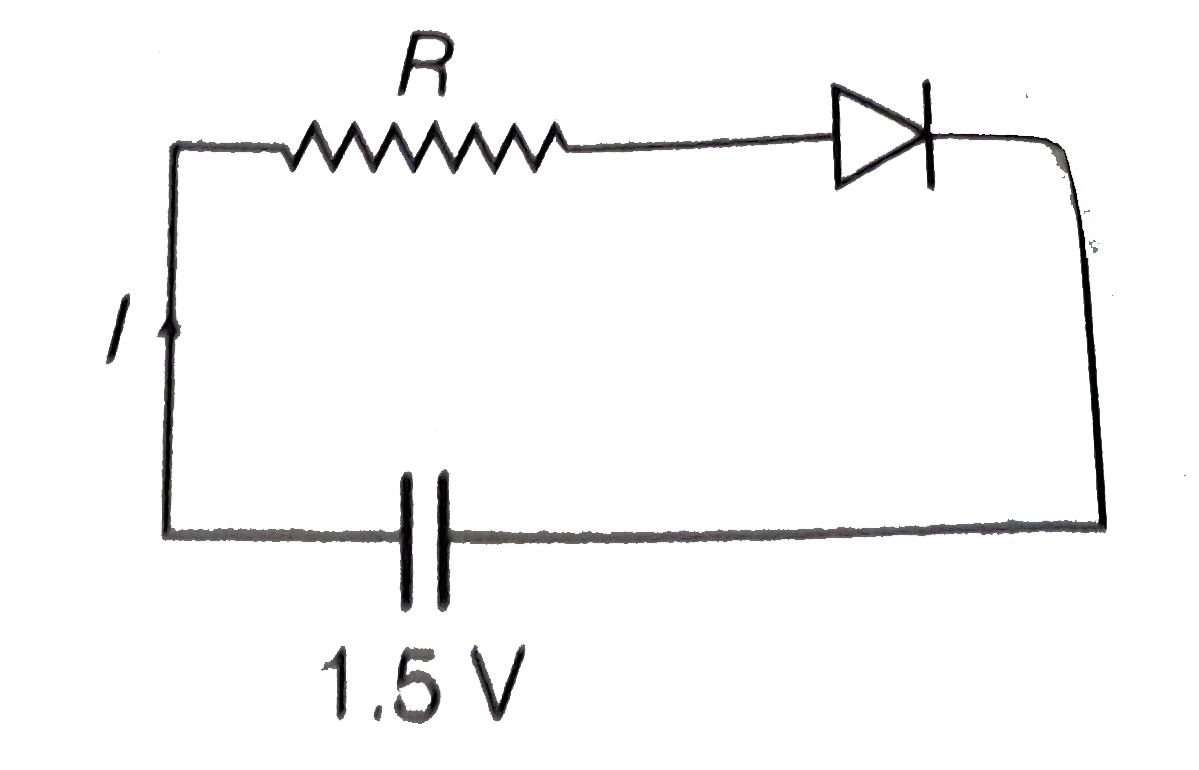 A200ΩB6.67ΩC5ΩD15Ω
A200ΩB6.67ΩC5ΩD15Ω
A telephone wire of length 200km has a capacitance of 0.0125 muFper km.If it carries an ac of frequency 5KHz.What should be the value of an inductor required to be connected in series.So that the impedance of the circuit is minimum (pi^(2)=10)
View SolutionA coil of inductance L=5/8H and of resistance R=62.8(Ω) is connected to the mains alternating voltage of frequency 50 Hz. What can be the capacitance of the capacitor (∈(μ)F) connected in series with the coil if the power dissipated has to remain unchanged? Take (π)2=10.
View SolutionA capacitor of capacitance 0.5 μF is connected to a source of alternating e.m.f. of frequency 100 Hz. What is the capacitive reactance? (π=3.142)
View SolutionA 10 m long nichrome wire having 80Ω resistance has current carrying capacity of 5 A. This wire can be cut into equal parts and equal parts can be connected in series or parallel. What is the maximum power which can be obtain as heat by the wire from a 200 V mains supply? (in kW)
View SolutionIf resistance of 100Ω , inductance of 0.5 henry and capacitor of 10×10−6F are connected in series through 50Hz AC supply, then impedence is
View Solution
