Two coplanar circular coils of equal radius carrying currents i1,i2 in opposite directions are at a large distance 'd'. The distance from the first coil where the resultant magnetic induction is zero is
d1+i2i1
d1+√i2i1
d1+(i2i1)13
d1+(i2i1)2
The correct Answer is:C
Step by step video, text & image solution for Two coplanar circular coils of equal radius carrying currents i_(1),i_(2) in opposite directions are at a large distance 'd'. The distance from the first coil where the resultant magnetic induction is zero is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISMBOOK - NARAYNACHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - LEVEL-I(C.W)
58 Videos
- MOVING CHARGES AND MAGNETISMBOOK - NARAYNACHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Level-II(C.W)
63 Videos
- MOVING CHARGES AND MAGNETISMBOOK - NARAYNACHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - EXERCISE - 4
20 Videos
- MAGNETISM AND MATTERBOOK - NARAYNACHAPTER - MAGNETISM AND MATTEREXERCISE - EXERCISE - 4 (SINGLE ANSWER TYPE QUESTION)
17 Videos
- NUCLEAR PHYSICSBOOK - NARAYNACHAPTER - NUCLEAR PHYSICSEXERCISE - LEVEL-II-(H.W)
9 Videos
Similar Questions
Two parallel wires P and Q placed distance r apart. They are carrying currents I1 and I2 where I1>I2, but in opposite directions as shown in figure. Find the point on the line PQ where the resultant magnetic field is zero.
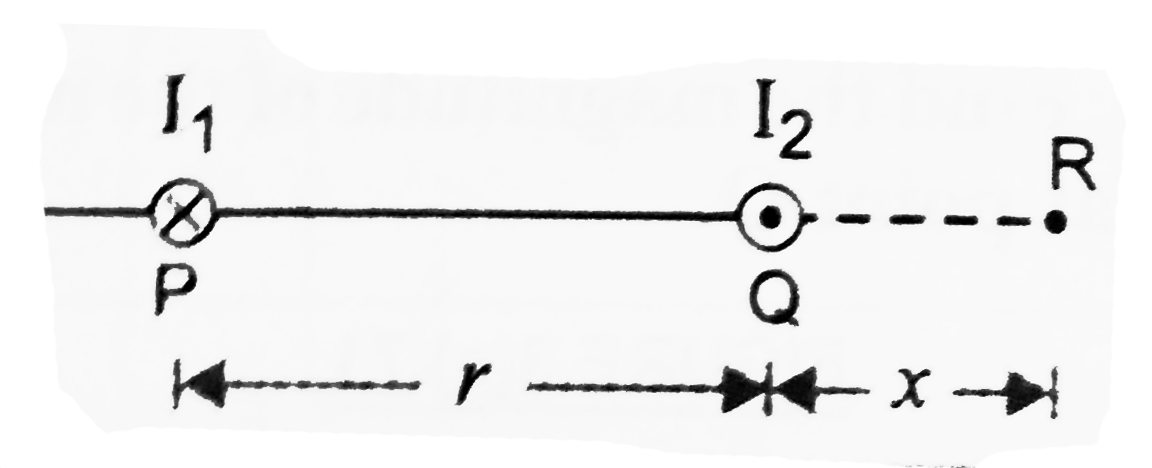
View SolutionTwo parallel coaxial circular coils of equal radius R and equal number of turns N carry equal currents I in the same direction and are separated by a distance 2R. Find the magnitude and direction of the net magnetic field produced at the mid-point of the line joining their centres.
View Solution
Knowledge Check
Two parallel wires carrying currents I1 and I2 in opposite directions and separated by a distance d experience a
Arepulsive force μ0I1I2/2πdBattractive force μ0I1I2/2πdCrepulsive force μ0I1I2/2πd2Dattractive force μ0I1I2/2πd2Two parallel wires carrying currents I1 and I2 in opposite directions and separated by a distance d experience a
Arepulsive force μ0I1I2/2πdBattractive force μ0I1I2/2πdCrepulsive force μ0I1I2/2πd2Dattractive force μ0I1I2/2πd2A circular coil of radius R carries a current i. The magnetic field at its centre is B. The distance from the centre on the axis of the coil where the magnetic field will be B/8 is
A√2RB√3RC2RD3R
For a circular coil of radius R and N carrying current I, the magnitude of the magnetic field at a point on its axis at a distance x from its centre is given by
B=μ0IR2N2(x2+R2)3/2
(a) Show that this reduces to the familiar result for field at the centre of the coil.
(b) Consider two parallel co-axial circular coil of equal radius R, and number of turns N, carrying equal currents in the same direction, and separated by a distance R. Show that the field on the axis around the mid-point between the coils is uniform over a distance that is small as compared to R, and is given by ,
B=0.72μ0NIR , approximately.
[Such an arrangment to produce a nearly uniform magnetic field over a small region is known as Helmholtz coils.]
View SolutionA circular coil of n turns and radius r carries a current I. The magnetic field at the center is
View SolutionTwo circular coils P & Q are coaxially & carry currents I1 and I2 respectively (all direction are w.r.t. the observer)

View SolutionA circular coil of radius r carries a current I. The magnetic field at its centre is B. At what distance from the centre, on the axis of the coil the magneitc field will be B/27 ?
View SolutionTwo identical coils each of radius R and each carrying a current I in the same direction are placed along a common axis and separated by distance R. At the midpoint between the two coils the
View Solution
