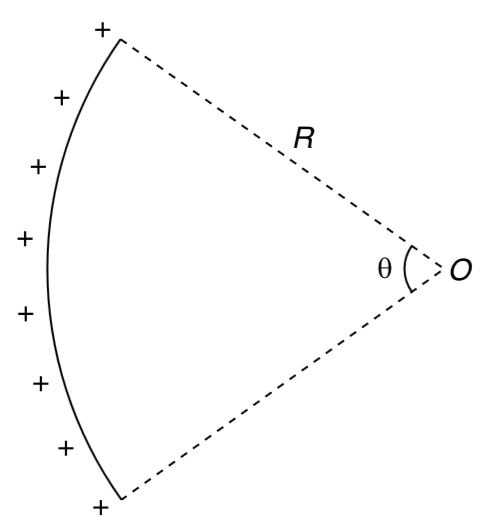
Consider a circular arc of radius $R$ which subtends an angle $ϕ$ at its centre .Let us calculate the electric field strength at $C$

Video Solution

Text Solution

Verified by Experts

Consider a polar segment on arc of angular width $d θ$ at an angle $θ$ form the angular bisector $X Y$ as shown ,the length of element segment is $R d θ$ .The charge on this element $d q$ is
$d q = \frac{Q}{ϕ} d θ$
Due to this $d q$ ,electric field at a centre of arc $C$ is given as
$d E = \frac{d q}{4 π ε_{0} R^{2}}$
The electric field components $d E$ to this segment $d E sin θ$ which is perpendicular to the angle bisector gets cancelled out integration.
the net electric field at centre wil be along angle bisector which can be calculated by intersecting $d E cos θ$ which limits from $- ϕ / 2$ to $ϕ / 2$ .
Hence net elctric field strength at centre C is
$E_{c} = \int d E cos θ$
$= \int_{ϕ / 2}^{- ϕ / 2} \frac{Q}{4 π ε_{0} ϕ R^{2}} cos θ d θ$
$\frac{Q}{4 π ε_{0} ϕ R^{2}} cos θ d θ E_{c} = \int d E cos θ$
$= \int_{ϕ / 2}^{- ϕ / 2} \frac{Q}{4 π ε_{0} R^{2} ϕ} cos θ d θ$
$= \frac{Q}{4 π ε_{0} R^{2} ϕ} = \int_{ϕ / 2}^{- ϕ / 2} cos θ d θ$
$\frac{Q}{4 π ε_{0} ϕ R^{2}} {[sin θ]}_{ϕ / 2}^{ϕ / 2}$
$= \frac{Q}{4 π ε_{0} R^{2} ϕ} [sin ϕ / 2 + sin ϕ / 2]$
$E_{c} = \frac{2 Q sin (ϕ / 2)}{4 π ε_{0} R^{2} ϕ}$
for a semi circualr ring $ϕ = π$ So at centre
$E_{c} = \frac{2 Q sin (ϕ / 2)}{4 π ε_{0} R^{2} ϕ} = \frac{2 Q sin (ϕ / 2)}{4 ϕ ε_{0} R^{2} π} = \frac{2 Q}{4 π ε_{0} R^{2}}$

Updated on:21/07/2023