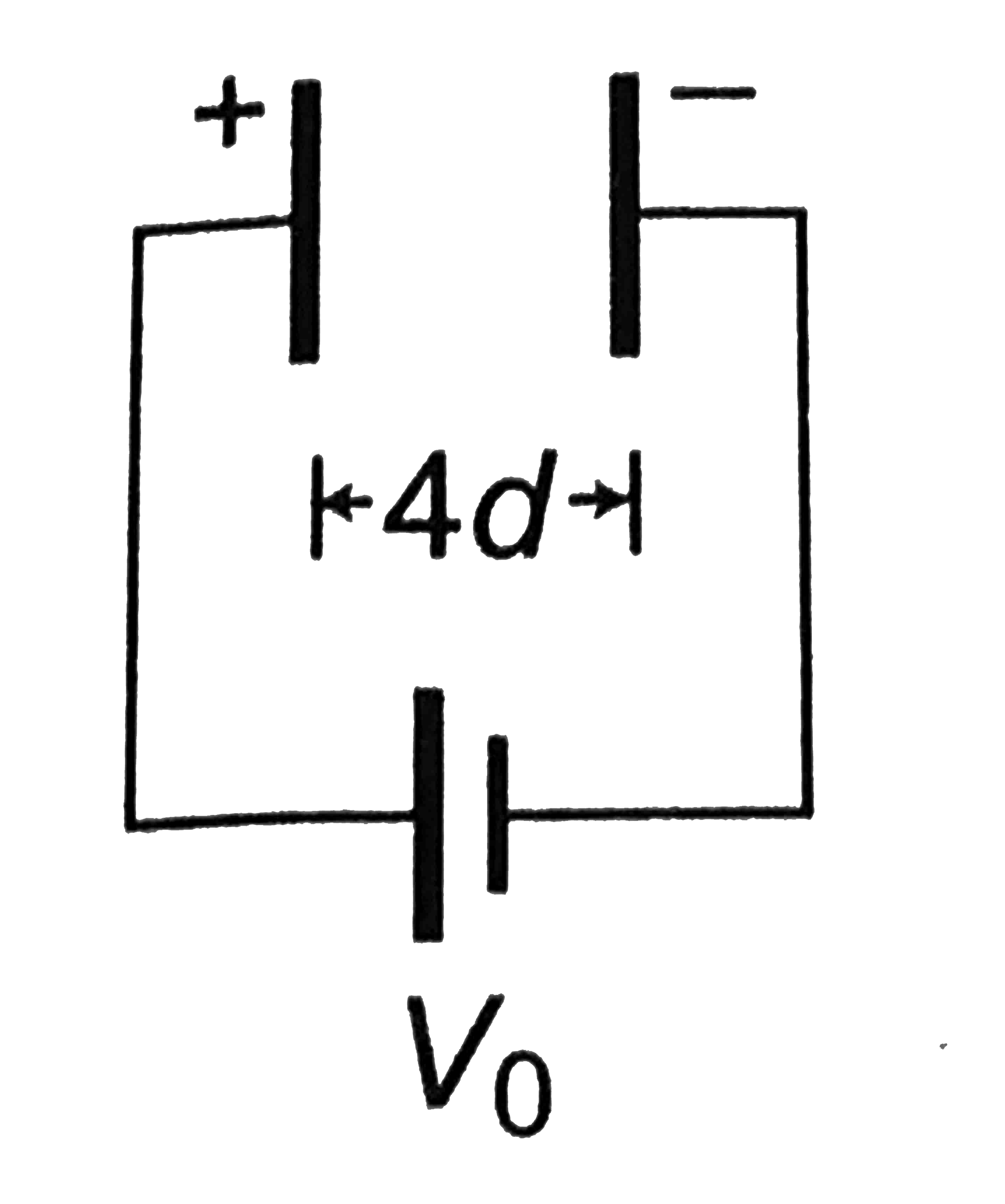
A parallel plate capacitor having plate area A and separation 4d is connected to a battery of emf V0. A diellectric slab of thickness 2d and dielectric constant 2.0 is inserted into the gap. (a) find the increase in energy strored in capacitor. (b) Now battery is removed and dielectric slab taken out, find further increase in energy.
(a) C1=A∈04d,V1=V0
U1=12C1V21=12A∈04dV20=A∈0V208d
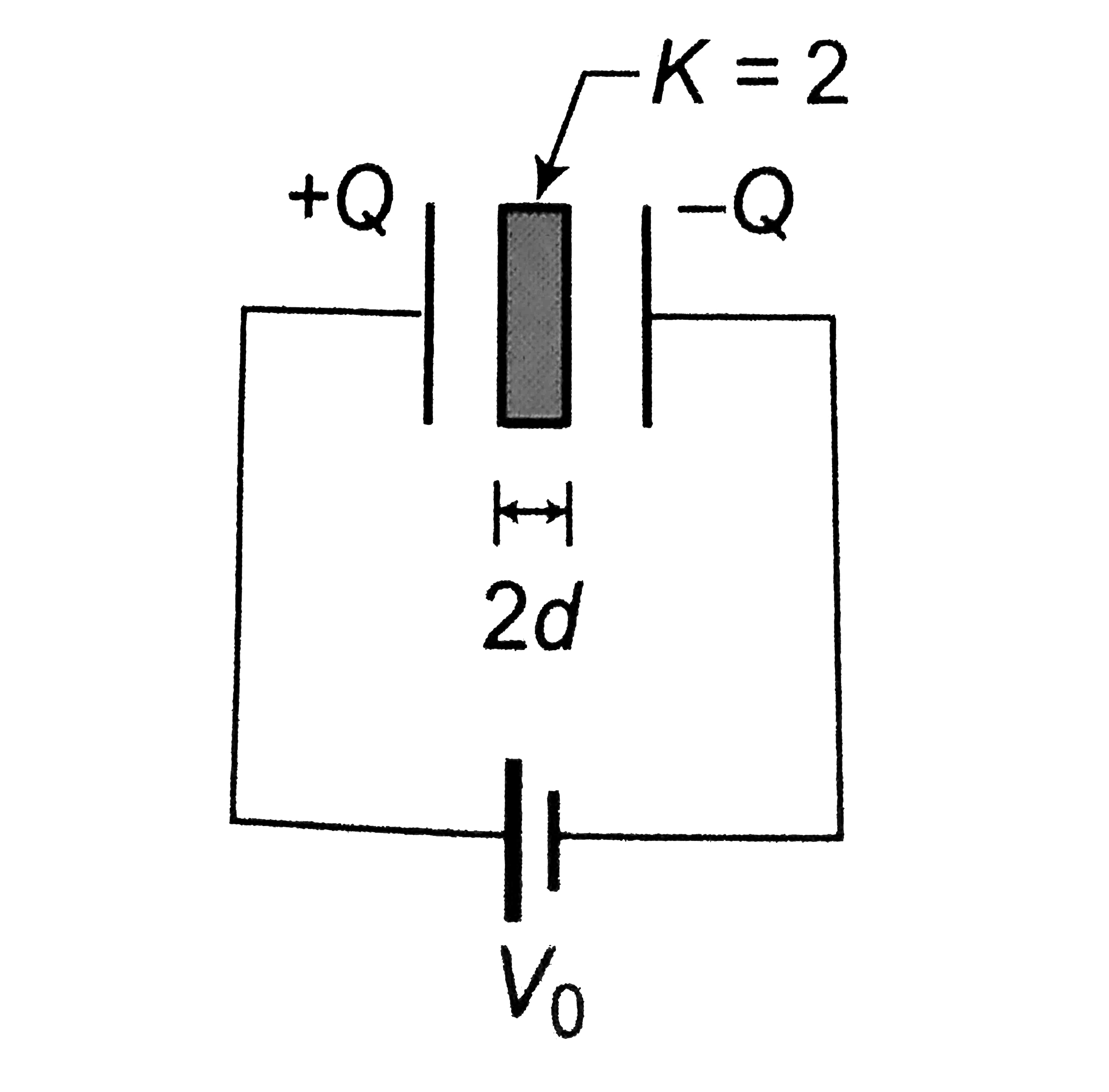
C2=A∈04d−t+tK=A∈04d−2d+2d2=A∈03d,V2=V0
U2=12C2V22=12A∈03d.V20=A∈0V206d
ΔU=U2−U1=(16−18)A∈0V20d=A∈0V2024d
Q=C2V2=A∈0V03d
(c) C3=C1=A∈04d
U3=Q22C3=(A∈0V03d)212.A∈04d=A∈0V209d
ΔU'=U3−U2=(29−16)A∈0V20d
=A∈0V2018d
Topper's Solved these Questions
Similar Questions
A parallel -plate capacitor having plate area 400cm2 and separation between the plate 1.0mm is connected to a power supply of 100V. A dielectric slab of thickness1.0mm and dielectric constant 5.0 is inserted into the gap .(a)Find the increase in electrostatic energy .(b) If the power supply is now disconnected and the dielectric slab is taken out , find the further increase in energy. (c) Why does the energy increase in inserting the slab as well as in taking it out?
View SolutionA parallel plate capacitor (plate length L, plate width b, separation between plates d) is connected to a battery of emf V. A dielectric slab of dielectric constant K and before capacitor. Determine the force by which dielectric is attrached by capacitor.
View Solution
Knowledge Check
After charging a capacitor the battery is removed. Now by placing a dielectric slab between the plates
Athe potential difference between the plates and the energy stored will decreases but the charge on plates will remains sameBthe charge on the plates will decreases and the potential difference between the plates will increasesCthe potential difference between the plates will increases and energy stored will decreases but the charge on the plates will remains sameDthe potential difference, energy stored and the charge will remain unchangedA capacitor with plate separation d is charged to V volts. The battery is disconnected and a dielectric slab of thickness d2 and dielectric constant '2' is inserted between the plates. The potential difference across its terminals becomes
AVB2 VC4V3D3V4Two identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
A50V,50VB80V,20VC20V,80VD75V,25V
A parallel plate capacitor has plate of length 'l' width 'w' and separation of plates is 'd' . It is connected to a battery of emf V . A dielectric slab of the same thickness 'd' and of dielectric constant k=4 is being inserted between the plates of the capacitor . At what lenght of the slab inside plates , will the energy stored in the capacitor be two time the initial energy stored ?
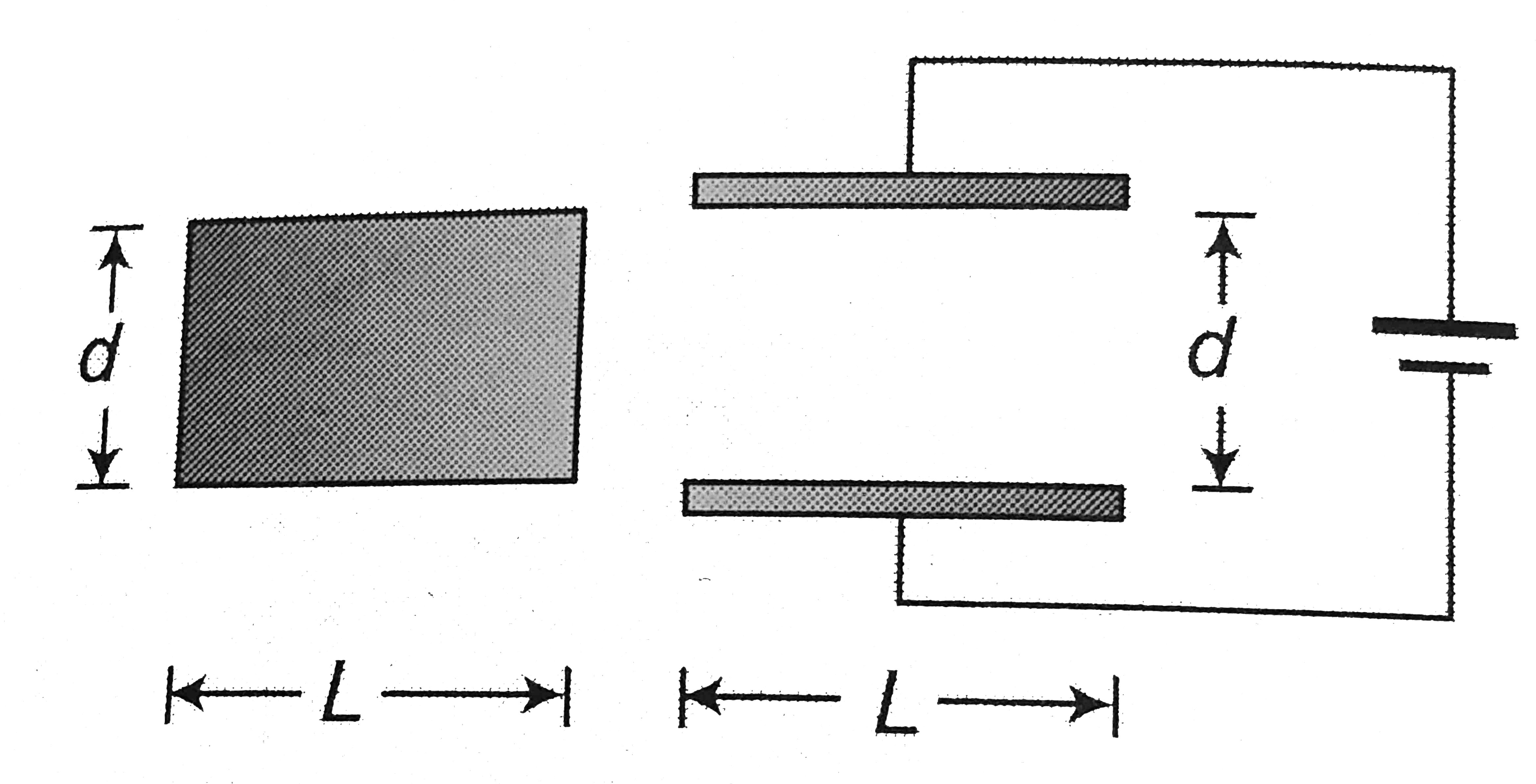
View SolutionShown a parallel plate capacitor with plates of width b and length l. The separation between the platesis d. The plates are rigidly clamped and connected to a battery of emf V. A dielectric slab of thickness d and dielectric constant K is slowly inserted between the plates. (a) Calculate the energy of the system when a length x of the slab is introduced into the capacitor. (b) what force should be applied on teh slab to ensure that is goes slowly into the capacitor? Neglect any effect of friction or gravity.

View SolutionA parallel-plate capacitor having plate are 100cm2 and separation 1.0 mm holds a charge of 0.12μC when connected to a 120 V battery. Find the dielectric constant of the material filling the gap.
View SolutionA parallel plate capacitor , each with plate area A and separation d , is charged to a potential difference V . The battery used to charge it remains connected . A dielectric slab of thickness of and dielectric constant K is now placed between the plates . What change , if any , will take place in :
charge on plates ?
Justify your answer
View SolutionA parallel plate capacitor has plates with area A and separation d. A battery charges the plates to a potential difference V0. The battery is then disconnected and a dielectric slab of thikness d is introduced. The ratio of the enrgy stored in the capacitor before and after the slab is introduced, is
View Solution