A parallel plate capacitor (plate length L, plate width b, separation between plates d) is connected to a battery of emf V. A dielectric slab of dielectric constant K and before capacitor. Determine the force by which dielectric is attrached by capacitor.
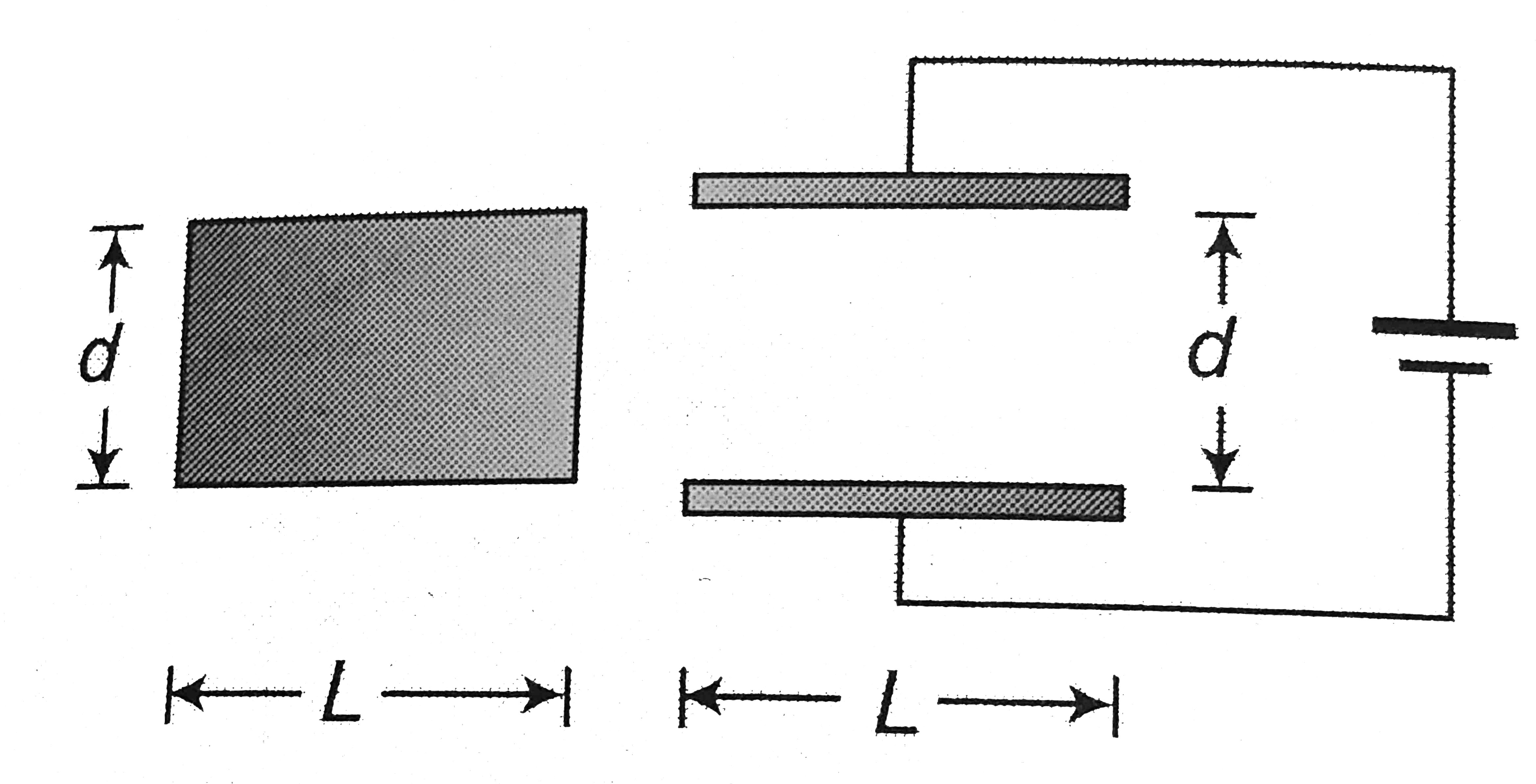
Here capacitor is connected to battery and hence it is attracted by constant force, as shown in the following solution.
Let at some instant, x length of dielectric is inside capacitor.
C1=Kxb∈0d,C2=(L−x)b∈0d
C1 and C2 are in parallel
Ceq=C1+C2=b∈0d[kx+(L−x)]
=b∈0d[(K−1)x+L]
Energy stored in capacitor
U=12CeqV2=12b∈0d[(K−1)x+L]V2
F=−dUdx=−(K−1)b∈0V22d
−ve sign shows that force F is attractive.
OR
Since force is constant, it can be determined as follows. when capacitor is empty i.e., no dielectric slab in it,
Energy stored U1=12CV2,C=Lb∈0d
when dielectric slab is between plates,
U2=12KCV2
work done W=U2−U1=12(K−1)CV2 ...(i)
Since force is constant,
Work done by force =FL ...(ii)
FL=12(K−1)CV2=12(K−1)Lb∈0dV2
F=(K−1)b∈0V22d
Topper's Solved these Questions
Similar Questions
A parallel plate capacitor has plate of length 'l' width 'w' and separation of plates is 'd' . It is connected to a battery of emf V . A dielectric slab of the same thickness 'd' and of dielectric constant k=4 is being inserted between the plates of the capacitor . At what lenght of the slab inside plates , will the energy stored in the capacitor be two time the initial energy stored ?
View SolutionShown a parallel plate capacitor with plates of width b and length l. The separation between the platesis d. The plates are rigidly clamped and connected to a battery of emf V. A dielectric slab of thickness d and dielectric constant K is slowly inserted between the plates. (a) Calculate the energy of the system when a length x of the slab is introduced into the capacitor. (b) what force should be applied on teh slab to ensure that is goes slowly into the capacitor? Neglect any effect of friction or gravity.

View Solution
Knowledge Check
A parallel plate capacitor of capacitance 90 pF is connected to a battery of emf 20 V. If a dielectric material of dielectric constant K=53 is inserted between the plates, the magnitude of the induced charge will be :
A0.9nCB1.2nCC0.3nCD2.4nCConsider the diagram,

A parallel plate capacitor has the plate width t and length L while the separation between the plates is d. The capacitor is connected to a battery of voltage rating V. A dielectric which carefully occupy, the space between the plates of the capacitor is slowly inserted between the plates. when length x of the dielectric slab is introduced into the capacitor, then energy stored in the system isAε0tV22dLBε0tV22dL[x+1]Cε0tV22d[L+x(K−1)]Dε20t2V22d2[L2+x+1]Two identical parallel plate capacitors are connected in series to a battery of 100V. A dielectric slab of dielectric constant 4.0 is inserted between the plates of second capacitor. The potential difference across the capacitors will now be respectively
A50V,50VB80V,20VC20V,80VD75V,25V
A parallel plate capacitor of capacitance 200μF is connected to a battery of 200 V. A dielectric slab of dielectric 2 is now inserted into the space between plates of capacitor while the battery remain connected .The change in the electrostatic energy in the capacitor will be __________J.
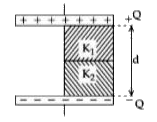
View SolutionA parallel-plate capacitor with plate area A has separation d between the plates. Two dielectric slabs of dielectric constant K1 and K2 of same area A/2 and thickness d/2 are inserted in the space between the plates. The capacitance of the capacitor will be given by :
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dielectric constant K is placed in between the plates of the capacitor as shown.
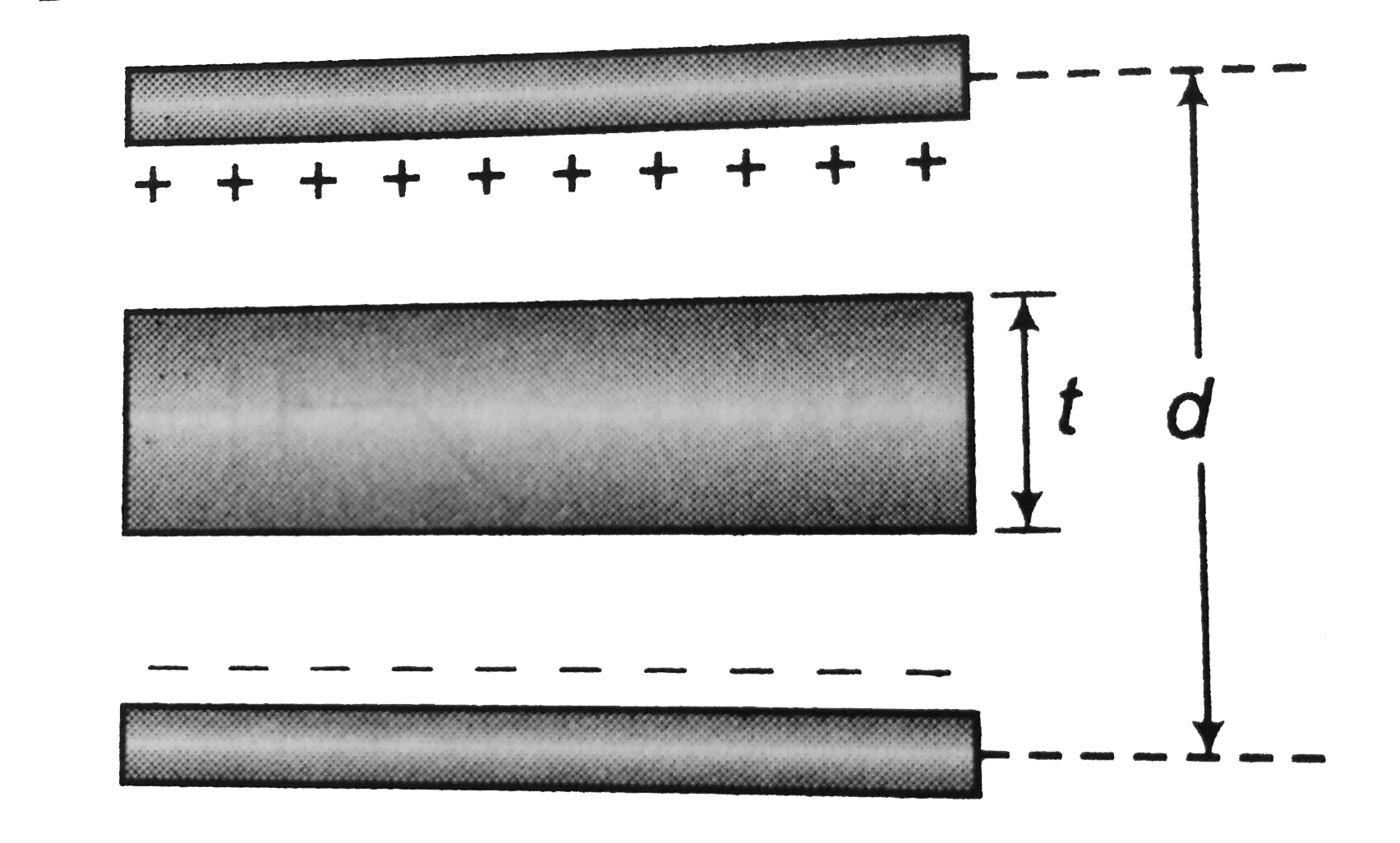
The electric field in the dielectric slab is
View SolutionFigure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
The electric field in the gaps between the plates and the electric slab will be
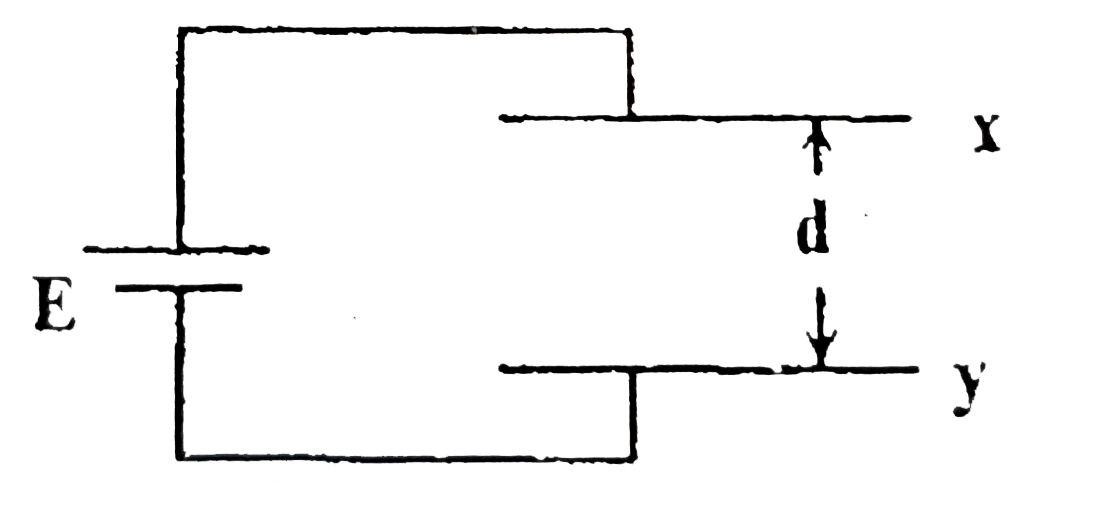
View SolutionA parallel-plate capacitor has plate area A, the distance between its two plates x and y is d and a dielectric slab of dielectric constant εr is filled between the regions. It is connected to a cell of emf E. If the dielectric slab is removed, then
View Solution
